Hostname: page-component-76fb5796d-r6qrq Total loading time: 0 Render date: 2024-04-26T13:04:33.768Z Has data issue: false hasContentIssue false
  • English
  • Français

BRAIDED MIXED DATUMS AND THEIR APPLICATIONS ON HOM-QUANTUM GROUPS

Part of: Hopf algebras, quantum groups and related topics

Published online by Cambridge University Press:  04 September 2017

XIAOHUI ZHANG  and
LIHONG DONG
XIAOHUI ZHANG
Affiliation:
School of Mathematical Sciences, Qufu Normal University, Qufu 273165, P. R. China e-mail: zxhhhhh@hotmail.com
LIHONG DONG
Affiliation:
College of Mathematics and Information Science, Henan Normal University, Xinxiang Henan 453007, P. R. China e-mail: lihongdong2010@gmail.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

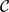In this paper, we mainly provide a categorical view on the braided structures appearing in the Hom-quantum groups. Let $\mathcal{C}$ be a monoidal category on which F is a bimonad, G is a bicomonad, and ϕ is a distributive law, we discuss the necessary and sufficient conditions for $\mathcal{C}^G_F(\varphi)$, the category of mixed bimodules to be monoidal and braided. As applications, we discuss the Hom-type (co)quasitriangular structures, the Hom–Yetter–Drinfeld modules, and the Hom–Long dimodules.

MSC classification

Primary: 16T25: Yang-Baxter equations
Type
Research Article
Information
Glasgow Mathematical Journal , Volume 60 , Issue 1 , January 2018 , pp. 231 - 251
Copyright
Copyright © Glasgow Mathematical Journal Trust 2017 

References

REFERENCES

1. Alonso, J. N. Álvarez, J. M. Vilaboa, Fernández and Rodríguez, R. González, Cleft extensions and Galois extensions for Hom-associative algebras, Int. J. Math. 27 (3) (2016), 1650025.Google Scholar
2. Beck, J., Distributive laws, Lect. Notes Math. 80 (1969), 119–140.Google Scholar
3. Böhm, G., Lack, S. and Street, R., Weak bimonads and weak Hopf monads, J. Algebra 328 (1) (2011), 1–30.Google Scholar
4. Bruguières, A. and Virelizier, A., Hopf monads, Adv. Math. 215 (2) (2007), 679–733.Google Scholar
5. Bruguières, A., Lack, S. and Virelizier, A., Hopf monads on monoidal categories, Adv. Math. 227 (2) (2011), 745–800.Google Scholar
6. Caenepeel, S. and Goyvaerts, I., Monoidal Hom–Hopf algebras, Comm. Algebra 39 (6) (2011), 2216–2240.Google Scholar
7. Chen, Y. Y., Wang, Z. W. and Zhang, L. Y., Integrals for monoidal Hom–Hopf algebras and their applications, J. Math. Phys. 54 (7) (2013), 073515.Google Scholar
8. Hartwig, J. T., Larsson, D. and Silvestrov, S. D., Deformation of Lie algebras using σ-derivations, J. Algebra 295 (2) (2006), 314–361.Google Scholar
9. Hobst, D. and Pareigis, B., Double quantum groups, J. Algebra 242 (2) (2001), 460–494.Google Scholar
10. Hu, N. H., q-Witt algebras, q-Lie algebras, q-holomorph structure and representations, Algebra Colloq. 6 (1999), 51–70.Google Scholar
11. Makhlouf, A. and Panaite, F., Yetter–Drinfeld modules for Hom-bialgebras, J. Math. Phys. 55 (1) (2014), 013501.Google Scholar
12. Makhlouf, A. and Panaite, F., Hom-L-R-smash products, Hom-diagonal crossed products and the Drinfeld double of a Hom-Hopf algebra, J. Algebra 441 (1) (2015), 313–343.Google Scholar
13. Makhlouf, A. and Silvestrov, S. D., Hom-algebras and Hom-coalgebras, J. Algebra Appl. 9 (4) (2010), 553–589.Google Scholar
14. Makhlouf, A. and Silvestrov, S. D., Hom-algebras structures, J. Gen. Lie Theory Appl. 2 (2008), 51–64.Google Scholar
15. Makhlouf, A. and Silvestrov, S. D., Hom–Lie admissible Hom-coalgebras and Hom–Hopf algebras, in Generalized lie theory in mathematics, physics and beyond (Silvestrov, S., Paal, E., Abramov, V. and Stolin, A., Editors) (Springer-Verlag, Berlin, 2008), Chp 17, 189–206.Google Scholar
16. Makhlouf, A. and Silvestrov, S. D., Notes on formal deformations of Hom-associative and Hom-Lie algebras, Forum Math. 22 (2010), 715–759.CrossRefGoogle Scholar
17. Moerdijk, I., Monads on tensor categories, J. Pure Appl. Algebra 168 (2) (2002), 189–208.Google Scholar
18. Montgomery, S., Hopf algebras and their actions on rings, in CMBS Reg. Conf. Ser. in Math., vol. 82, Am. Math. Soc., Providence, 1993.Google Scholar
19. Power, J. and Watanabe, H., Combining a monad and a comonad, Theor. Comput. Sci. 280 (1–2) (2002), 137–162.Google Scholar
20. Street, R., The formal theory of monads, J. Pure Appl. Algebra 2 (2) (1972), 149–168.Google Scholar
21. Yau, D., Hom-quantum groups I: Quasitriangular Hom-bialgebras, J. Phys. A 45 (6) (2012), 065203.Google Scholar
22. Yau, D., Hom–Yang–Baxter equation, Hom–Lie algebras and quasitriangular bialgebras, J. Phys. A 42 (16) (2009), 165202.Google Scholar
23. Yau, D., The Hom–Yang–Baxter equation and Hom–Lie algebras, J. Math. Phys. 52 (5) (2011), 053502.Google Scholar
24. Zhang, X. H. and Wang, S. H., Weak Hom–Hopf algebras and their (co)representations, J. Geom. Phys. 94 (2015), 50–71.Google Scholar