No CrossRef data available.
Article contents
Invariant measures for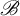 
$\mathscr {B}$-free systems revisited
Part of:
Set functions and measures on spaces with additional structure
Ergodic theory
Topological dynamics
Published online by Cambridge University Press: 08 March 2024
Abstract
For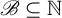 
$\mathscr {B} \subseteq \mathbb {N} $, the 
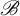$ \mathscr {B} $-free subshift 
$ X_{\eta } $ is the orbit closure of the characteristic function of the set of 
$ \mathscr {B} $-free integers. We show that many results about invariant measures and entropy, previously only known for the hereditary closure of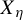 
$ X_{\eta } $, have their analogues for 
$ X_{\eta } $ as well. In particular, we settle in the affirmative a conjecture of Keller about a description of such measures [G. Keller. Generalized heredity in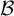 
$\mathcal B$-free systems. Stoch. Dyn. 21(3) (2021), Paper No. 2140008]. A central assumption in our work is that 
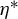$\eta ^{*} $ (the Toeplitz sequence that generates the unique minimal component of 
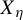$ X_{\eta } $) is regular. From this, we obtain natural periodic approximations that we frequently use in our proofs to bound the elements in 
$ X_{\eta } $ from above and below.
Keywords
- Type
- Original Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
Baake, M. and Grimm, U.. Aperiodic Order. Volume 1: A Mathematical Invitation (Encyclopedia of Mathematics and its Applications, 149). Cambridge University Press, Cambridge, 2013; with a foreword by R. Penrose.CrossRefGoogle Scholar
Bergelson, V., Downarowicz, T. and Vandehey, J.. Deterministic functions on amenable semigroups and a generalization of the Kamae–Weiss theorem on normality preservation. J. Anal. Math. 148(1) (2022), 213–286.CrossRefGoogle Scholar
Davenport, H. and Erdös, P.. On sequences of positive integers. J. Indian Math. Soc. (N.S.) 15 (1951), 19–24.Google Scholar
Downarowicz, T.. Survey of odometers and Toeplitz flows. Algebraic and Topological Dynamics (Contemporary Mathematics, 385). Ed. Kolyada, S., Manin, Y. and Ward, T.. American Mathematical Society, Providence, RI, 2005, pp. 7–37.CrossRefGoogle Scholar
Downarowicz, T.. Entropy in Dynamical Systems (New Mathematical Monographs, 18). Cambridge University Press, Cambridge, 2011.Google Scholar
Dymek, A., Kasjan, S. and Kułaga-Przymus, J.. Minimality of
$\mathfrak{B}$
-free systems in number fields. Discrete Contin. Dyn. Syst. 43(9) (2023), 3512–3548.CrossRefGoogle Scholar
$\mathfrak{B}$
-free systems in number fields. Discrete Contin. Dyn. Syst. 43(9) (2023), 3512–3548.CrossRefGoogle ScholarDymek, A., Kasjan, S., Kułaga-Przymus, J. and Lemańczyk, M.. 𝔅-free sets and dynamics. Trans. Amer. Math. Soc. 370(8) (2018), 5425–5489.Google Scholar
Eizenberg, A., Kifer, Y. and Weiss, B. Large deviations for Zd-actions. Comm. Math. Phys. 164(3) (1994), 433–454.Google Scholar
El Abdalaoui, E. H., Lemańczyk, M. and de la Rue, T.. A dynamical point of view on the set of 𝔅-free integers. Int. Math. Res. Not. IMRN 16 (2015), 7258–7286.CrossRefGoogle Scholar
Furstenberg, H.. Disjointness in ergodic theory, minimal sets, and a problem in Diophantine approximation. Math. Systems Theory 1 (1967), 1–49.Google Scholar
Glasner, E.. Ergodic Theory via Joinings (Mathematical Surveys and Monographs, 101). American Mathematical Society, Providence, RI, 2003.CrossRefGoogle Scholar
Hall, R. R.. Sets of Multiples (Cambridge Tracts in Mathematics, 118). Cambridge University Press, Cambridge, 1996.CrossRefGoogle Scholar
Jacobs, K. and Keane, M..
$0-1$
-sequences of Toeplitz type. Z. Wahrscheinlichkeitstheorie und Verw. Gebiete 13 (1969), 123–131.Google Scholar
$0-1$
-sequences of Toeplitz type. Z. Wahrscheinlichkeitstheorie und Verw. Gebiete 13 (1969), 123–131.Google ScholarKasjan, S., Keller, G. and Lemańczyk, M.. Dynamics of 𝔅-free sets: a view through the window. Int. Math. Res. Not. IMRN 9 (2019), 2690–2734.Google Scholar
Kechris, A. S.. Classical Descriptive Set Theory (Graduate Texts in Mathematics, 156). Springer-Verlag, New York, 1995.CrossRefGoogle Scholar
Keller, G.. Tautness for sets of multiples and applications to 𝔅-free dynamics. Studia Math. 247(2) (2019), 205–216.Google Scholar
Keller, G.. Generalized heredity in 𝔅-free systems. Stoch. Dyn. 21(3) (2021), Paper no. 2140008.CrossRefGoogle Scholar
Keller, G.. Irregular 𝔅-free Toeplitz sequences via Besicovitch’s construction of sets of multiples without density. Monatsh. Math. 199(4) (2022), 801–816.Google Scholar
Keller, G. and Richard, C.. Dynamics on the graph of the torus parametrization. Ergod. Th. & Dynam. Sys. 38(3) (2018), 1048–1085.CrossRefGoogle Scholar
Konieczny, J., Kupsa, M. and Kwietniak, D.. Arcwise connectedness of the set of ergodic measures of hereditary shifts. Proc. Amer. Math. Soc. 146(8) (2018), 3425–3438.CrossRefGoogle Scholar
Konieczny, J., Kupsa, M. and Kwietniak, D.. On
$\overline{d}$
-approachability, entropy density and 𝔅-free shifts. Ergod. Th. & Dynam. Sys. 43(3) (2023), 943–970.Google Scholar
$\overline{d}$
-approachability, entropy density and 𝔅-free shifts. Ergod. Th. & Dynam. Sys. 43(3) (2023), 943–970.Google ScholarKułaga-Przymus, J., Lemańczyk, M. and Weiss, B.. On invariant measures for 𝔅-free systems. Proc. Lond. Math. Soc. (3) 110(6) (2015), 1435–1474.Google Scholar
Kułaga-Przymus, J., Lemańczyk, M. and Weiss, B.. Hereditary subshifts whose simplex of invariant measures is Poulsen. Ergodic Theory, Dynamical Systems, and the Continuing Influence of John C. Oxtoby (Contemporary Mathematics, 678). Ed. Auslander, J., Johnson, A. and Silva, C. E.. American Mathematical Society, Providence, RI, 2016, pp. 245–253.CrossRefGoogle Scholar
Kułaga-Przymus, J. and Lemańczyk, M. D. Hereditary subshifts whose measure of maximal entropy does not have the Gibbs property. Colloq. Math. 166(1) (2021), 107–127.CrossRefGoogle Scholar
Kułaga-Przymus, J., Lemańczyk, M. D. and Rams, M.. Basic thermodynamical formalism for sandwich subshifts. Preprint, 2024, https://arxiv.org/pdf/2402.12579.pdf.Google Scholar
Kwietniak, D.. Topological entropy and distributional chaos in hereditary shifts with applications to spacing shifts and beta shifts. Discrete Contin. Dyn. Syst. 33(6) (2013), 2451–2467.CrossRefGoogle Scholar
Kwietniak, D., Łącka, M. and Oprocha, P.. Generic points for dynamical systems with average shadowing. Monatsh. Math. 183(4) (2017), 625–648.CrossRefGoogle Scholar
Lemańczyk, M. D.. Recurrence of stochastic processes in some concentration of measure and entropy problems. Available at: https://www.mimuw.edu.pl/sites/default/files/lemanczyk_rozprawa_doktorska.pdf.Google Scholar
Lindenstrauss, J., Olsen, G. and Sternfeld, Y.. The Poulsen simplex. Ann. Inst. Fourier (Grenoble) 28(1) (1978), vi, 91–114.CrossRefGoogle Scholar
Mirsky, L.. Note on an asymptotic formula connected with
$r$
-free integers. Q. J. Math. Oxford Ser. 18 (1947), 178–182.CrossRefGoogle Scholar
$r$
-free integers. Q. J. Math. Oxford Ser. 18 (1947), 178–182.CrossRefGoogle ScholarMirsky, L.. Arithmetical pattern problems relating to divisibility by
$r$
th powers. Proc. Lond. Math. Soc. (2) 50 (1949), 497–508.Google Scholar
$r$
th powers. Proc. Lond. Math. Soc. (2) 50 (1949), 497–508.Google ScholarPeckner, R.. Uniqueness of the measure of maximal entropy for the squarefree flow. Israel J. Math. 210(1) (2015), 335–357.CrossRefGoogle Scholar
Pfister, C.-E. and Sullivan, W. G.. Large deviations estimates for dynamical systems without the specification property. Applications to the β-shifts. Nonlinearity 18(1) (2005), 237–261.Google Scholar
Sarnak, P.. Three lectures on the Möbius function, randomness and dynamics. Available at: http://publications.ias.edu/sarnak/.Google Scholar
Williams, S.. Toeplitz minimal flows which are not uniquely ergodic. Z. Wahrscheinlichkeitstheorie und Verw. Gebiete 67(1) (1984), 95–107.Google Scholar