Hostname: page-component-7479d7b7d-68ccn Total loading time: 0 Render date: 2024-07-10T12:23:24.083Z Has data issue: false hasContentIssue false
  • English
  • Français

K-stability of birationally superrigid Fano varieties

Part of: Surfaces and higher-dimensional varieties Birational geometry Complex manifolds

Published online by Cambridge University Press:  07 August 2019

Charlie Stibitz  and
Ziquan Zhuang
Charlie Stibitz
Affiliation:
Department of Mathematics, Northwestern University, Evanston, IL 60208, USA email cstibitz@math.northwestern.edu
Ziquan Zhuang
Affiliation:
Department of Mathematics, Princeton University, Princeton, NJ 08544-1000, USA email zzhuang@math.princeton.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

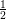We prove that every birationally superrigid Fano variety whose alpha invariant is greater than (respectively no smaller than) $\frac{1}{2}$ is K-stable (respectively K-semistable). We also prove that the alpha invariant of a birationally superrigid Fano variety of dimension $n$ is at least 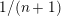$1/(n+1)$ (under mild assumptions) and that the moduli space (if it exists) of birationally superrigid Fano varieties is separated.

Keywords

K-stabilitybirational superrigidityKähler–Einstein metricsFano varieties

MSC classification

Primary: 14J45: Fano varieties 14E05: Rational and birational maps 32Q20: Kähler-Einstein manifolds
Type
Research Article
Information
Compositio Mathematica , Volume 155 , Issue 9 , September 2019 , pp. 1845 - 1852
Copyright
© The Authors 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Blum, H. and Jonsson, M., Thresholds, valuations, and K-stability, Preprint (2017),arXiv:1706.04548.Google Scholar
Blum, H. and Xu, C., Uniqueness of K-polystable degenerations of Fano varieties, Ann. of Math., to appear. Preprint (2018), arXiv:1812.03538.Google Scholar
Cheltsov, I. A., Log canonical thresholds on hypersurfaces , Mat. Sb. 192 (2001), 155–172.Google Scholar
Cheltsov, I., On singular cubic surfaces , Asian J. Math. 13 (2009), 191–214.Google Scholar
Cheltsov, I. A. and Shramov, K. A., Log-canonical thresholds for nonsingular Fano threefolds , Uspekhi Mat. Nauk 63 (2008), 73–180.Google Scholar
de Fernex, T., Erratum to: Birationally rigid hypersurfaces , Invent. Math. 203 (2016), 675–680.Google Scholar
de Fernex, T., Ein, L. and Mustaţă, M., Bounds for log canonical thresholds with applications to birational rigidity , Math. Res. Lett. 10 (2003), 219–236.Google Scholar
de Fernex, T. and Hacon, C. D., Deformations of canonical pairs and Fano varieties , J. Reine Angew. Math. 651 (2011), 97–126.Google Scholar
Donaldson, S. K., Scalar curvature and stability of toric varieties , J. Differential Geom. 62 (2002), 289–349.Google Scholar
Ein, L., Lazarsfeld, R., Mustaţă, M., Nakamaye, M. and Popa, M., Restricted volumes and base loci of linear series , Amer. J. Math. 131 (2009), 607–651.Google Scholar
Fujita, K., A valuative criterion for uniform K-stability of ℚ-Fano varieties , J. Reine Angew. Math. 751 (2019), 309–338.Google Scholar
Fujita, K., K-stability of Fano manifolds with not small alpha invariants , J. Inst. Math. Jussieu 18 (2019), 519–530.Google Scholar
Fujita, K., Uniform K-stability and plt blowups of log Fano pairs , Kyoto J. Math. 59 (2019), 399–418.Google Scholar
Fujita, K. and Odaka, Y., On the K-stability of Fano varieties and anticanonical divisors , Tohoku Math. J. (2) 70 (2018), 511–521.Google Scholar
Iskovskih, V. A. and Manin, Ju. I., Three-dimensional quartics and counterexamples to the Lüroth problem , Mat. Sb. (N.S.) 86 (1971), 140–166.Google Scholar
Kim, I.-K., Okada, T. and Won, J., Alpha invariants of birationally rigid Fano threefolds , Int. Math. Res. Not. IMRN 2018 (2018), 2745–2800.Google Scholar
Kollár, J., Singularities of pairs , in Algebraic geometry—Santa Cruz 1995, Proceedings of Symposia in Pure Mathematics, vol. 62 (American Mathematical Society, Providence, RI, 1997), 221–287.Google Scholar
Lazarsfeld, R., Positivity in algebraic geometry I. Classical setting: line bundles and linear series, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge [A Series of Modern Surveys in Mathematics], vol. 48 (Springer, Berlin, 2004).Google Scholar
Li, C., K-semistability is equivariant volume minimization , Duke Math. J. 166 (2017), 3147–3218.Google Scholar
Li, C., Wang, X. and Xu, C., On the proper moduli spaces of smoothable Kähler–Einstein Fano varieties , Duke Math. J. 168 (2019), 1387–1459.Google Scholar
Odaka, Y. and Okada, T., Birational superrigidity and slope stability of Fano manifolds , Math. Z. 275 (2013), 1109–1119.Google Scholar
Pukhlikov, A. V., Birationally rigid Fano hypersurfaces , Izv. Ross. Akad. Nauk Ser. Mat. 66 (2002), 159–186.Google Scholar
Pukhlikov, A. V., Birational geometry of Fano hypersurfaces of index two , Math. Ann. 366 (2016), 721–782.Google Scholar
Pukhlikov, A. V., Birational geometry of singular Fano hypersurfaces of index two, Preprint (2017), arXiv:1712.08796.Google Scholar
Tian, G., Kähler–Einstein metrics with positive scalar curvature , Invent. Math. 130 (1997), 1–37.Google Scholar
Zhuang, Z., Birational superrigidity and K-stability of Fano complete intersections of index one (with an appendix written jointly with Charlie Stibitz), Preprint (2018), arXiv:1802.08389.Google Scholar