No CrossRef data available.
Article contents
BPS invariants from p-adic integrals
Published online by Cambridge University Press: 30 May 2024
Abstract
We define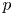 
$p$-adic 
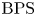$\mathrm {BPS}$ or 
$p\mathrm {BPS}$ invariants for moduli spaces 
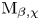$\operatorname {M}_{\beta,\chi }$ of one-dimensional sheaves on del Pezzo and K3 surfaces by means of integration over a non-archimedean local field 
$F$. Our definition relies on a canonical measure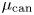 
$\mu _{\rm can}$ on the 
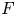$F$-analytic manifold associated to 
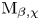$\operatorname {M}_{\beta,\chi }$ and the 
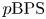$p\mathrm {BPS}$ invariants are integrals of natural 
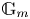${\mathbb {G}}_m$ gerbes with respect to 
$\mu _{\rm can}$. A similar construction can be done for meromorphic and usual Higgs bundles on a curve. Our main theorem is a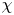 
$\chi$-independence result for these 
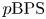$p\mathrm {BPS}$ invariants. For one-dimensional sheaves on del Pezzo surfaces and meromorphic Higgs bundles, we obtain as a corollary the agreement of 
$p\mathrm {BPS}$ with usual 
$\mathrm {BPS}$ invariants through a result of Maulik and Shen [Cohomological 
$\chi$-independence for moduli of one-dimensional sheaves and moduli of Higgs bundles, Geom. Topol. 27 (2023), 1539–1586].
- Type
- Research Article
- Information
- Copyright
- © 2024 The Author(s). The publishing rights in this article are licensed to Foundation Compositio Mathematica under an exclusive licence
References
Alper, J., Halpern-Leistner, D. and Heinloth, J., Existence of moduli spaces for algebraic stacks, Invent. Math. 234 (2023), 949–1038.CrossRefGoogle Scholar
Altman, A. B. and Kleiman, S. L., Compactifying the Picard scheme, Adv. Math. 35 (1980), 50–112.CrossRefGoogle Scholar
Arbarello, E. and Saccà, G., Singularities of moduli spaces of sheaves on K3 surfaces and Nakajima quiver varieties, Adv. Math. 329 (2018), 649–703.CrossRefGoogle Scholar
Bayer, A., Lahoz, M., Macrì, E., Nuer, H., Perry, A. and Stellari, P., Stability conditions in families, Publ. Math. Inst. Hautes Études Sci. 133 (2021), 157–325.CrossRefGoogle Scholar
Beilinson, A., Bernstein, J., Deligne, P. and Gabber, O., Faisceaux pervers (Société Mathématique de France, Paris, 2018).Google Scholar
Ben-Bassat, O., Brav, C., Bussi, V. and Joyce, D., A ‘Darboux theorem’ for shifted symplectic structures on derived Artin stacks, with applications, Geom. Topol. 19 (2015), 1287–1359.CrossRefGoogle Scholar
Bosch, S., Lütkebohmert, W. and Raynaud, M., Néron models, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge/A Series of Modern Surveys in Mathematics, vol. 21 (Springer, 2012).Google Scholar
Bousseau, P., A proof of N. Takahashi's conjecture for 
$(\mathbb {P}^2,E)$ and a refined sheaves/Gromov–Witten correspondence, Duke Math. J. 172 (2023), 2895–2955.Google Scholar
$(\mathbb {P}^2,E)$ and a refined sheaves/Gromov–Witten correspondence, Duke Math. J. 172 (2023), 2895–2955.Google ScholarBrav, C., Bussi, V., Dupont, D., Joyce, D. and Szendroi, B., Symmetries and stabilization for sheaves of vanishing cycles, J. Singul. 11 (2015), 85–151.Google Scholar
Brochard, S., Duality for commutative group stacks, Int. Math. Res. Not. IMRN 2021 (2021), 2321–2388.CrossRefGoogle Scholar
Budur, N. and Zhang, Z., Formality conjecture for K3 surfaces, Compos. Math. 155 (2019), 902–911.CrossRefGoogle Scholar
Chambert-Loir, A., Nicaise, J. and Sebag, J., Motivic integration (Springer, 2018), 305–361.CrossRefGoogle Scholar
Chaudouard, P.-H. and Laumon, G., Un théorème du support pour la fibration de Hitchin, Ann. Inst. Fourier (Grenoble) 66 (2016), 711–727.CrossRefGoogle Scholar
Crawley-Boevey, W., Geometry of the moment map for representations of quivers, Compos. Math. 126 (2001), 257–293.Google Scholar
Davison, B., Hennecart, L. and Mejia, S. S., BPS Lie algebras for totally negative 2-Calabi-Yau categories and nonabelian Hodge theory for stacks, Preprint (2022), arXiv:2212.07668v2.Google Scholar
Davison, B., Hennecart, L. and Mejia, S. S., BPS algebras and generalised Kac-Moody algebras from 2-Calabi-Yau categories, Preprint (2023), arXiv:2303.12592v3.Google Scholar
Davison, B. and Meinhardt, S., Donaldson-Thomas theory for categories of homological dimension one with potential, Preprint (2015), arXiv:1512.08898.Google Scholar
Davison, B. and Meinhardt, S., Cohomological Donaldson–Thomas theory of a quiver with potential and quantum enveloping algebras, Invent. Math. 221 (2020), 777–871.CrossRefGoogle Scholar
Deligne, P. and Mumford, D., The irreducibility of the space of curves of given genus, Publ. Math. Inst. Hautes Études Sci. 36 (1969), 75–109.CrossRefGoogle Scholar
Fantechi, B., Goettsche, L. and Van Sraten, D., Euler number of the compactified Jacobian and multiplicity of rational curves, J. Algebraic Geom. 8 (1999), 115–133.Google Scholar
Groechenig, M., Wyss, D. and Ziegler, P., Geometric stabilisation via p-adic integration, J. Amer. Math. Soc. 33 (2020), 807–873.Google Scholar
Groechenig, M., Wyss, D. and Ziegler, P., Mirror symmetry for moduli spaces of Higgs bundles via $p$-adic integration, Invent. Math. 221 (2020), 505–596.CrossRefGoogle Scholar
$p$-adic integration, Invent. Math. 221 (2020), 505–596.CrossRefGoogle ScholarGrothendieck, A., Éléments de géométrie algébrique: IV. Etude locale des schémas et des morphismes de schémas, Seconde partie, Publ. Math. Inst. Hautes Études Sci. 24 (1965), 5–231.Google Scholar
Grothendieck, A., Éléments de géométrie algébrique: IV. Etude locale des schémas et des morphismes de schémas, Troisième partie, Publ. Math. Inst. Hautes Études Sci. 28 (1966), 5–255.CrossRefGoogle Scholar
Hartshorne, R., Algebraic geometry, Graduate Texts in Mathematics, vol. 52 (Springer, New York, 1977); MR 0463157 (57 #3116).CrossRefGoogle Scholar
Hitchin, N., Stable bundles and integrable systems, Duke Math. J. 54 (1987), 91–114.CrossRefGoogle Scholar
Huybrechts, D. and Lehn, M., The geometry of moduli spaces of sheaves, Aspects of Mathematics, vol. E31 (Friedr. Vieweg & Sohn, Braunschweig, 1997); MR 1450870 (98g:14012).CrossRefGoogle Scholar
Huybrechts, D. and Thomas, R. P., Deformation-obstruction theory for complexes via Atiyah and Kodaira–Spencer classes, Math. Ann. 346 (2010), 545–569.CrossRefGoogle Scholar
Igusa, J., An introduction to the theory of local zeta functions, AMS/IP Studies in Advanced Mathematics, vol. 14 (American Mathematical Society, Providence, RI, Cambridge, MA, 2000); MR 1743467.Google Scholar
Inaba, M.-A., Smoothness of the moduli space of complexes of coherent sheaves on an abelian or a projective K3 surface, Adv. Math. 227 (2011), 1399–1412.CrossRefGoogle Scholar
Joyce, D. D. and Song, Y., A theory of generalized Donaldson-Thomas invariants (American Mathematical Society, 2012).CrossRefGoogle Scholar
Katz, S., Genus zero Gopakumar-Vafa invariants of contractible curves, J. Differential Geom. 79 (2008), 185–195.Google Scholar
Keel, S. and Mori, S., Quotients by groupoids, Ann. of Math. (2) 145 (1997), 193–213; MR 1432041 (97m:14014).CrossRefGoogle Scholar
Kinjo, T. and Koseki, N., Cohomological $\chi$-independence for Higgs bundles and Gopakumar-Vafa invariants, J. Eur. Math. Soc., to appear. Preprint (2021), arXiv:2112.10053.Google Scholar
$\chi$-independence for Higgs bundles and Gopakumar-Vafa invariants, J. Eur. Math. Soc., to appear. Preprint (2021), arXiv:2112.10053.Google ScholarKontsevich, M. and Soibelman, Y., Stability structures, motivic Donaldson-Thomas invariants and cluster transformations, Preprint (2008), arXiv:0811.2435.Google Scholar
Langer, A., Moduli spaces of sheaves in mixed characteristic, Duke Math. J. 124 (2004), 571–586.CrossRefGoogle Scholar
Langer, A., Semistable sheaves in positive characteristic, Ann. of Math. (2) 159 (2004), 251–276.CrossRefGoogle Scholar
Langton, S. G., Valuative criteria for families of vector bundles on algebraic varieties, Ann. of Math. (2) 101 (1975), 88–110; MR 0364255 (51 #510).CrossRefGoogle Scholar
Lichtenbaum, S., Duality theorems for curves over p-adic fields, Invent. Math. 7 (1969), 120–136.CrossRefGoogle Scholar
Lieblich, M., Moduli of complexes on a proper morphism, J. Algebraic Geom. 15 (2006), 175–206.CrossRefGoogle Scholar
Liu, Q., Algebraic geometry and arithmetic curves, Oxford Graduate Texts in Mathematics, vol. 6 (Oxford University Press, Oxford, 2006).Google Scholar
López-Martín, A.C., Simpson Jacobians of reducible curves, J. Reine Angew. Math. 582 (2005), 1–39.CrossRefGoogle Scholar
Maulik, D. and Shen, J., Cohomological $\chi$-independence for moduli of one-dimensional sheaves and moduli of Higgs bundles, Geom. Topol. 27 (2023), 1539–1586.CrossRefGoogle Scholar
$\chi$-independence for moduli of one-dimensional sheaves and moduli of Higgs bundles, Geom. Topol. 27 (2023), 1539–1586.CrossRefGoogle ScholarMaulik, D. and Thomas, R. P., Sheaf counting on local K3 surfaces, Pure Appl. Math. Q. 14 (2019), 419–441.CrossRefGoogle Scholar
Maulik, D. and Toda, Y., Gopakumar–Vafa invariants via vanishing cycles, Invent. Math. 213 (2018), 1017–1097.CrossRefGoogle Scholar
Meinhardt, S., Donaldson–Thomas invariants vs. intersection cohomology for categories of homological dimension one, Preprint (2015), arXiv:1512.03343.Google Scholar
Mellit, A., Poincaré polynomials of moduli spaces of Higgs bundles and character varieties (no punctures), Invent. Math. 221 (2020), 301–327.Google Scholar
Melo, M., Rapagnetta, A. and Viviani, F., Fine compactified Jacobians of reduced curves, Trans. Amer. Math. Soc. 369 (2017), 5341–5402.CrossRefGoogle Scholar
Milne, J. S., Arithmetic duality theorems, Perspectives in Mathematics, vol. 1 (Academic Press, Boston, MA, 1986); MR 881804.Google Scholar
Mozgovoy, S. and O'Gorman, R., Counting twisted Higgs bundles, Preprint (2019), arXiv:1901.02439Google Scholar
Mozgovoy, S. and Schiffmann, O., Counting Higgs bundles, Preprint (2014), arXiv:1411.2101.Google Scholar
Mukai, S., Symplectic structure of the moduli space of sheaves on an abelian or K3 surface, Invent. Math. 77 (1984), 101–116.CrossRefGoogle Scholar
Ngô, B. C., Fibration de Hitchin et endoscopie, Invent. Math. 164 (2006), 399–453; MR 2218781 (2007k:14018).CrossRefGoogle Scholar
Nitsure, N., Moduli space of semistable pairs on a curve, Proc. Lond. Math. Soc. (3) 62 (1991), 275–300; MR 1085642 (92a:14010).CrossRefGoogle Scholar
Pandharipande, R. and Thomas, R., Stable pairs and BPS invariants, J. Amer. Math. Soc. 23 (2010), 267–297.CrossRefGoogle Scholar
Poonen, B. and Stoll, M., The Cassels–Tate pairing on polarized abelian varieties, Ann. of Math. 150 (1999), 1109–1149.CrossRefGoogle Scholar
Rego, C. J., The compactified Jacobian, Ann. Sci. Éc. Norm. Supér. (4) 13 (1980), 211–223; MR 584085 (81k:14006).CrossRefGoogle Scholar
Schoutens, H., The use of ultraproducts in commutative algebra (Springer, 2010).CrossRefGoogle Scholar
Schiffmann, O., Indecomposable vector bundles and stable Higgs bundles over smooth projective curves, Ann. of Math. 183 (2016), 297–362.CrossRefGoogle Scholar
Serre, J.-P., Corps locaux, Deuxième édition, Publications de l'Université de Nancago, vol. VIII (Hermann, Paris, 1968); MR 0354618 (50 #7096).Google Scholar
Seshadri, C. S., Geometric reductivity over arbitrary base, Adv. Math. 26 (1977), 225–274.CrossRefGoogle Scholar
Stacks Project Authors, Stacks project, http://math.columbia.edu/algebraic_geometry/stacks-git.Google Scholar
Thomas, R. P., A holomorphic Casson invariant for Calabi-Yau 3-folds, and bundles on K3 fibrations, J. Differential Geom. 54 (2000), 367–438.CrossRefGoogle Scholar
Toda, Y., Multiple cover formula of generalized DT invariants I: parabolic stable pairs, Adv. Math. 257 (2014), 476–526.CrossRefGoogle Scholar
Toda, Y., Gopakumar–Vafa invariants and wall-crossing, J. Differential Geom. 123 (2023), 141–193.Google Scholar
Travkin, R., Quantum geometric Langlands correspondence in positive characteristic: The $\mathrm {GL}_{N}$ case, Duke Math. J. 165 (2016), 1283–1361.CrossRefGoogle Scholar
$\mathrm {GL}_{N}$ case, Duke Math. J. 165 (2016), 1283–1361.CrossRefGoogle ScholarVernet, T., Rational singularities for moment maps of totally negative quivers, Preprint (2022), arXiv:2209.14791.Google Scholar
Yasuda, T., The wild McKay correspondence and $p$-adic measures, J. Eur. Math. Soc. (JEMS) 19 (2017), 3709–3743.CrossRefGoogle Scholar
$p$-adic measures, J. Eur. Math. Soc. (JEMS) 19 (2017), 3709–3743.CrossRefGoogle ScholarYu, H., Comptage des systémes locaux $\ell$-adiques sur une courbe, Ann. Math. 197 (2023), 423–531.CrossRefGoogle Scholar
$\ell$-adiques sur une courbe, Ann. Math. 197 (2023), 423–531.CrossRefGoogle ScholarYuan, Y., Sheaves on non-reduced curves in a projective surface, Sci. China Math. 66 (2023), 237–250.CrossRefGoogle Scholar