Hostname: page-component-84b7d79bbc-fnpn6 Total loading time: 0 Render date: 2024-07-26T20:24:27.386Z Has data issue: false hasContentIssue false
  • English
  • Français

Colouring Planar Graphs With Three Colours and No Large Monochromatic Components

Published online by Cambridge University Press:  01 April 2014

LOUIS ESPERET  and
GWENAËL JORET
LOUIS ESPERET
Affiliation:
Laboratoire G-SCOP (CNRS, Grenoble-INP), Grenoble, France (e-mail: louis.esperet@g-scop.fr)
GWENAËL JORET
Affiliation:
Department of Mathematics and Statistics, The University of Melbourne, Melbourne, Australia (e-mail: gwenael.joret@unimelb.edu.au)
Get access Rights & Permissions [Opens in a new window]

Abstract

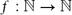We prove the existence of a function $f :\mathbb{N} \to \mathbb{N}$ such that the vertices of every planar graph with maximum degree Δ can be 3-coloured in such a way that each monochromatic component has at most f(Δ) vertices. This is best possible (the number of colours cannot be reduced and the dependence on the maximum degree cannot be avoided) and answers a question raised by Kleinberg, Motwani, Raghavan and Venkatasubramanian in 1997. Our result extends to graphs of bounded genus.

Keywords

Primary 05C15Secondary 05C10
Type
Paper
Information
Combinatorics, Probability and Computing , Volume 23 , Issue 4 , July 2014 , pp. 551 - 570
Copyright
Copyright © The Authors 2014. Published by Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1]Alon, N., Ding, G., Oporowski, B. and Vertigan, D. (2003) Partitioning into graphs with only small components. J. Combin. Theory Ser. B 87 231–243.Google Scholar
[2]Berke, R. (2008) Coloring and transversals of graphs. PhD thesis, ETH Zürich. Dissertation 17797.Google Scholar
[3]Grötzsch, H. (1959) Ein Dreifarbensatz für dreikreisfreie Netze auf der Kugel. Wiss. Z. Martin-Luther-Univ. Halle-Wittenberg Math.-Natur. Reihe 8 109–120.Google Scholar
[4]Haxell, P., Szabó, T. and Tardos, G. (2003) Bounded size components: Partitions and transversals. J. Combin. Theory Ser. B 88 281–297.Google Scholar
[5]Kawarabayashi, K. and Mohar, B. (2007) A relaxed Hadwiger's conjecture for list colorings. J. Combin. Theory Ser. B 97 647–651.Google Scholar
[6]Kawarabayashi, K. and Thomassen, C. (2012) From the plane to higher surfaces. J. Combin. Theory Ser. B 102 852–868.Google Scholar
[7]Kleinberg, J., Motwani, R., Raghavan, P. and Venkatasubramanian, S. (1997) Storage management for evolving databases. In Proc. 38th Annual IEEE Symposium on Foundations of Computer Science: FOCS 1997, pp. 353–362.Google Scholar
[8]Kostochka, A. (1984) Lower bound of the Hadwiger number of graphs by their average degree. Combinatorica 4 307–316.Google Scholar
[9]Linial, N., Matoušek, J., Sheffet, O. and Tardos, G. (2008) Graph colouring with no large monochromatic components. Combin. Probab. Comput. 17 577–589.Google Scholar
[10]Linial, N. and Saks, M. (1993) Low diameter graph decompositions. Combinatorica 13 441–454.Google Scholar
[11]Mohar, B. and Thomassen, C. (2001) Graphs on Surfaces, The Johns Hopkins University Press.CrossRefGoogle Scholar
[12]Thomason, A. (1984) An extremal function for contractions of graphs. Math. Proc. Cambridge Philos. Soc. 95 261–265.Google Scholar
[13]Wood, D. R. (2010) Contractibility and the Hadwiger conjecture. Europ. J. Combin. 31 2102–2109.Google Scholar