Hostname: page-component-7479d7b7d-fwgfc Total loading time: 0 Render date: 2024-07-13T13:31:36.294Z Has data issue: false hasContentIssue false
  • English
  • Français

2-Local Isometries on Spaces of Lipschitz Functions

Published online by Cambridge University Press:  20 November 2018

A. Jiménez-Vargas  and
Moisés Villegas-Vallecillos
A. Jiménez-Vargas
Affiliation:
Departamento de Álgebra y Análisis Matemático, Universidad de Almería, 04120 Almería, Spaine-mail: ajimenez@ual.ese-mail: mvv042@alboran.ual.es
Moisés Villegas-Vallecillos
Affiliation:
Departamento de Álgebra y Análisis Matemático, Universidad de Almería, 04120 Almería, Spaine-mail: ajimenez@ual.ese-mail: mvv042@alboran.ual.es
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

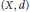Let $(X,\,d)$ be a metric space, and let 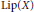$\text{Lip(}X\text{)}$ denote the Banach space of all scalar-valued bounded Lipschitz functions 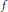$f$ on 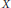$X$ endowed with one of the natural norms

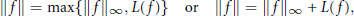$$\left\| f \right\|\,=\,\max \{{{\left\| f \right\|}_{\infty }},\,L(f)\}\,\,\text{or}\,\,\left\| f \right\|\,=\,{{\left\| f \right\|}_{\infty }}\,+\,L(f),$$

where $L(f)$ is the Lipschitz constant of 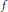$f$. It is said that the isometry group of 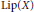$\text{Lip(}X\text{)}$ is canonical if every surjective linear isometry of 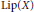$\text{Lip(}X\text{)}$ is induced by a surjective isometry of 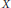$X$. In this paper we prove that if 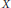$X$ is bounded separable and the isometry group of 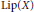$\text{Lip(}X\text{)}$ is canonical, then every 2-local isometry of 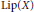$\text{Lip(}X\text{)}$ is a surjective linear isometry. Furthermore, we give a complete description of all 2-local isometries of 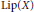$\text{Lip(}X\text{)}$ when 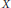$X$ is bounded.

Keywords

46B0446J1046E15isometrylocal isometryLipschitz function
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 4 , 01 December 2011 , pp. 680 - 692
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Chebotar, M. A., Ke, W.-F., Lee, P.-H., and Shiao, L.-S., On maps preserving products. Canad. Math. Bull. 48(2005), no. 3, 355–369. doi:10.4153/CMB-2005-033-8Google Scholar
[2] Győory, M., 2-local isometries of (X). Acta Sci. Math. (Szeged) 67(2001), no. 3–4, 735–746.Google Scholar
[3] Hatori, O., Miura, T., Oka, H., and Takagi, H., 2-local isometries and 2-local automorphisms on uniform algebras. Int. Math. Forum 2(2007), no. 49–52, 2491–2502.Google Scholar
[4] Jarosz, K. and Pathak, V., Isometries between function spaces. Trans. Amer. Math. Soc. 305(1998), no. 1, 193–206. doi:10.1090/S0002-9947-1988-0920154-7Google Scholar
[5] Ji, P. S. and Wei, C. P., Isometries and 2-local isometries on Cartan bimodule algebras in hyperfinite factors of type II 1 . (Chinese) Acta Math. Sinica (Chin. Ser.) 49(2006), no. 1, 51–58.Google Scholar
[6] Kim, S. O. and Kim, J. S., Local automorphisms and derivations on certain C*-algebras. Proc. Amer. Math. Soc. 133(2005), no. 11, 3303–3307. doi:10.1090/S0002-9939-05-08059-7Google Scholar
[7] Lin, Y.-F. and Wong, T.-L., A note on 2-local maps. Proc. Edinb. Math. Soc. 49(2006), no. 3, 701–708. doi:10.1017/S0013091504001142Google Scholar
[8] Liu, J.-H. and Wong, N.-C., 2-local automorphisms of operator algebras. J. Math. Anal. Appl. 321(2006), no. 2, 741–750. doi:10.1016/j.jmaa.2005.09.003Google Scholar
[9] Molnár, L. and Šemrl, P., Local automorphisms of the unitary group and the general linear group on a Hilbert space. Exp. Math. 18(2000), no. 3, 231–238.Google Scholar
[10] Molnár, L., 2-local isometries of some operator algebras. Proc. Edinb. Math. Soc. 45(2002), no. 2, 349–352.Google Scholar
[11] Molnár, L., Local automorphisms of operator algebras on Banach spaces. Proc. Amer. Math. Soc. 131(2003), no. 6, 1867–1874. doi:10.1090/S0002-9939-02-06786-2Google Scholar
[12] Rao, N. V. and Roy, A. K., Linear isometries of some function spaces.. Pacific J. Math. 38(1971), 177–192.Google Scholar
[13] Roy, A. K., Extreme points and linear isometries of the Banach space of Lipschitz functions.. Canad. J. Math. 20(1968), 1150–1164. doi:10.4153/CJM-1968-109-9Google Scholar
[14] Šemrl, P., Local automorphisms and derivations on B(H) . Proc. Amer. Math. Soc. 125(1997), no. 9, 2677–2680. doi:10.1090/S0002-9939-97-04073-2Google Scholar
[15] Vasavada, M. H., Closed ideals and linear isometries of certain function spaces. Ph.D. Thesis, University of Wisconsin, 1969.Google Scholar
[16] Weaver, N., Isometries of noncompact Lipschitz spaces. Canad. Math. Bull. 38(1995), no. 2, 242–249. doi:10.4153/CMB-1995-035-3Google Scholar
[17] Xie, J. and Lu, F., A note on 2-local automorphisms of diagraph algebras. Linear Algebra Appl. 378(2004), 93–98. doi:10.1016/j.laa.2003.09.004Google Scholar
[18] Zhang, J. H., Yang, A. L., and Pan, F. F., Local automorphisms of nest subalgebras of factor von Neumann algebras.. Linear Algebra Appl. 402(2005), 335–344. doi:10.1016/j.laa.2005.01.005Google Scholar