No CrossRef data available.
Article contents
SUBADDITIVITY OF AN INTEGRAL TRANSFORM FOR POSITIVE OPERATORS IN HILBERT SPACES
Published online by Cambridge University Press: 08 November 2022
Abstract
For a continuous and positive function
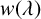 $w(\lambda )$
,
$w(\lambda )$
,
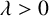 $\lambda>0$
and
$\lambda>0$
and
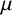 $\mu $
a positive measure on
$\mu $
a positive measure on
 $(0,\infty )$
, we consider the integral transform
$(0,\infty )$
, we consider the integral transform
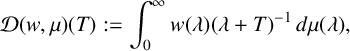 $$ \begin{align*} \mathcal{D}( w,\mu ) ( T) :=\int_{0}^{\infty }w(\lambda) ( \lambda +T) ^{-1}\,d\mu ( \lambda ) , \end{align*} $$
$$ \begin{align*} \mathcal{D}( w,\mu ) ( T) :=\int_{0}^{\infty }w(\lambda) ( \lambda +T) ^{-1}\,d\mu ( \lambda ) , \end{align*} $$
where the integral is assumed to exist for T a positive operator on a complex Hilbert space H. We show among other things that if B,
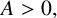 $A>0,$
then
$A>0,$
then
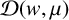 $\mathcal {D}( w,\mu ) $
is operator subadditive on
$\mathcal {D}( w,\mu ) $
is operator subadditive on
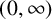 $(0,\infty ) $
, that is,
$(0,\infty ) $
, that is,
 $$ \begin{align*} \mathcal{D}( w,\mu ) ( A) +\mathcal{D}( w,\mu) ( B) \geq \mathcal{D}( w,\mu )(A+B). \end{align*} $$
$$ \begin{align*} \mathcal{D}( w,\mu ) ( A) +\mathcal{D}( w,\mu) ( B) \geq \mathcal{D}( w,\mu )(A+B). \end{align*} $$
From this, we derive that if
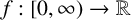 $f:[0,\infty )\rightarrow \mathbb {R}$
is an operator monotone function on
$f:[0,\infty )\rightarrow \mathbb {R}$
is an operator monotone function on
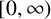 $[0,\infty )$
, then the function
$[0,\infty )$
, then the function
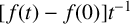 $[ f( t) -f( 0) ] t^{-1}$
is operator subadditive on
$[ f( t) -f( 0) ] t^{-1}$
is operator subadditive on
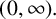 $( 0,\infty ) .$
Also, if
$( 0,\infty ) .$
Also, if
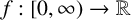 $f:[0,\infty )\rightarrow \mathbb {R}$
is an operator convex function on
$f:[0,\infty )\rightarrow \mathbb {R}$
is an operator convex function on
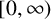 $[0,\infty )$
, then the function
$[0,\infty )$
, then the function
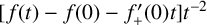 $[ f( t) -f( 0) -f_{+}^{\prime }( 0) t ] t^{-2}$
is operator subadditive on
$[ f( t) -f( 0) -f_{+}^{\prime }( 0) t ] t^{-2}$
is operator subadditive on
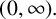 $( 0,\infty ) .$
$( 0,\infty ) .$
Keywords
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.





