Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-30T14:44:24.569Z Has data issue: false hasContentIssue false
  • English
  • Français

A symmetry-breaking phenomenon and asymptotic profiles of least-energy solutions to a nonlinear Schrödinger equation

Published online by Cambridge University Press:  12 July 2007

Kazuhiro Kurata ,
Tatsuya Watanabe  and
Masataka Shibata
Kazuhiro Kurata
Affiliation:
Department of Mathematics, Tokyo Metropolitan University, 1-1 Minami-osawa, Hachioji-shi, Tokyo 192-0397, Japan
Tatsuya Watanabe
Affiliation:
Department of Mathematics, Tokyo Metropolitan University, 1-1 Minami-osawa, Hachioji-shi, Tokyo 192-0397, Japan
Masataka Shibata
Affiliation:
Department of Mathematics, Tokyo Institute of Technology University, 2-12-1 Oh-okayama, Meguro-ku, Tokyo 152-8551, Japan
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we study a symmetry-breaking phenomenon of a least-energy solution to a nonlinear Schrödinger equation 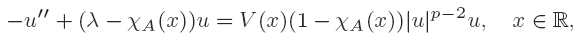under suitable assumptions on V(x), where λ > 1, p > 2 and χA is the characteristic function of the set A = [−(l + 2), −l] ∪ [l,l + 2] with l > 0. We also study asymptotic profiles of least-energy solutions for the singularly perturbed problem 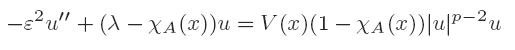for small ε > 0.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 135 , Issue 2 , April 2005 , pp. 357 - 392
Copyright
Copyright © Royal Society of Edinburgh 2005

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)