Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Guo, Victor J. W.
2019.
Common q-Analogues of Some Different Supercongruences.
Results in Mathematics,
Vol. 74,
Issue. 4,
Guo, Victor J.W.
2020.
q-Supercongruences modulo the fourth power of a cyclotomic polynomial via creative microscoping.
Advances in Applied Mathematics,
Vol. 120,
Issue. ,
p.
102078.
Jana, Arijit
and
Kalita, Gautam
2020.
Supercongruences for sums involving fourth power of some rising factorials.
Proceedings - Mathematical Sciences,
Vol. 130,
Issue. 1,
Guo, Victor J. W.
and
Schlosser, Michael J.
2020.
A family of q-hypergeometric congruences modulo the fourth power of a cyclotomic polynomial.
Israel Journal of Mathematics,
Vol. 240,
Issue. 2,
p.
821.
Guo, Victor J.W.
2020.
Proof of a generalization of the (B.2) supercongruence of Van Hamme through a q-microscope.
Advances in Applied Mathematics,
Vol. 116,
Issue. ,
p.
102016.
Guo, Victor J. W.
and
Schlosser, Michael J.
2020.
A New Family of q-Supercongruences Modulo the Fourth Power of a Cyclotomic Polynomial.
Results in Mathematics,
Vol. 75,
Issue. 4,
Guo, Victor J.W.
2020.
q-Analogues of Dwork-type supercongruences.
Journal of Mathematical Analysis and Applications,
Vol. 487,
Issue. 2,
p.
124022.
Guo, Victor J. W.
2020.
Proof of Some q-Supercongruences Modulo the Fourth Power of a Cyclotomic Polynomial.
Results in Mathematics,
Vol. 75,
Issue. 3,
Li, Long
and
Wang, Su-Dan
2020.
Proof of a q-supercongruence conjectured by Guo and Schlosser.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 114,
Issue. 4,
Guo, Victor J. W.
2020.
A q-analogue of the (A.2) supercongruence of Van Hamme for primes $$p\equiv 1\pmod {4}$$.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 114,
Issue. 3,
Guo, Victor J. W.
2021.
Some variations of a ‘divergent’ Ramanujan-type q-supercongruence.
Journal of Difference Equations and Applications,
Vol. 27,
Issue. 3,
p.
376.
Liu, Yudong
and
Wang, Xiaoxia
2021.
q-analogues of the (G.2) supercongruence of Van Hamme.
Rocky Mountain Journal of Mathematics,
Vol. 51,
Issue. 4,
El Bachraoui, Mohamed
2021.
On supercongruences for truncated sums of squares of basic hypergeometric series.
The Ramanujan Journal,
Vol. 54,
Issue. 2,
p.
415.
Guo, Victor J. W.
and
Lian, Xiuguo
2021.
Some q-congruences on double basic hypergeometric sums.
Journal of Difference Equations and Applications,
Vol. 27,
Issue. 3,
p.
453.
Song, Hanfei
and
Wang, Chun
2021.
Some q-Supercongruences Modulo the Fifth Power of a Cyclotomic Polynomial from Squares of q-Hypergeometric Series.
Results in Mathematics,
Vol. 76,
Issue. 4,
Guo, Victor J. W.
2021.
Proof of a generalization of the (C.2) supercongruence of Van Hamme.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 115,
Issue. 2,
Liu, Yudong
and
Wang, Xiaoxia
2021.
q-Analogues of two Ramanujan-type supercongruences.
Journal of Mathematical Analysis and Applications,
Vol. 502,
Issue. 1,
p.
125238.
Wei, Chuanan
2021.
Some q-supercongruences modulo the fourth power of a cyclotomic polynomial.
Journal of Combinatorial Theory, Series A,
Vol. 182,
Issue. ,
p.
105469.
Jana, Arijit
and
Kalita, Gautam
2021.
On Some Conjectural Supercongruences for Sums Involving Certain Rising Factorials.
Results in Mathematics,
Vol. 76,
Issue. 3,
Guo, Victor J. W.
and
Schlosser, Michael J.
2021.
Some q-supercongruences modulo the square and cube of a cyclotomic polynomial.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 115,
Issue. 3,
 $$\sum\limits_{k = 0}^{((p^r-1)/(2))} {\displaystyle{{4k + 1} \over {{256}^k}}} \left( \matrix{2k \cr k} \right)^4\equiv p^r\quad \left( {\bmod p^{r + 3}} \right),$$
$$\sum\limits_{k = 0}^{((p^r-1)/(2))} {\displaystyle{{4k + 1} \over {{256}^k}}} \left( \matrix{2k \cr k} \right)^4\equiv p^r\quad \left( {\bmod p^{r + 3}} \right),$$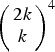 $\left( {\matrix{ {2k} \cr k \cr}} \right)^4$. J. Difference Equ. Appl. 24 (2018), 1375–1383.10.1080/10236198.2018.1485667CrossRefGoogle Scholar
$\left( {\matrix{ {2k} \cr k \cr}} \right)^4$. J. Difference Equ. Appl. 24 (2018), 1375–1383.10.1080/10236198.2018.1485667CrossRefGoogle Scholar



