Article contents
On the L∞-maximization of the solution of Poisson's equation: Brezis–Gallouet–Wainger type inequalities and applications
Published online by Cambridge University Press: 20 February 2020
Abstract
For the solution of the Poisson problem with an L∞ right hand side
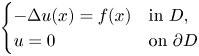 \begin{cases} -\Delta u(x) = f (x) & {\rm in}\ D, \\ u=0 & {\rm on}\ \partial D \end{cases}
\begin{cases} -\Delta u(x) = f (x) & {\rm in}\ D, \\ u=0 & {\rm on}\ \partial D \end{cases}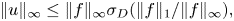 \|u\|_\infty\leq \|f\|_\infty \sigma_D(\|f\|_1/\|f\|_\infty),
\|u\|_\infty\leq \|f\|_\infty \sigma_D(\|f\|_1/\|f\|_\infty),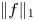 $\|f\|_1$ and
$\|f\|_1$ and 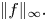 $\|f\|_\infty .$ We also show that
$\|f\|_\infty .$ We also show that
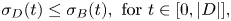 \sigma_D(t)\leq\sigma_B(t),\text{ for }t\in[0,|D|],
\sigma_D(t)\leq\sigma_B(t),\text{ for }t\in[0,|D|],
Keywords
MSC classification
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 151 , Issue 1 , February 2021 , pp. 79 - 92
- Copyright
- Copyright © The Author(s) 2020. Published by The Royal Society of Edinburgh
References
- 1
- Cited by





