Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zhong, Deguang
2021.
ON A QUASI‐ISOMETRY FOR QUASICONFORMAL MAPPING SATISFYING POISSON DIFFERENTIAL INEQUALITY.
Mathematika,
Vol. 67,
Issue. 1,
p.
61.




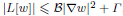 , then
, then 