Hostname: page-component-848d4c4894-8kt4b Total loading time: 0 Render date: 2024-07-08T03:37:03.950Z Has data issue: false hasContentIssue false
  • English
  • Français

Behaviour of solutions to p-Laplacian with Robin boundary conditions as p goes to 1

Part of: Elliptic equations and systems

Published online by Cambridge University Press:  26 January 2023

Francesco Della Pietra ,
Francescantonio Oliva  and
Sergio Segura de León
Francesco Della Pietra
Affiliation:
Dipartimento di Matematica e Applicazioni, Università di Napoli Federico II, Via Cintia, Monte S. Angelo, 80126 Napoli, Italy (f.dellapietra@unina.it, francescantonio.oliva@unina.it)
Francescantonio Oliva
Affiliation:
Dipartimento di Matematica e Applicazioni, Università di Napoli Federico II, Via Cintia, Monte S. Angelo, 80126 Napoli, Italy (f.dellapietra@unina.it, francescantonio.oliva@unina.it)
Sergio Segura de León
Affiliation:
Departament d'Anàlisi Matemàtica, Universitat de València, Dr. Moliner 50, 46100 Burjassot, València, Spain (sergio.segura@uv.es)
Get access Rights & Permissions [Opens in a new window]

Abstract

We study the asymptotic behaviour, as $p\to 1^+$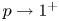, of the solutions of the following inhomogeneous Robin boundary value problem:P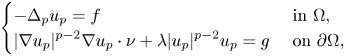

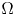\begin{equation*} \begin{cases} \displaystyle -\Delta_p u_p = f & \text{ in }\Omega,\\ \displaystyle |\nabla u_p|^{p-2}\nabla u_p\cdot \nu +\lambda |u_p|^{p-2}u_p = g & \text{ on } \partial\Omega, \end{cases} \end{equation*}
where $\Omega$ is a bounded domain in $\mathbb {R}^{N}$ 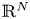with sufficiently smooth boundary, $\nu$ is its unit outward normal vector and $\Delta _p v$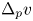 is the $p$-Laplacian operator with $p>1$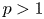. The data $f\in L^{N,\infty }(\Omega )$ 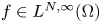(which denotes the Marcinkiewicz space) and $\lambda,\,g$ are bounded functions defined on $\partial \Omega$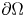 with $\lambda \ge 0$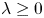. We find the threshold below which the family of $p$–solutions goes to 0 and above which this family blows up. As a second interest we deal with the $1$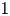-Laplacian problem formally arising by taking $p\to 1^+$ 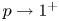in (P).

Keywords

Robin boundary conditionsasymptotic behaviour1-Laplacian

MSC classification

Primary: 35J92: Quasilinear elliptic equations with $p$-Laplacian
Secondary: 35J25: Boundary value problems for second-order elliptic equations 35J70: Degenerate elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 1 , February 2024 , pp. 105 - 130
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alves, C., Ourraoui, A. and Pimenta, M. T. O.. Multiplicity of solutions for a class of quasilinear problems involving the 1-Laplacian operator with critical growth. J. Diff. Equ. 308 (2022), 545–574.CrossRefGoogle Scholar
Alvino, A.. Sulla diseguglianza di Sobolev in spazi di Lorentz. Boll. Un. Mat. Ital. 14 (1977), 3–11.Google Scholar
Andreu, F., Ballester, C., Caselles, V. and Mazón, J. M.. Minimizing total variation flow. Differ. Integral Equ. 14 (2001), 321–360.Google Scholar
Andreu, F., Ballester, C., Caselles, V. and Mazón, J. M.. The Dirichlet problem for the total variation flow. J. Funct. Anal. 180 (2001), 347–403.CrossRefGoogle Scholar
Anzellotti, G.. Pairings between measures and bounded functions and compensated compactness. Ann. Mat. Pura Appl. 135 (1983), 293–318.CrossRefGoogle Scholar
Ambrosio, L., Fusco, N. and Pallara, D., Functions of Bounded Variation and Free Discontinuity Problems, Oxford Mathematical Monographs (2000).CrossRefGoogle Scholar
Bertalmio, M., Caselles, V., Rougé, B. and Solé, A.. TV based image restoration with local constraints, Special issue in honor of the sixtieth birthday of Stanley Osher. J. Sci. Comput. 19 (2003), 95–122.CrossRefGoogle Scholar
Chen, G. Q. and Frid, H.. Divergence-measure fields and hyperbolic conservation laws. Arch. Ration. Mech. Anal. 147 (1999), 89–118.CrossRefGoogle Scholar
Cicalese, M. and Trombetti, C.. Asymptotic behaviour of solutions to $p$-Laplacian equation. Asymptot. Anal. 35 (2003), 27–40.Google Scholar
De Cicco, V., Giachetti, D., Oliva, F. and Petitta, F.. The Dirichlet problem for singular elliptic equations with general nonlinearities. Calc. Var. Partial Differ. Equ. 58 (2019), 129.CrossRefGoogle Scholar
Demengel, F.. On some nonlinear partial differential equations involving the ‘1’–Laplacian and critical Sobolev exponent. ESAIM, Control Optim, Calc. Var. 4 (1999), 667–686.CrossRefGoogle Scholar
Della Pietra, F., Nitsch, C., Oliva, F. and Trombetti, C.. On the behaviour of the first eigenvalue of the $p$-Laplacian with Robin boundary conditions as $p$ goes to $1$. Adv. Calc. Vari.Google Scholar
Hunt, R.. On $L(p,\,q)$ spaces. Enseign Math. (2) 12 (1966), 249–276.Google Scholar
Kawohl, B.. On a family of torsional creep problems. J. Reine Angew. Math. 410 (1990), 1–22.Google Scholar
Kawohl, B., From $p$-Laplace to mean curvature operator and related questions, Progress in Partial Differential Equations: the Metz Surveys, Pitman Res. Notes Math. Ser., Vol. 249 (Longman Sci. Tech., Harlow, 1991), pp. 40–56.Google Scholar
Leray, J. and Lions, J.-L.. Quelques résulatats de Višik sur les problèmes elliptiques nonlinéaires par les méthodes de Minty-Browder. Bull. Soc. Math. France 93 (1965), 97–107.CrossRefGoogle Scholar
Li, Z. and Liu, R.. Existence and concentration behavior of solutions to $1$-Laplace equations on $\mathbb {R}^N$. J. Diff. Eq. 272 (2021), 399–432.CrossRefGoogle Scholar
Littig, S. and Schuricht, F.. Convergence of the eigenvalues of the $p$-Laplace operator as $p$ goes to $1$. Calc. Var. Partial Differ. Equ. 49 (2014), 707–727.CrossRefGoogle Scholar
Mazón, J. M., Rossi, J. D. and Segura de León, S.. The $1$-Laplacian elliptic equation with inhomogeneous Robin boundary conditions. Diff. Int. Eq. 28 (2015), 409–430.Google Scholar
Mercaldo, A., Rossi, J. D., Segura de León, S. and Trombetti, C.. Behaviour of $p$-Laplacian problems with Neumann boundary conditions when $p$ goes to $1$. Communi. Pure Appl. Anal. 12 (2013), 253–267.CrossRefGoogle Scholar
Mercaldo, A., Segura de León, S. and Trombetti, C.. On the behaviour of the solutions to $p$-Laplacian equations as $p$ goes to $1$. Publ. Mat. 52 (2008), 377–411.CrossRefGoogle Scholar
Mercaldo, A., Segura de León, S. and Trombetti, C.. On the solutions to $1$-Laplacian equation with $L^1$ data. J. Funct. Anal. 256 (2009), 2387–2416.CrossRefGoogle Scholar
Modica, L.. Gradient theory of phase transitions with boundary contact energy, Ann. Inst. H. Poincaré 4 (1987), 487–512.CrossRefGoogle Scholar
Molino, A. and Segura de León, S.. Gelfand-type problems involving the $1$-Laplacian operator. Publi. Matemat. 66 (2022), 269–304.CrossRefGoogle Scholar
Moll, S. and Petitta, F.. Large solutions for the elliptic 1-Laplacian with absorption. J. Anal. Math. 125 (2015), 113–138.CrossRefGoogle Scholar
Moser, R.. The inverse mean curvature flow and p-harmonic functions. JEMS 9 (2007), 77–83.CrossRefGoogle Scholar
Nečas, J., Direct methods in the theory of elliptic equations, Transl. from the French. Springer Monographs in Mathematics. (Berlin: Springer, 2012).CrossRefGoogle Scholar
Osher, S. and Sethian, J.. Fronts propagating with curvature-dependent speed: algorithms based on Hamilton-Jacobi formulations. J. Comput. Phys. 79 (1988), 12–49.CrossRefGoogle Scholar
Sapiro, G.. Geometric partial differential equations and image analysis (Cambridge: Cambridge University Press, 2001).CrossRefGoogle Scholar
Scheven, C. and Schmidt, T.. BV supersolutions to equations of 1-Laplace and minimal surface type. J. Differ. Equ. 261 (2016), 1904–1932.CrossRefGoogle Scholar