Hostname: page-component-76fb5796d-r6qrq Total loading time: 0 Render date: 2024-04-26T04:37:06.280Z Has data issue: false hasContentIssue false
  • English
  • Français

Absence of singularities in solutions for the compressible Euler equations with source terms in $\mathbb {R}^d$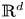

Part of: Equations of mathematical physics and other areas of application

Published online by Cambridge University Press:  26 April 2022

Xinglong Wu
Xinglong Wu*
Affiliation:
Center for Mathematical Sciences, School of Science, Wuhan University of Technology, Wuhan 430070, P. R. China (wxl8758669@aliyun.com)
Get access Rights & Permissions [Opens in a new window]

Abstract

The present article is devoted to the study of global solution and large time behaviour of solution for the isentropic compressible Euler system with source terms in $\mathbb {R}^d$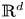, $d\geq 1$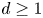, which extends and improves the results obtained by Sideris et al. in ‘T.C. Sideris, B. Thomases, D.H. Wang, Long time behavior of solutions to the 3D compressible Euler equations with damping, Comm. Partial Differential Equations 28 (2003) 795–816’. We first establish the existence and uniqueness of global smooth solution provided the initial datum is sufficiently small, which tells us that the damping terms can prevent the development of singularity in small amplitude. Next, under the additional smallness assumption, the large time behaviour of solution is investigated, we only obtain the algebra decay of solution besides the $L^2$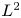-norm of $\nabla u$ 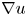is exponential decay.

Keywords

The isentropic compressible Euler equationsthe global smooth solutionthe large time behaviour of solution

MSC classification

Primary: 35Q31: Euler equations
Secondary: 35Q35: PDEs in connection with fluid mechanics
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 3 , June 2023 , pp. 978 - 1001
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bahouri, H., Chemin, J. Y. and Danchin, R.. Fourier Analysis and Nonlinear Partial Differential Equations (Springer-Verlag, Berlin Heidelberg, 2011).CrossRefGoogle Scholar
Chen, G. Q.. Remarks on spherically symmetric solutions to the compressible Euler equations. Proc. Roy. Soc. Edinburgh Sect. A 127 (1997), 243–259.CrossRefGoogle Scholar
Chen, G. Q. and LeFloch, P.. Entropies and flux-splittings for the isentropic Euler equations. Chinese Annals Math. Ser. B 22 (2001), 145–158.10.1142/S0252959901000140CrossRefGoogle Scholar
Chen, G. Q. and Glimm, J.. Global solutions to the compressible Euler equations with geometrical structure. Comm. Math. Phys. 180 (1996), 153–193.CrossRefGoogle Scholar
Chen, G. Q. and Wang, D.. The Cauchy problem for the Euler equations for compressible fluids. Handbook of Mathematical Fluid Dynamics, North–Holland, Amsterdam 1 (2002), 421–543.CrossRefGoogle Scholar
Dafermos, C. M. and Pan, R. H.. Global BV solutions for the p-system with frictional damping. SIAM J. Math. Anal. 41 (2009), 1190–1205.CrossRefGoogle Scholar
Ding, X. X., Chen, G. Q. and Luo, P. Z.. Convergence of the fraction step Lax–Friedrichs scheme and Godunov scheme for the isentropic system of gas dynamics. Comm. Math. Phys. 121 (1989), 63–84.Google Scholar
DiPerna, R.. Convergence of viscosity method for isentropic gas dynamics. Commun. Math. Phys. 91 (1983), 1–30.CrossRefGoogle Scholar
Guo, B. L. and Wu, X. L.. Qualitative analysis of solution for the full compressible Euler equations in $\mathbb {R}^N$. Indiana Univ. Math. J. 1 (2018), 343–373.Google Scholar
Hsiao, L. and Liu, T. P.. Convergence to nonlinear diffusion waves for solutions of a system of hyperbolic conservation laws with damping. Comm. Math. Phys. 143 (1992), 599–605.CrossRefGoogle Scholar
Hsiao, L. and Luo, T.. Nonlinear diffusive phenomena of solutions for the system of compressible adiabatic flow through porous media. J. Differ. Equ. 125 (1996), 329–365.10.1006/jdeq.1996.0034CrossRefGoogle Scholar
Hsiao, L., Luo, T. and Yang, T.. Global BV solutions of compressible Euler equations with spherical symmetry and damping. J. Differ. Equ. 146 (1998), 203–225.CrossRefGoogle Scholar
Huang, F. M., Marcati, P. and Pan, R. H.. Convergence to Barenblatt solution for the compressible Euler equations with damping and vacuum. Arch. Ration. Mech. Anal. 176 (2005), 1–24.10.1007/s00205-004-0349-yCrossRefGoogle Scholar
Huang, F. M. and Pan, R. H.. Asymptotic behavior of the solutions to the damped compressible Euler equations with vacuum. J. Differ. Equ. 220 (2006), 207–233.CrossRefGoogle Scholar
Huang, F. M. and Pan, R. H.. Convergence rate for compressible Euler equations with damping and vacuum. Arch. Ration. Mech. Anal. 166 (2003), 359–376.CrossRefGoogle Scholar
Huang, F. M., Pan, R. H. and Wang, Z.. $L^1$ convergence to the Barenblatt solution for compressible Euler equations with damping. Arch. Ration. Mech. Anal. 200 (2011), 665–689.CrossRefGoogle Scholar
John, F.. Blow-up for quasilinear wave equations in three space dimensions. Comm. Pure Appl. Math. 34 (1981), 29–51.CrossRefGoogle Scholar
Li, T. H. and Wang, D. H.. Blowup phenomena of solutions to the Euler equations for compressible fluid flow. J. Differ. Equ. 221 (2006), 91–101.10.1016/j.jde.2004.12.004CrossRefGoogle Scholar
Lin, L. W.. On the vacuum state for the equations of isentropic gas dynamics. J. Math. Anal. Appl. 121 (1987), 406–425.CrossRefGoogle Scholar
Lions, P. L., Perthame, B. and Souganidis, P.. Existence and stability of entropy solutions for the hyperbolic systems of isentropic gas dynamics in Eulerian and Lagrangian coordinates. Comm. Pure Appl. Math. 49 (1996), 599–638.3.0.CO;2-5>CrossRefGoogle Scholar
Lions, P. L., Perthame, B. and Tadmor, E.. Kinetic formulation of the isentropic gas dynamics and p-systems. Commun. Math. Phys. 163 (1994), 169–172.CrossRefGoogle Scholar
Majda, A.. Compressible Fluid Flow and Systems of Conservation Laws in Several Space Variables, Applied Mathematical Science 53 Springer, New York, 1984.CrossRefGoogle Scholar
Makino, T., Mizohata, K. and Ukai, S.. The global weak solutions of the compressible Euler equation with spherical symmetry. Japan J. Indust. Appl. Math. 9 (1992), 431–449.10.1007/BF03167276CrossRefGoogle Scholar
Makino, T., Mizohata, K. and Ukai, S.. The global weak solutions of the compressible Euler equation with spherical symmetry (II). Japan J. Indust. Appl. Math. 11 (1994), 417–426.10.1007/BF03167230CrossRefGoogle Scholar
Pan, R. H. and Zhao, K.. Initial boundary value problem for compressible Euler equations with damping. Indiana Univ. Math. J. 57 (2008), 2257–2282.10.1512/iumj.2008.57.3366CrossRefGoogle Scholar
Pan, R. H. and Zhao, K.. The 3D compressible Euler equations with damping in a bounded domain. J. Differ. Equ. 246 (2009), 581–596.CrossRefGoogle Scholar
Sideris, T. C.. Formation of singularities in three-dimensional compressible fluids. Commun. Math. Phys. 101 (1985), 475–485.CrossRefGoogle Scholar
Sideris, T. C., Thomases, B. and Wang, D. H.. Long time behavior of solutions to the 3D compressible Euler equations with damping. Comm. Partial Differ. Equ. 28 (2003), 795–816.CrossRefGoogle Scholar
Smoller, J.. Shock Waves and Reaction-Diffusion Equations, 2nd Ed. (Springer-Verlag, New York, 1994).CrossRefGoogle Scholar
Taylor, G. I.. The formation of a blast wave by a very intense explosion. Ministry of Home Security RC 210 (1950), 1941.Google Scholar
Taylor, G. I., The propagation and decay of blast waves, British Civilian Defense Research Committee, 1944.Google Scholar
Tsuge, N.. Global $L^\infty$ solutions of the compressible Euler equations with spherical symmetry. J. Math. Kyoto Univ. 46 (2006), 457–524.Google Scholar
Wang, W. K. and Yang, T.. The pointwise estimates of solutions for Euler equations with damping in multi-dimensions. J. Differ. Equ. 173 (2001), 410–450.CrossRefGoogle Scholar
Wu, X. L.. On the blow-up phenomena of solutions for the full compressible Euler equations in $\mathbb {R}^N$. Nonlinearity 29 (2016), 3837–3856.CrossRefGoogle Scholar