Hostname: page-component-76fb5796d-5g6vh Total loading time: 0 Render date: 2024-04-28T12:21:10.868Z Has data issue: false hasContentIssue false
  • English
  • Français

Cohomology of a real toric variety and shellability of posets arising from a graph

Part of: Topological manifolds Ordered sets Applied homological algebra and category theory

Published online by Cambridge University Press:  03 November 2023

Boram Park  and
Seonjeong Park
Boram Park
Affiliation:
Department of Mathematics, Ajou University, Suwon-si, Gyeonggi-do, Republic of Korea (borampark@ajou.ac.kr)
Seonjeong Park
Affiliation:
Department of Mathematics Education, Jeonju University, Jeonju-si, Jeollabuk-do, Republic of Korea (seonjeongpark@jj.ac.kr)
Get access Rights & Permissions [Opens in a new window]

Abstract

Given a graph G without loops, the pseudograph associahedron PG is a smooth polytope, so there is a projective smooth toric variety XG corresponding to PG. Taking the real locus of XG, we have the projective smooth real toric variety 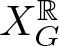$X^{\mathbb{R}}_G$. The integral cohomology groups of 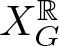$X^{\mathbb{R}}_G$ can be computed by studying the topology of certain posets of even subgraphs of G; such a poset is neither pure nor shellable in general. We completely characterize the graphs whose posets of even subgraphs are always shellable. It follows that we get a family of projective smooth real toric varieties whose integral cohomology groups are torsion-free or have only 2-torsion.

Keywords

shellable posetchain-lexicographic-shellabilityeven subgraphpseudograph associahedronreal smooth toric varietyBetti number

MSC classification

Primary: 06A07: Combinatorics of partially ordered sets 55U10: Simplicial sets and complexes 57N65: Algebraic topology of manifolds 14P25: Topology of real algebraic varieties
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 66 , Issue 4 , November 2023 , pp. 1044 - 1084
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Björner, A., Shellable and Cohen-Macaulay partially ordered sets, Trans. Amer. Math. Soc. 260(1) (1980), 159–183.CrossRefGoogle Scholar
Björner, A. and Wachs, M. L., Bruhat order of Coxeter groups and shellability, Adv. Math. 43(1) (1982), .CrossRefGoogle Scholar
Björner, A. and Wachs, M. L., Shellable nonpure complexes and posets I, Trans. Amer. Math. Soc. 348(4) (1996), 1299–1327.CrossRefGoogle Scholar
Björner, A. and Wachs, M. L., Shellable nonpure complexes and posets II, Trans. Amer. Math. Soc. 349(10) (1997), .CrossRefGoogle Scholar
Cai, L. and Choi, S., Integral cohomology groups of real toric manifolds and small covers, Mosc. Math. J. 21(3) (2021), 467–492.CrossRefGoogle Scholar
Carr, M. and Devadoss, S. L., Coxeter complexes and graph-associahedra, Topology Appl. 153(12) (2006), 2155–2168.CrossRefGoogle Scholar
Carr, M., Devadoss, S. L. and Forcey, S., Pseudograph associahedra, J. Combin. Theory Ser. A. 118(7) (2011), 2035–2055.CrossRefGoogle Scholar
Choi, S., Park, B. and Park, S., Pseudograph and its associated real toric manifold, J. Math. Soc. Japan 69(2) (2017), 693–714.CrossRefGoogle Scholar
Choi, S. and Park, H., A new graph invariant arises in toric topology, J. Math. Soc. Japan 67(2) (2015), 699–720.CrossRefGoogle Scholar
Choi, S. and Park, H., On the cohomology and their torsion of real toric objects, Forum Math. 29(3) (2017), 543–553.CrossRefGoogle Scholar
Cox, D. A., Little, J. B. and Schenck, H. K., Toric varieties. Graduate Studies in Mathematics, Volume 124 (American Mathematical Society, Providence, RI, 2011).Google Scholar
Danilov, V.I., The geometry of toric varieties, Uspekhi Mat. Nauk 33(2) (1978), 85–134.Google Scholar
Jurkiewicz, J., Chow ring of projective nonsingular torus embedding, Colloq. Math. 43(2) (1980), 261–270.CrossRefGoogle Scholar
Jurkiewicz, J., Torus embeddings, polyhedra, k*-actions and homology, (Instytut Matematyczny Polskiej Akademi Nauk, Warszawa, 1985).Google Scholar
Postnikov, A., Permutohedra, Associahedra, and Beyond, Int. Math. Res. 2009(6) (2009), 1026–1106. doi:10.1093/imrn/rnn153CrossRefGoogle Scholar
Schläfli, L., Theorie der vielfachen Kontiutät, written 1850–1852; Zürcher und Furrer, Zürich 1901; Denkschriften der Schweizerischen naturforschenden Gesellschaft, Volume 38 (Birkh user, 1901).CrossRefGoogle Scholar
Stanley, R. P., Supersolvable lattices, Algebra Universalis 2(1) (1972), 197–217.CrossRefGoogle Scholar
Stanley, R. P., Finite lattices and Jordan-Hölder sets, Algebra Universalis 4(1) (1974), 361–371.CrossRefGoogle Scholar
Trevisan, A., Generalized Davis-Januszkiewicz spaces and their applications in algebra and topology, Ph.D. Thesis , Vrije University Amsterdam, 2012.Google Scholar
Wachs, M. L., Poset topology: tools and Applications. Geometric Combinatorics, IAS/PCMI Lecture Note Series (eds. Miller, E., Reiner, V. and Sturmfels, B.), Volume 13, (American Mathematical Society, Providence, 2007).Google Scholar
Walker, J. W., A poset which is shellable but not lexicographically shellable, European J. Combin. 6(3) (1985), 287–288.CrossRefGoogle Scholar
The on-line encyclopedia of integer sequences, available at https://oeis.org/.Google Scholar