Hostname: page-component-77c89778f8-rkxrd Total loading time: 0 Render date: 2024-07-17T03:56:08.106Z Has data issue: false hasContentIssue false
  • English
  • Français

Transversal parameters and tangential flatness

Published online by Cambridge University Press:  24 October 2008

U. Orbanz
U. Orbanz
Affiliation:
Mathematisches Institut, Universität zu Köln, 5000 Köln 41, West Germany
Get access Rights & Permissions [Opens in a new window]

Extract

Let X be an algebraic variety over a field k, z a point of X and 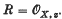Let t = {t1..., ts} be part of a system of parameters of R. Then these parameters are algebraically independent over k, and therefore they define (near z) a projection f: X → As(k) to an s-dimensional affine space over k, sending z to the origin 0∊s(k). In general, the Hilbert function (resp. multiplicity) of will be worse than that of 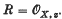, and we will call the system t transversal, if the Hilbert function (resp. multiplicity) of R and R/tR agree. This gives two notions of transversality: one for Hilbert functions (H-transversal), and a weaker one for multiplicities (e-transversal). For s = dim R we recover the notion of a transversal system of parameters introduced by Zariski for studying equisingularity problems (see e.g. [17]). In the above set-up the numerical characters are defined with respect to the maximal ideal of R, but we will consider this problem for arbitrary ideals I using generalized Hilbert functions of type H[x, I, R] (see [7] and [10]).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 98 , Issue 1 , July 1985 , pp. 37 - 49
Copyright
Copyright © Cambridge Philosophical Society 1985

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Böger, E.. Einige Bemerkungen zur Theorie der ganz-algebraischen Abhängigkeit von Idealen. Math. Ann. 185 (1970), 303–308.Google Scholar
[2]Grothe, U., Herrmann, M. and Orbanz, U.. Graded Cohen-Macaulay rings associated to equimultiple ideals. Math. Z. 186 (1984), 531–556.CrossRefGoogle Scholar
[3]Herrmann, M. and Orbanz, U.. Faserdimensionen von Aufblasungen lokaler Ringe und Äquimultiplizität. J. Math. Kyoto Univ. 20 (1980), 651–659.Google Scholar
[4]Herrmann, M. and Orbanz, U.. Between equimultiplicity and normal flatness. In Algebraic Geometry, Proceedings La Rabida, 1981 (ed. Aroca, , Buchweitz, , Giusti, and Merle, ). Lecture Notes in Math. vol. 961 (Springer-Verlag, 1982), 200–232.Google Scholar
[5]Herrmann, M. and Orbanz, U.. On equimultiplicity. Math. Proc. Cambridge Philos. Soc. 91 (1982), 207–213.CrossRefGoogle Scholar
[6]Herrmann, M. and Orbanz, U.. Two notes on flatness. Manuscripta Math. 40 (1982), 109–133.CrossRefGoogle Scholar
[7]Herrmann, M., Schmidt, R. and Vogel, W.. Theorie der normalen Flachheit, Teubner Texte zur Mathematik (Leipzig, 1977).Google Scholar
[8]Lipman, J.. Equimultiplicity, reduction and blowing up. In Commutative Algebra: Analytic Methods (ed. Draper, R.), Lecture Notes in Pure and Appl. vol. 68, (Marcel Dekker, 1981).Google Scholar
[9]Northcott, D. G., Lessons on Rings, Modules, and Multiplicities (Cambridge University Press, 1968).CrossRefGoogle Scholar
[10]Orbanz, U.. Multiplicities and Hilbert functions under blowing up. Manuscripta Math. 36(1981), 179–186.CrossRefGoogle Scholar
[11]Ratliff, L. J.. On quasi-unmixed local domains, the altitude formula, and the chain condition for prime ideals: II. Amer. J. Math. 92 (1970), 99–144.CrossRefGoogle Scholar
[12]Rees, D.. r-transforms of local rings and a theorem on the multiplicities of ideals. Proc. Cambridge Philos. Soc. 57 (1961), 8–17.CrossRefGoogle Scholar
[13]Robbiano, L.. On normal flatness and related topics. In Commutative Algebra, Proc. of the Trento Conf. (ed. Greco, S. and Valla, G.). Lecture Notes in Pure and Appl. Math. vol. 84 (Marcel Dekker, 1983).Google Scholar
[14]Singh, B.. Effect of a permissible blow-up on the local Hilbert functions. Inventiones Math. 26 (1974), 201–212.CrossRefGoogle Scholar
[15]Singh, B.. A numerical criterion for the permissibility of a blowing-up. Compositio Math. 33 (1976), 15–28.Google Scholar
[16]Valabrega, P. and Valla, G.. Form rings and regular sequences. Nagoya Math. J. 72 (1978), 93–101.CrossRefGoogle Scholar
[17]Zariski, O.. Studies in equisingularity: II. Amer. J. Math. 87 (1965), 972–1006.CrossRefGoogle Scholar