Hostname: page-component-8448b6f56d-wq2xx Total loading time: 0 Render date: 2024-04-23T19:08:11.368Z Has data issue: false hasContentIssue false
  • English
  • Français

Local rigidity of complex hyperbolic lattices in semisimple Lie groups

Published online by Cambridge University Press:  29 June 2017

INKANG KIM  and
GENKAI ZHANG
INKANG KIM
Affiliation:
School of Mathematics, KIAS, Hoegiro 85, Dongdaemun-gu, Seoul, 130-722, Korea. e-mail: inkang@kias.re.kr
GENKAI ZHANG
Affiliation:
Mathematical Sciences, Chalmers University of Technology, Mathematical Sciences, Göteborg University, SE-412 96 Göteborg, Sweden. e-mail: genkai@chalmers.se
Get access Rights & Permissions [Opens in a new window]

Abstract

We show the local rigidity of complex hyperbolic lattices in classical Hermitian semisimple Lie groups, SU(p, q), Sp(2n + 2, 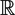$\mathbb{R}$), SO*(2n + 2), SO(2n, 2). This reproves or generalises some results in [2, 9, 11, 15].

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 165 , Issue 1 , July 2018 , pp. 179 - 191
Copyright
Copyright © Cambridge Philosophical Society 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Eastwood, M. and Wolf, J. Branching of representations to symmetric subgroups. Münster J. Math. 4 (2011), 1–27.Google Scholar
[2] Goldman, W. and Millson, J. Local rigidity of discrete groups acting on complex hyperbolic space. Inv. Math. 88 (1987), 495–520.Google Scholar
[3] Helgason, S. Differential Geometry, Lie groups and Symmetric spaces (Academic press, New York, 1978).Google Scholar
[4] Howe, R. θ-series and invariant theory. Automorphic forms, representations and L-functions (Proc. Sympos. Pure Math., Oregon State Univ., Corvallis, Ore., 1977), Part 1, pp. 275–285, Proc. Sympos. Pure Math., XXXIII (Amer. Math. Soc., Providence, R.I., 1979).Google Scholar
[5] Humphreys, J. Introduction to Lie Algebras and Representation Theory (Springer-Verlag, New York, 1972).CrossRefGoogle Scholar
[6] Ihara, S. Holomorphic embeddings of symmetric domains. J. Math. Soc. Japan 19, no.3 (1967).Google Scholar
[7] Johnson, K. D. Composition series and intertwining operators for the spherical principal series. II. Trans. Amer. Math. Soc. 215 (1976), 269–283.Google Scholar
[8] Kim, I. and Pansu, P. Local Rigidity in quaternionic hyperbolic space. J. European Math Society 11 (2009), no 6, 1141–1164.Google Scholar
[9] Kim, I., Klingler, B. and Pansu, P. Local quaternionic rigidity for complex hyperbolic lattices. J. Inst. Math. Jussieu 11 (2012), no 1, 133–159.Google Scholar
[10] Kim, I. and Zhang, G. Eichler–Shimura isomorphism for complex hyperbolic lattices, submitted.Google Scholar
[11] Klingler, B. Local rigidity for complex hyperbolic lattices and Hodge theory. Invent. Math. 184 (2011), no.3, 455–498.CrossRefGoogle Scholar
[12] Koziarz, V. and Maubon, J. Maximal representations of uniform complex hyperbolic lattices, preprint.Google Scholar
[13] Matsushima, Y. and Murakami, S. On vector bundle valued harmonic forms and automorphic forms on symmetric Riemannian manifolds. Ann. Math. 78 (1963), 329–416.Google Scholar
[14] Matsushima, Y. and Murakami, S. On certain cohomology groups attached to Hermitian symmetric spaces. Osaka J. Math. 2 (1965), 1–35.Google Scholar
[15] Pozzetti, M. B. Maximal representations of complex hyperbolic lattices in SU(m,n). Geom. Funct. Anal. 25 (2015), 1290–1332.Google Scholar
[16] Raghunathan, M. S. On the first cohomology of discrete subgroups of semisimple Lie groups. Amer. J. Math. 87 (1965), 103–139.Google Scholar
[17] Raghunathan, M. S. Discrete subgroups of Lie groups (Springer-Verlag, Berlin, Heidelberg, New York, 1972).CrossRefGoogle Scholar
[18] Satake, I. Holomorphic embeddings of symmetric domains into a Siegel domains. Amer. J. Math. 87, no.2 (1965).Google Scholar
[19] Weil, A. Discrete subgroups of Lie groups, II. Ann. of Math 75 (1962), 97–123.Google Scholar
[20] Zucker, S. Locally homogeneous variations of Hodge structure. Enseign. Math. (2) 27 (1982), 243–276.Google Scholar