Hostname: page-component-76dd75c94c-ccc76 Total loading time: 0 Render date: 2024-04-30T09:56:10.827Z Has data issue: false hasContentIssue false
  • English
  • Français

Nonvanishing of twists of 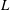$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}L$-functions attached to Hilbert modular forms

Part of: Discontinuous groups and automorphic forms Zeta and $L$-functions: analytic theory

Published online by Cambridge University Press:  01 August 2014

Nathan C. Ryan ,
Gonzalo Tornaría  and
John Voight
Nathan C. Ryan
Affiliation:
Department of Mathematics, Bucknell University, Lewisburg, PA 17837, USA email nathan.c.ryan@gmail.com
Gonzalo Tornaría
Affiliation:
Centro de Matemática, Universidad de la República, 11400 Montevideo, Uruguay email tornaria@cmat.edu.uy
John Voight
Affiliation:
Department of Mathematics , Dartmouth College , 6188 Kemeny Hall , Hanover, NH 03755 , USA email jvoight@gmail.com

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We describe algorithms for computing central values of twists of 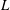$L$-functions associated to Hilbert modular forms, carry out such computations for a number of examples, and compare the results of these computations to some heuristics and predictions from random matrix theory.

MSC classification

Secondary: 11F41: Automorphic forms on $(2)$; Hilbert and Hilbert-Siegel modular groups and their modular and automorphic forms; Hilbert modular surfaces 11M50: Relations with random matrices 11F67: Special values of automorphic $L$-series, periods of modular forms, cohomology, modular symbols
Type
Research Article
Information
LMS Journal of Computation and Mathematics , Volume 17 , Special Issue A: Algorithmic Number Theory Symposium XI , 2014 , pp. 330 - 348
Copyright
© The Author(s) 2014 

References

Baruch, E. M. and Mao, Z., ‘Central value of automorphic L-functions’, Geom. Funct. Anal. 17 (2007) no. 2, 333–384.CrossRefGoogle Scholar
Bosma, W., Cannon, J. and Playoust, C., ‘The Magma algebra system. I. The user language’, J. Symbolic Comput. 24 (1997) no. 3–4, 235–265; Computational algebra and number theory (London, 1993).CrossRefGoogle Scholar
Böcherer, S. and Schulze-Pillot, R., ‘On a theorem of Waldspurger and on Eisenstein series of Klingen type’, Math. Ann. 288 (1990) no. 3, 361–388.CrossRefGoogle Scholar
Conrey, J. B., Keating, J. P., Rubinstein, M. O. and Snaith., N. C., ‘On the frequency of vanishing of quadratic twists of modular L-functions’, Number theory for the millennium, I (Urbana, IL, 2000) (A K Peters, Natick, MA, 2002) 301–315.Google Scholar
Conrey, J. B., Keating, J. P., Rubinstein, M. O. and Snaith, N. C., ‘Random matrix theory and the Fourier coefficients of half-integral-weight forms’, Experiment Math. 15 (2006) no. 1, 67–82.CrossRefGoogle Scholar
David, C., Fearnley, J. and Kisilevsky, H., ‘Vanishing of L-functions of elliptic curves over number fields’, Ranks of elliptic curves and random matrix theory, London Mathematical Society Lecture Note Series 341 (Cambridge University Press, Cambridge, 2007) 247–259.Google Scholar
Delaunay, C. and Watkins, M., ‘The powers of logarithm for quadratic twists’, Ranks of elliptic curves and random matrix theory, London Mathematical Society Lecture Note Series 341 (Cambridge University Press, Cambridge, 2007) 189–193.CrossRefGoogle Scholar
Dembélé, L., ‘Quaternionic Manin symbols, Brandt matrices, and Hilbert modular forms’, Math. Comp. 76 (2007) no. 258, 1039–1057.Google Scholar
Démbéle, L., Donnelly, S. and Voight, J., LMFDB: ${L}$-function and modular form database, 2013,http://www.lmfdb.org.Google Scholar
Dembélé, L. and Voight, J., ‘Explicit methods for Hilbert modular forms’, Elliptic curves, Hilbert modular forms and Galois deformations (Birkhäuser, Basel, 2013) 135–198.CrossRefGoogle Scholar
Diaz y Diaz, F. and Friedman, E., ‘Colmez cones for fundamental units of totally real cubic fields’, J. Number Theory 132 (2012) no. 8, 1653–1663.CrossRefGoogle Scholar
Fincke, U. and Pohst., M., ‘Improved methods for calculating vectors of short length in a lattice, including a complexity analysis’, Math. Comp. 44 (1985) no. 170, 463–471.CrossRefGoogle Scholar
Freitag, E., Hilbert modular forms (Springer, Berlin, 1990).CrossRefGoogle Scholar
Goren, E. Z., Lectures on Hilbert modular varieties and modular forms, CRM Monograph Series 14 (American Mathematical Society, Providence, RI, 2002) With the assistance of Marc-Hubert Nicole.Google Scholar
Gross, B. H., ‘Heights and the special values of L-series’, Number theory (Montreal, Que., 1985), CMS Conference Proceedings 7 (American Mathematical Society, Providence, RI, 1987) 115–187.Google Scholar
Halbritter, U. and Pohst, M. E., ‘On lattice bases with special properties’, J. Théor. Nombres Bordeaux 12 (2000) no. 2, 437–453; Colloque International de Théorie des Nombres (Talence, 1999).CrossRefGoogle Scholar
Hart, W. B., Tornaría, G. and Watkins, M., ‘Congruent number theta coefficients to 1012’, Algorithmic number theory (Springer, 2010) 186–200.CrossRefGoogle Scholar
Hiraga, K. and Ikeda, T., ‘On the Kohnen plus space for Hilbert modular forms of half-integral weight I’, Compos. Math. 149 (2013) no. 12, 1963–2010.CrossRefGoogle Scholar
Keating, J. P. and Snaith, N. C., ‘Random matrix theory and L-functions at s = 1∕2’, Comm. Math. Phys. 214 (2000) no. 1, 91–110.CrossRefGoogle Scholar
Keating, J. P. and Snaith, N. C., ‘Random matrix theory and ζ (1∕2 + i t)’, Comm. Math. Phys. 214 (2000) no. 1, 57–89.CrossRefGoogle Scholar
Kirschmer, M. and Voight, J., ‘Algorithmic enumeration of ideal classes for quaternion orders’, SIAM J. Comput. 39 (2010) no. 5, 1714–1747.CrossRefGoogle Scholar
Kirschmer, M. and Voight, J., ‘Corrigendum: Algorithmic enumeration of ideal classes for quaternion orders’, SIAM J. Comput. 41 (2012) no. 3, 714; MR 2592031.CrossRefGoogle Scholar
Lenstra, A. K., Lenstra, H. W. Jr. and Lovász, L., ‘Factoring polynomials with rational coefficients’, Math. Ann. 261 (1982) no. 4, 515–534.CrossRefGoogle Scholar
Mao, Z., Rodriguez-Villegas, F. and Tornaría, G., ‘Computation of central value of quadratic twists of modular L-functions’, Ranks of elliptic curves and random matrix theory, London Mathematical Society Lecture Note Series 341 (Cambridge University Press, Cambridge, 2007) 273–288.CrossRefGoogle Scholar
Neukirch, J., Algebraic number theory, Grundlehren der Mathematischen Wissenschaften (Fundamental Principles of Mathematical Sciences) 322 (Springer, Berlin, 1999) Translated from the 1992 German original and with a note by Norbert Schappacher, with a foreword by G. Harder.Google Scholar
Okazaki, R., ‘On an effective determination of a Shintani’s decomposition of the cone R+n’, J. Math. Kyoto Univ. 33 (1993) no. 4, 1057–1070.Google Scholar
Pacetti, A. and Tornaría, G., ‘Examples of the Shimura correspondence for level p 2 and real quadratic twists’, Ranks of elliptic curves and random matrix theory, London Mathematical Society Lecture Note Series 341 (Cambridge University Press, Cambridge, 2007) 289–314.CrossRefGoogle Scholar
Pacetti, A. and Tornaría, G., ‘Shimura correspondence for level p 2 and the central values of L-series’, J. Number Theory 124 (2007) no. 2, 396–414.Google Scholar
Pacetti, A. and Tornaría, G., ‘Computing central values of twisted L-series: the case of composite levels’, Experiment. Math. 17 (2008) no. 4, 459–471.CrossRefGoogle Scholar
Rosson, H. and Tornaría, G., ‘Central values of quadratic twists for a modular form of weight 4’, Ranks of elliptic curves and random matrix theory, London Mathematical Society Lecture Note Series 341 (Cambridge University Press, Cambridge, 2007) 315–321.CrossRefGoogle Scholar
Rubinstein, M. O., lcalc: The L-function calculator, a C++ class library and command line program, 2008, http://www.math.uwaterloo.ca/∼mrubinst.Google Scholar
Shimura, G., ‘The special values of the zeta functions associated with Hilbert modular forms’, Duke Math. J. 45 (1978) no. 3, 637–679.Google Scholar
Shimura, G., ‘On Eisenstein series of half-integral weight’, Duke Math. J. 52 (1985) no. 2, 281–314.CrossRefGoogle Scholar
Shimura, G., ‘On Hilbert modular forms of half-integral weight’, Duke Math. J. 55 (1987) no. 4, 765–838.CrossRefGoogle Scholar
Shimura, G., ‘On the Fourier coefficients of Hilbert modular forms of half-integral weight’, Duke Math. J. 71 (1993) no. 2, 501–557.CrossRefGoogle Scholar
Shimura, G., ‘On the transformation formulas of theta series’, Amer. J. Math. 115 (1993) no. 5, 1011–1052.CrossRefGoogle Scholar
Shintani, T., ‘On evaluation of zeta functions of totally real algebraic number fields at non-positive integers’, J. Fac. Sci. Univ. Tokyo Sect. IA Math. 23 (1976) no. 2, 393–417.Google Scholar
Sirolli, N., Preimages for the Shimura map on Hilbert modular forms, Preprint, 2012, arXiv:1208.4011 [math.NT].Google Scholar
Socrates, J. and Whitehouse, D., ‘Unramified Hilbert modular forms, with examples relating to elliptic curves’, Pacific J. Math. 219 (2005) no. 2, 333–364.CrossRefGoogle Scholar
Thomas, E. and Vasquez, A. T., ‘On the resolution of cusp singularities and the Shintani decomposition in totally real cubic number fields’, Math. Ann. 247 (1980) no. 1, 1–20.CrossRefGoogle Scholar
van der Geer, G., Hilbert modular surfaces, Ergebnisse der Mathematik und ihrer Grenzgebiete (3) [Results in Mathematics and Related Areas (3)] 16 (Springer, Berlin, 1988).CrossRefGoogle Scholar
Vignéras, M.-F., ‘Arithmétique des algèbres de quaternions’, Lecture Notes in Mathematics 800 (Springer, Berlin, 1980).Google Scholar
Waldspurger, J.-L., ‘Sur les coefficients de Fourier des formes modulaires de poids demi-entier’, J. Math. Pures Appl. (9) 60 (1981) no. 4, 375–484.Google Scholar
Watkins, M., ‘On elliptic curves and random matrix theory’, J. Théor. Nombres Bordeaux 20 (2008) no. 3, 829–845.CrossRefGoogle Scholar
Watkins, M., ‘Some heuristics about elliptic curves’, Experiment Math. 17 (2008) no. 1, 105–125.CrossRefGoogle Scholar
Xue, H., ‘Central values of L-functions and half-integral weight forms’, Proc. Amer. Math. Soc. 139 (2011) no. 1, 21–30.CrossRefGoogle Scholar