No CrossRef data available.
Article contents
DERIVED NON-ARCHIMEDEAN ANALYTIC HILBERT SPACE
Published online by Cambridge University Press: 26 August 2020
Abstract
In this short paper, we combine the representability theorem introduced in [Porta and Yu, Representability theorem in derived analytic geometry, preprint, 2017, arXiv:1704.01683; Porta and Yu, Derived Hom spaces in rigid analytic geometry, preprint, 2018, arXiv:1801.07730] with the theory of derived formal models introduced in [António, 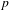 $p$-adic derived formal geometry and derived Raynaud localization theorem, preprint, 2018, arXiv:1805.03302] to prove the existence representability of the derived Hilbert space
$p$-adic derived formal geometry and derived Raynaud localization theorem, preprint, 2018, arXiv:1805.03302] to prove the existence representability of the derived Hilbert space 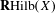 $\mathbf{R}\text{Hilb}(X)$ for a separated
$\mathbf{R}\text{Hilb}(X)$ for a separated 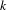 $k$-analytic space
$k$-analytic space 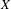 $X$. Such representability results rely on a localization theorem stating that if
$X$. Such representability results rely on a localization theorem stating that if 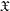 $\mathfrak{X}$ is a quasi-compact and quasi-separated formal scheme, then the
$\mathfrak{X}$ is a quasi-compact and quasi-separated formal scheme, then the 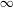 $\infty$-category
$\infty$-category 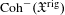 $\text{Coh}^{-}(\mathfrak{X}^{\text{rig}})$ of almost perfect complexes over the generic fiber can be realized as a Verdier quotient of the
$\text{Coh}^{-}(\mathfrak{X}^{\text{rig}})$ of almost perfect complexes over the generic fiber can be realized as a Verdier quotient of the  $\infty$-category
$\infty$-category 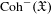 $\text{Coh}^{-}(\mathfrak{X})$. Along the way, we prove several results concerning the
$\text{Coh}^{-}(\mathfrak{X})$. Along the way, we prove several results concerning the 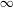 $\infty$-categories of formal models for almost perfect modules on derived
$\infty$-categories of formal models for almost perfect modules on derived 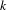 $k$-analytic spaces.
$k$-analytic spaces.
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 21 , Issue 1 , January 2022 , pp. 335 - 366
- Copyright
- © The Author(s) 2020. Published by Cambridge University Press




