A group will be called full if it is the Galois group of an algebraic closure of a field. In this paper we first investigate full Abelian groups and classify them. Then we examine full groups from the point of view of how we can operate on them and still maintain the property of being full. Of course, by the fundamental theorem of the infinite Galois theory, closed subgroups (with standard profinite topology) of full groups are full. In general, products of full groups are notfull (for example, Z2 × Z2 is not full, by a theorem of Artin and Schreier (1927)); however we produce a set of groups which can always be attached as direct factors to full groups and still retain full groups. For the definition and basic properties of profinite groups we refer the reader to Cassels and Frohlich (1967). It has been shown by Leptin (1955) and independently by the author that profinite groups are Galois groups. The author (1971) has shown that if G is profinite, then G = Gdl(k/L) where L may have any desired characteristic and contains all primitive nth roots of unity, for all n. We will denote by 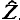p the pro-p-group which is the inverse limit of cyclic p-groups. (This is the group of p-adic integers).