Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Carlucci, Lorenzo
and
Zdanowski, Konrad
2012.
How the World Computes.
Vol. 7318,
Issue. ,
p.
89.
Flumini, Dandolo
and
Sato, Kentaro
2014.
From hierarchies to well-foundedness.
Archive for Mathematical Logic,
Vol. 53,
Issue. 7-8,
p.
855.
Rathjen, Michael
and
Vizcaíno, Pedro Francisco Valencia
2015.
Gentzen's Centenary.
p.
533.
Arai, Toshiyasu
2018.
Derivatives of normal functions and
$$\omega $$
ω
-models.
Archive for Mathematical Logic,
Vol. 57,
Issue. 5-6,
p.
649.
Freund, Anton
2019.
Π11-comprehension as a well-ordering principle.
Advances in Mathematics,
Vol. 355,
Issue. ,
p.
106767.
Arai, Toshiyasu
2020.
Proof-theoretic strengths of the well-ordering principles.
Archive for Mathematical Logic,
Vol. 59,
Issue. 3-4,
p.
257.
Freund, Anton
2020.
A note on ordinal exponentiation and derivatives of normal functions.
Mathematical Logic Quarterly,
Vol. 66,
Issue. 3,
p.
326.
Melnikov, Alexander G.
Selivanov, Victor L.
and
Yamaleev, Mars M.
2020.
Turing reducibility in the fine hierarchy.
Annals of Pure and Applied Logic,
Vol. 171,
Issue. 7,
p.
102766.
Marcone, Alberto
2020.
Well-Quasi Orders in Computation, Logic, Language and Reasoning.
Vol. 53,
Issue. ,
p.
189.
Arai, Toshiyasu
2020.
Ordinal Analysis with an Introduction to Proof Theory.
p.
175.
Freund, Anton
2020.
Computable aspects of the Bachmann–Howard principle.
Journal of Mathematical Logic,
Vol. 20,
Issue. 02,
p.
2050006.
Rathjen, Michael
and
Thomson, Ian Alexander
2020.
The Legacy of Kurt Schütte.
p.
171.
Freund, Anton
and
Rathjen, Michael
2021.
Derivatives of normal functions in reverse mathematics.
Annals of Pure and Applied Logic,
Vol. 172,
Issue. 2,
p.
102890.
Rathjen, Michael
2022.
Axiomatic Thinking II.
p.
89.
Freund, Anton
and
Manca, Davide
2023.
Normal functions and maximal order types.
Journal of Logic and Computation,
Freund, Anton
and
Rathjen, Michael
2023.
Well ordering principles for iterated $$\Pi ^1_1$$-comprehension.
Selecta Mathematica,
Vol. 29,
Issue. 5,
Aguilera, J. P.
and
Pakhomov, F.
2023.
The Π21$\Pi ^1_2$ consequences of a theory.
Journal of the London Mathematical Society,
Vol. 107,
Issue. 3,
p.
1045.
Friedman, Harvey M.
and
Weiermann, Andreas
2023.
Some independence results related to finite trees.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2248,
Freund, Anton
2023.
On the logical strength of the better quasi order with three elements.
Transactions of the American Mathematical Society,
FREUND, ANTON
and
MANCA, DAVIDE
2023.
WEAK WELL ORDERS AND FRAÏSSÉ’S CONJECTURE.
The Journal of Symbolic Logic,
p.
1.
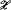 is a well-ordering, then so is
is a well-ordering, then so is 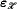 ”, and (2) “If
”, and (2) “If 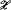 is a well-ordering, then so is φ(α,
is a well-ordering, then so is φ(α, 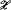 )”, where ∝ is a fixed computable ordinal and φ represents the two-placed Veblen function. For the former statement, we show that ω iterations of the Turing jump are necessary in the proof and that the statement is equivalent to
)”, where ∝ is a fixed computable ordinal and φ represents the two-placed Veblen function. For the former statement, we show that ω iterations of the Turing jump are necessary in the proof and that the statement is equivalent to  over RCA0. To prove the latter statement we need to use ωα iterations of the Turing jump, and we show that the statement is equivalent to
over RCA0. To prove the latter statement we need to use ωα iterations of the Turing jump, and we show that the statement is equivalent to 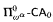 . Our proofs are purely computability-theoretic. We also give a new proof of a result of Friedman: the statement “if
. Our proofs are purely computability-theoretic. We also give a new proof of a result of Friedman: the statement “if 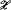 is a well-ordering, then so is φ(
is a well-ordering, then so is φ(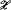 , 0)” is equivalent to ATR0 over RCA0.
, 0)” is equivalent to ATR0 over RCA0.



