Hostname: page-component-76fb5796d-wq484 Total loading time: 0 Render date: 2024-04-26T03:59:21.552Z Has data issue: false hasContentIssue false
  • English
  • Français

Post completeness in modal logic

Published online by Cambridge University Press:  12 March 2014

Krister Segerberg
Krister Segerberg*
Affiliation:
University of Pittsburgh, Pittsburgh, Pennsylvania 15213
Get access Rights & Permissions [Opens in a new window]

Extract

Let ⊥, →, and □ be primitive, and let us have a countable supply of propositional letters. By a (modal) logic we understand a proper subset of the set of all formulas containing every tautology and being closed under modus ponens and substitution. A logic is regular if it contains every instance of □A ∧ □B ↔ □(A ∧ B) and is closed under the rule

A regular logic is normal if it contains □⊤. The smallest regular logic we denote by C (the same as Lemmon's C2), the smallest normal one by K. If L and L' are logics and L ⊆ L′, then L is a sublogic of L', and L' is an extension of L; properly so if L ≠ L'. A logic is quasi-regular (respectively, quasi-normal) if it is an extension of C (respectively, K).

A logic is Post complete if it has no proper extension. The Post number, denoted by p(L), is the number of Post complete extensions of L. Thanks to Lindenbaum, we know that

There is an obvious upper bound, too:

Furthermore,

.

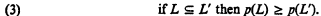Type
Research Article
Information
The Journal of Symbolic Logic , Volume 37 , Issue 4 , December 1972 , pp. 711 - 715
Copyright
Copyright © Association for Symbolic Logic 1972

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Halldén, Sören, Results concerning the decision problem of Lewis's calculi S3 and S6, this Journal, vol. 14 (1950), pp. 230–236.Google Scholar
[2]McCall, Storks and Nat, Arnold Vander, The system S9. Philosophical logic (Davis, J. W., Hockney, D. J., and Wilson, W. K., Editors), Reidel, Dordrecht, 1969, pp. 194–214.CrossRefGoogle Scholar
[3]McKinsey, J. C. C., On the number of complete extensions of the Lewis systems of sentential calculus, this Journal, vol. 9 (1944), pp. 42–45.Google Scholar
[4]Makinson, David, Some embedding theorems for modal logic, Notre Dame Journal of Formal Logic, vol. 12 (1971), pp. 252–254.CrossRefGoogle Scholar
[5]Segerberg, Krister, An essay in classical modal logic, Philosophical Society and Department of Philosophy, University of Uppsala, Uppsala, no. 13, 1971.Google Scholar
[6]Segerberg, Krister, On Post completeness in modal logic, this Journal, vol. 37 (1972). Abstract.Google Scholar
[7]Shukla, Anjan, The existence postulate and non-regular systems of modal logic, Notre Dame Journal of Formal Logic, vol. 13 (1972), pp. 369–378.CrossRefGoogle Scholar
[8]Sobociński, Bolesław, A note on the regular and irregular modal systems of Lewis, Notre Dame Journal of Formal Logic, vol. 3 (1962), pp. 109–113.CrossRefGoogle Scholar
[9]Tarski, A., Logic, semantics, metamathematics. Papers from 1923 to 1938 (translated by Woodger, J. H.), Clarendon Press, Oxford, 1956.Google Scholar
[10]Thomason, S. K., Semantic analysis of tense logics, this Journal, vol. 37 (1972), pp. 150–158.Google Scholar