Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Minami, Hiroaki
2010.
Around splitting and reaping for partitions of ω.
Archive for Mathematical Logic,
Vol. 49,
Issue. 4,
p.
501.
Hrušák, M.
Martínez-Ranero, C. A.
Ramos-García, U. A.
and
Téllez-Nieto, O. A.
2013.
Intersection numbers of families of ideals.
Archive for Mathematical Logic,
Vol. 52,
Issue. 3-4,
p.
403.
Hrušák, Michael
Wohofsky, Wolfgang
and
Zindulka, Ondřej
2016.
Strong measure zero in separable metric spaces and Polish groups.
Archive for Mathematical Logic,
Vol. 55,
Issue. 1-2,
p.
105.
Hrušák, M.
Meza-Alcántara, D.
Thümmel, E.
and
Uzcátegui, C.
2017.
Ramsey type properties of ideals.
Annals of Pure and Applied Logic,
Vol. 168,
Issue. 11,
p.
2022.
Hrušák, Michael
2017.
Katětov order on Borel ideals.
Archive for Mathematical Logic,
Vol. 56,
Issue. 7-8,
p.
831.
Kwela, Adam
2018.
Ideal weak QN-spaces.
Topology and its Applications,
Vol. 240,
Issue. ,
p.
98.
Sakai, Hiroshi
2018.
On Katětov and Katětov–Blass orders on analytic P-ideals and Borel ideals.
Archive for Mathematical Logic,
Vol. 57,
Issue. 3-4,
p.
317.
Cancino-Manríquez, J.
2021.
Every maximal ideal may be Katětov above of all 𝐹_{𝜎} ideals.
Transactions of the American Mathematical Society,
Vol. 375,
Issue. 3,
p.
1861.
Koszmider, Piotr
and
Martínez-Celis, Arturo
2021.
Rosenthal families, pavings, and generic cardinal invariants.
Proceedings of the American Mathematical Society,
Vol. 149,
Issue. 3,
p.
1289.
FARKAS, BARNABÁS
and
ZDOMSKYY, LYUBOMYR
2022.
WAYS OF DESTRUCTION.
The Journal of Symbolic Logic,
Vol. 87,
Issue. 3,
p.
938.
Guzmán, O.
Hrušák, M.
Rodrigues, V.O.
Todorčević, S.
and
Tomita, A.H.
2022.
Maximal almost disjoint families and pseudocompactness of hyperspaces.
Topology and its Applications,
Vol. 305,
Issue. ,
p.
107872.
Leonetti, Paolo
and
Orhan, Cihan
2022.
On some locally convex FK spaces.
Topology and its Applications,
Vol. 322,
Issue. ,
p.
108327.
Bardyla, Serhii
Šupina, Jaroslav
and
Zdomskyy, Lyubomyr
2023.
Ideal approach to convergence in functional spaces.
Transactions of the American Mathematical Society,
Shelah, Saharon
and
Steprāns, Juris
2024.
Some variations on the splitting number.
Annals of Pure and Applied Logic,
Vol. 175,
Issue. 1,
p.
103321.
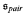 which is a variation of splitting number, pair-reaping number
which is a variation of splitting number, pair-reaping number 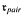 which is a variation of reaping number and cardinal invariants of ideals on ω. We also study cardinal invariants of Fσ ideals and their upper bounds and lower bounds. As an application, we answer a question of S. Solecki by showing that the ideal of finitely chromatic graphs is not locally Katětov-minimal among ideals not satisfying Fatou's lemma.
which is a variation of reaping number and cardinal invariants of ideals on ω. We also study cardinal invariants of Fσ ideals and their upper bounds and lower bounds. As an application, we answer a question of S. Solecki by showing that the ideal of finitely chromatic graphs is not locally Katětov-minimal among ideals not satisfying Fatou's lemma.



