Hostname: page-component-8448b6f56d-t5pn6 Total loading time: 0 Render date: 2024-04-23T17:14:59.525Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE STRENGTH OF TWO RECURRENCE THEOREMS

Published online by Cambridge University Press:  29 September 2016

ADAM R. DAY
ADAM R. DAY*
Affiliation:
SCHOOL OF MATHEMATICS, STATISTICS AND OPERATIONS RESEARCH VICTORIA UNIVERSITY OF WELLINGTON WELLINGTON, NEW ZEALANDE-mail: adam.day@vuw.ac.nz
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper uses the framework of reverse mathematics to investigate the strength of two recurrence theorems of topological dynamics. It establishes that one of these theorems, the existence of an almost periodic point, lies strictly between WKL and ACA (working over RCA0). This is the first example of a theorem with this property. It also shows the existence of an almost periodic point is conservative over RCA0 for 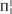${\rm{\Pi }}_1^1$-sentences.

Keywords

Reverse mathematicsdynamical systemsrecurrencecomputability theory
Type
Articles
Information
The Journal of Symbolic Logic , Volume 81 , Issue 4 , December 2016 , pp. 1357 - 1374
Copyright
Copyright © The Association for Symbolic Logic 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Beiglböck, M. and Towsner, H., Transfinite approximation of Hindman’s theorem . Israel Journal of Mathematics, vol. 191 (2012), no. 1, pp. 41–59.Google Scholar
Blass, A. R., Hirst, J. L., and Simpson, S. G., Logical analysis of some theorems of combinatorics and topological dynamics. Logic and combinatorics (Arcata, Calif., 1985), Contemporary Mathematics, vol. 65, American Mathematical Society, Providence, RI, 1987, pp. 125–156.Google Scholar
Friedman, H., Simpson, S. G., and Yu, X., Periodic points and subsystems of second-order arithmetic . Annals of Pure and Applied Logic, vol. 62 (1993), no. 1, pp. 51–64. Logic Colloquium ’89 (Berlin).Google Scholar
Montalbán, A., Open questions in reverse mathematics . Bulletin of Symbolic Logic, vol. 17 (2011), no. 3, pp. 431–454.Google Scholar
Simpson, S. G., Subsystems of Second Order Arithmetic. 2nd edition, Cambridge University Press, New York, 2009.CrossRefGoogle Scholar
Tao, T., Poincaré’s Legacies, Part I. American Mathematical Society, Providence, 2009.Google Scholar
Towsner, H., A combinatorial proof of the dense Hindman’s theorem . Discrete Mathematics, vol. 311 (2011), no. 14, pp. 1380–1384.CrossRefGoogle Scholar
Towsner, H., Hindman’s theorem: an ultrafilter argument in second order arithmetic, this Journal, vol. 76 (2011), no. 1, pp. 353–360.Google Scholar
Towsner, H., A simple proof and some difficult examples for Hindman’s theorem . Notre Dame Journal of Formal Logic, vol. 53 (2012), no. 1, pp. 53–65.Google Scholar