Hostname: page-component-76fb5796d-5g6vh Total loading time: 0 Render date: 2024-04-27T00:32:16.720Z Has data issue: false hasContentIssue false
  • English
  • Français

Transition to chaos through period doublings of a forced oscillating cylinder in steady current

Published online by Cambridge University Press:  21 January 2020

Liang Cheng ,
Xiaoying Ju Open the ORCID record for Xiaoying Ju [Opens in a new window] ,
Feifei Tong Open the ORCID record for Feifei Tong [Opens in a new window]  and
Hongwei An Open the ORCID record for Hongwei An [Opens in a new window]
Liang Cheng
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia DUT-UWA Joint Research Centre, State Key Laboratory of Coastal and Offshore Engineering, Dalian University of Technology, No. 2 Linggong Road, Dalian116024, China
Xiaoying Ju*
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Feifei Tong
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
Hongwei An
Affiliation:
School of Engineering, The University of Western Australia, 35 Stirling Highway, Perth, WA 6009, Australia
*
†Email address for correspondence: xiaoying.ju@research.uwa.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

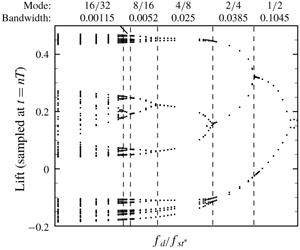
Transition to chaos through a cascade of period doublings of the primary $1/2$ synchronization mode is discovered in steady approaching flow around a forced inline oscillating cylinder near a plane boundary at a Reynolds number $(Re)$ of 175. The transition occurs well within the otherwise synchronized region (known as the Arnold tongue) in the frequency and amplitude space of the oscillating cylinder, creating two parameter strips of desynchronized flows within the Arnold tongue. Five orders of period doublings from mode $1/2$ to mode $16/32$ are revealed by progressively increasing the frequency resolution in the simulation. The ratio of frequency intervals of two successive period-doubling modes asymptotes towards the first Feigenbaum constant, reaching a value of 4.52 at mode of $16/32$. Additional three-dimensional simulations demonstrate the existence of period doubling with a regular spanwise flow structure similar to regular mode B of steady flow around an isolated cylinder. Although transition to chaos through cascades of period doublings is primarily reported for the primary $1/2$ synchronization mode, it is also observed for other synchronization modes $(p/q)$ (Tang et al., J. Fluid Mech., vol. 832, 2017, pp. 146–169), where $p$ and $q$ are integers with a non-reducible $p/q$, such as $2/3$. The physical mechanisms responsible for the present period-doubling bifurcations and transition to chaos through cascades of period doublings are ascribed to the interaction of asymmetric vortex shedding from the cylinder (due to a geometric asymmetry) and the boundary layer developed on the plane boundary, through specifically designed numerical tests.

JFM classification

Nonlinear Dynamical Systems: Bifurcation Vortex Flows: Vortex dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 887 , 25 March 2020 , A5
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Al-Mdallal, Q. M., Lawrence, K. P. & Kocabiyik, S. 2007 Forced streamwise oscillations of a circular cylinder: locked-on modes and resulting fluid forces. J. Fluids Struct. 23, 681–701.CrossRefGoogle Scholar
Blackburn, H. M. & Henderson, R. D. 1999 A study of two-dimensional flow past an oscillating cylinder. J. Fluid Mech. 385, 255–286.CrossRefGoogle Scholar
Blackburn, H. M. & Sheard, G. J. 2010 On quasiperiodic and subharmonic Floquet wake instabilities. Phys. Fluids 22 (3), 031701.CrossRefGoogle Scholar
Cantwell, C., Moxey, D., Comerford, A., Bolis, A., Rocco, G., Mengaldo, G., DE GRAZIA, D., Yakovlev, S., Lombard, J.-E. & Ekelschot, D. 2015 Nektar++: an open-source spectral/hp element framework. Comput. Phys. Commun. 192, 205–219.CrossRefGoogle Scholar
Feigenbaum, M. J.1976 Universality in complex discrete dynamics. LA-6816-PR, LASL Theoretical Division Annual Report, July 1975–September 1976. United States Energy Research and Development Administration.Google Scholar
Griffin, O. M. & Ramberg, S. E. 1976 Vortex shedding from a cylinder vibrating in line with an incident uniform flow. J. Fluid Mech. 75 (2), 257–271.CrossRefGoogle Scholar
Jeong, J. & Hussain, F. 1995 On the identification of a vortex. J. Fluid Mech. 285, 69–94.CrossRefGoogle Scholar
Jiang, H., Cheng, L., Draper, S. & An, H. 2017 Two- and three-dimensional instabilities in the wake of a circular cylinder near a moving wall. J. Fluid Mech. 812, 435–462.CrossRefGoogle Scholar
Jiang, H., Cheng, L., Draper, S., An, H. & Tong, F. 2016 Three-dimensional direct numerical simulation of wake transitions of a circular cylinder. J. Fluid Mech. 801, 353–391.CrossRefGoogle Scholar
Karniadakis, G. E., Israeli, M. & Orszag, S. A. 1991 High-order splitting methods for the incompressible Navier–Stokes equations. J. Comput. Phys. 97, 414–443.CrossRefGoogle Scholar
Konstantinidis, E. & Bouris, D. 2016 Vortex synchronization in the cylinder wake due to harmonic and non-harmonic perturbations. J. Fluid Mech. 804, 248–277.CrossRefGoogle Scholar
Lei, C., Cheng, L., Armfield, S. W. & Kavanagh, K. 2000 Vortex shedding suppression for flow over a circular cylinder near a plane boundary. Ocean Engng 10 (27), 1109–1127.CrossRefGoogle Scholar
Leontini, J. S., Lo Jacono, D. & Thompson, M. C. 2011 A numerical study of an inline oscillating cylinder in a free stream. J. Fluid Mech. 688, 551–568.CrossRefGoogle Scholar
Leontini, J. S., Lo Jacono, D. & Thompson, M. C. 2013 Wake states and frequency selection of a streamwise oscillating cylinder. J. Fluid Mech. 730, 162–192.CrossRefGoogle Scholar
Leontini, J. S., Stewart, B., Thompson, M. & Hourigan, K. 2006 Wake state and energy transitions of an oscillating cylinder at low Reynolds number. Phys. Fluids 18, 067101.CrossRefGoogle Scholar
McGehee, R. P. & Peckham, B. B. 1996 Arnold flames and resonance surface folds. Intl J. Bifurcation Chaos 6 (02), 315–336.CrossRefGoogle Scholar
Newman, D. J. & Karniadakis, G. E. 1997 A direct numerical simulation study of flow past a freely vibrating cable. J. Fluid Mech. 344, 95–136.CrossRefGoogle Scholar
Papaioannou, G. V., Yue, D. K., Triantafyllou, M. S. & Karniadakis, G. E. 2006 Evidence of holes in the Arnold tongues of flow past two oscillating cylinders. Phys. Rev. Lett. 96 (1), 014501.CrossRefGoogle ScholarPubMed
Peckham, B. B. & Kevrekidis, I. G. 2002 Lighting Arnold flames: resonance in doubly forced periodic oscillators. Nonlinearity 15 (2), 405–428.CrossRefGoogle Scholar
Pikovsky, A., Rosenblum, M. & Kurths, J. 2001 Synchronization: A Universal Concept in Nonlinear Sciences. Cambridge University Press.CrossRefGoogle Scholar
Rao, A., Thompson, M. C., Leweke, T. & Hourigan, K. 2013 The flow past a circular cylinder translating at different heights above a wall. J. Fluids Struct. 41, 9–21.CrossRefGoogle Scholar
Rao, A., Thompson, M. C., Leweke, T. & Hourigan, K. 2015 Flow past a rotating cylinder translating at different gap heights along a wall. J. Fluids Struct. 57, 314–330.CrossRefGoogle Scholar
Tang, G., Cheng, L., Tong, F., Lu, L. & Zhao, M. 2017 Modes of synchronisation in the wake of a streamwise oscillatory cylinder. J. Fluid Mech. 832, 146–169.CrossRefGoogle Scholar
Williamson, C. H. K. 1996 Three-dimensional wake transition. J. Fluid Mech. 328, 345–407.CrossRefGoogle Scholar
Xiong, C., Cheng, L., Tong, F. & An, H. 2018 On regime C flow around an oscillating circular cylinder. J. Fluid Mech. 849, 968–1008.CrossRefGoogle Scholar
Xu, S. J., Zhou, Y. & Wang, M. H. 2006 A symmetric binary-vortex street behind a longitudinally oscillating cylinder. J. Fluid Mech. 556, 27–43.CrossRefGoogle Scholar
Zhang, H. Q., Fey, U., Noack, B. R., König, M. & Eckelmann, H. 1995 On the transition of the cylinder wake. Phys. Fluids 7 (4), 779–794.CrossRefGoogle Scholar