Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
De Marinis, Dario
Chibbaro, Sergio
Meldi, Marcello
and
Sagaut, Pierre
2013.
Temperature dynamics in decaying isotropic turbulence with Joule heat production.
Journal of Fluid Mechanics,
Vol. 724,
Issue. ,
p.
425.
Scheel, Janet D
Emran, Mohammad S
and
Schumacher, Jörg
2013.
Resolving the fine-scale structure in turbulent Rayleigh–Bénard convection.
New Journal of Physics,
Vol. 15,
Issue. 11,
p.
113063.
Donzis, Diego A.
Aditya, Konduri
Sreenivasan, K. R.
and
Yeung, P. K.
2014.
The Turbulent Schmidt Number.
Journal of Fluids Engineering,
Vol. 136,
Issue. 6,
Iyer, K. P.
and
Yeung, P. K.
2014.
Structure functions and applicability of Yaglom's relation in passive-scalar turbulent mixing at low Schmidt numbers with uniform mean gradient.
Physics of Fluids,
Vol. 26,
Issue. 8,
Yeung, P. K.
and
Sreenivasan, K. R.
2014.
Direct numerical simulation of turbulent mixing at very low Schmidt number with a uniform mean gradient.
Physics of Fluids,
Vol. 26,
Issue. 1,
Ni, Qionglin
2015.
Compressible turbulent mixing: Effects of Schmidt number.
Physical Review E,
Vol. 91,
Issue. 5,
Hill, Reginald J.
2017.
Spectra of turbulently advected scalars that have small Schmidt number.
Physical Review Fluids,
Vol. 2,
Issue. 9,
Chatterjee, Anando G.
Verma, Mahendra K.
Kumar, Abhishek
Samtaney, Ravi
Hadri, Bilel
and
Khurram, Rooh
2018.
Scaling of a Fast Fourier Transform and a pseudo-spectral fluid solver up to 196608 cores.
Journal of Parallel and Distributed Computing,
Vol. 113,
Issue. ,
p.
77.
Zhai, X. M.
and
Yeung, P. K.
2018.
Evolution of anisotropy in direct numerical simulations of MHD turbulence in a strong magnetic field on elongated periodic domains.
Physical Review Fluids,
Vol. 3,
Issue. 8,
Verma, Mahendra K.
2019.
Asymmetric energy transfers in driven nonequilibrium systems and arrow of time.
The European Physical Journal B,
Vol. 92,
Issue. 9,
Sreenivasan, Katepalli R.
2019.
Turbulent mixing: A perspective.
Proceedings of the National Academy of Sciences,
Vol. 116,
Issue. 37,
p.
18175.
Götzfried, Paul
Emran, Mohammad S.
Villermaux, Emmanuel
and
Schumacher, Jörg
2019.
Comparison of Lagrangian and Eulerian frames of passive scalar turbulent mixing.
Physical Review Fluids,
Vol. 4,
Issue. 4,
Jolly, M. S.
and
Wirosoetisno, D.
2020.
Tracer Turbulence: The Batchelor–Howells–Townsend Spectrum Revisited.
Journal of Mathematical Fluid Mechanics,
Vol. 22,
Issue. 2,
Yasuda, Tatsuya
Gotoh, Toshiyuki
Watanabe, Takeshi
and
Saito, Izumi
2020.
Péclet-number dependence of small-scale anisotropy of passive scalar fluctuations under a uniform mean gradient in isotropic turbulence.
Journal of Fluid Mechanics,
Vol. 898,
Issue. ,
Verma, Manthan
Chatterjee, Soumyadeep
Garg, Gaurav
Sharma, Bharatkumar
Arya, Nishant
Kumar, Shashi
Saxena, Anish
and
Verma, Mahendra
2022.
Scalable Multi-Node Fast Fourier Transform on Gpus.
SSRN Electronic Journal ,
Noble, Christopher D.
Ames, Alex M.
McConnell, Raymond
Oakley, Jason
Rothamer, David A.
and
Bonazza, Riccardo
2023.
Simultaneous measurements of kinetic and scalar energy spectrum time evolution in the Richtmyer–Meshkov instability upon reshock.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
Verma, Manthan
Chatterjee, Soumyadeep
Garg, Gaurav
Sharma, Bharatkumar
Arya, Nishant
Kumar, Sashi
Saxena, Anish
and
Verma, Mahendra K.
2023.
Scalable Multi-node Fast Fourier Transform on GPUs.
SN Computer Science,
Vol. 4,
Issue. 5,
Jolly, M.S.
and
Wirosoetisno, D.
2023.
The Batchelor–Howells–Townsend spectrum: Three-dimensional case.
Physica D: Nonlinear Phenomena,
Vol. 445,
Issue. ,
p.
133615.
Martinez, L. Germes
Duret, B.
Reveillon, J.
and
Demoulin, F.X.
2023.
Vapor mixing in turbulent vaporizing flows.
International Journal of Multiphase Flow,
Vol. 161,
Issue. ,
p.
104388.
Jolly, M S
and
Wirosoetisno, D
2024.
The Batchelor–Howells–Townsend spectrum: large velocity case.
Nonlinearity,
Vol. 37,
Issue. 7,
p.
075025.
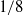 $1/ 8$ to
$1/ 8$ to 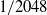 $1/ 2048$ for two values of the microscale Reynolds number,
$1/ 2048$ for two values of the microscale Reynolds number, 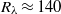 ${R}_{\lambda } \approx 140$ and
${R}_{\lambda } \approx 140$ and 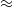 $\approx $240. The simulations show that the passive scalar spectrum assumes a slope of
$\approx $240. The simulations show that the passive scalar spectrum assumes a slope of 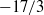 $- 17/ 3$ in a range of scales, as predicted by the theory, when the Schmidt number is small and the Reynolds number is simultaneously large. The observed agreement between theory and simulation in the prefactor in the spectrum is not perfect. We assess the reasons for this discrepancy by a careful examination of the scalar evolution equation in the light of the assumptions of the theory, and conclude that the finite range of scales resolved in simulations is the main reason. Numerical issues specific to the regime of very low Schmidt numbers are also addressed briefly.
$- 17/ 3$ in a range of scales, as predicted by the theory, when the Schmidt number is small and the Reynolds number is simultaneously large. The observed agreement between theory and simulation in the prefactor in the spectrum is not perfect. We assess the reasons for this discrepancy by a careful examination of the scalar evolution equation in the light of the assumptions of the theory, and conclude that the finite range of scales resolved in simulations is the main reason. Numerical issues specific to the regime of very low Schmidt numbers are also addressed briefly.



