Hostname: page-component-77c89778f8-sh8wx Total loading time: 0 Render date: 2024-07-17T01:46:48.681Z Has data issue: false hasContentIssue false
  • English
  • Français

Morphodynamics of active nematic fluid surfaces

Published online by Cambridge University Press:  10 February 2023

Sami C. Al-Izzi Open the ORCID record for Sami C. Al-Izzi [Opens in a new window]  and
Richard G. Morris Open the ORCID record for Richard G. Morris [Opens in a new window]
Sami C. Al-Izzi*
Affiliation:
School of Physics & EMBL Australia Node in Single Molecule Science, School of Medical Sciences, University of New South Wales, Sydney 2052, Australia
Richard G. Morris*
Affiliation:
School of Physics & EMBL Australia Node in Single Molecule Science, School of Medical Sciences, University of New South Wales, Sydney 2052, Australia
*
†Email addresses for correspondence: s.al-izzi@unsw.edu.au, r.g.morris@unsw.edu.au
†Email addresses for correspondence: s.al-izzi@unsw.edu.au, r.g.morris@unsw.edu.au
Get access Rights & Permissions [Opens in a new window]

Abstract

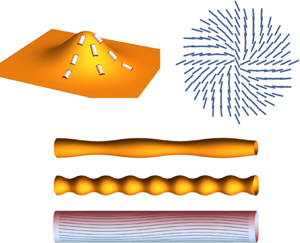
Morphodynamic equations governing the behaviour of active nematic fluids on deformable curved surfaces are constructed in the large deformation limit. Emphasis is placed on the formulation of objective rates that account for normal deformations whilst ensuring that tangential flows are Eulerian, and the use of the surface derivative (rather than the covariant derivative) in the nematic free energy, which elastically couples local order to out-of-plane bending of the surface. Focusing on surface geometry and its dynamical interplay with the hydrodynamics, several illustrative instabilities are then characterised. These include cases where the role of the Scriven–Love number and its nematic analogue are non-negligible, and where the active nematic forcing can be characterised by an analogue of the Föppl–von Kármán number. For the former, flows and changes to the nematic texture are coupled to surface geometry by viscous dissipation. This is shown to result in non-trivial relaxation dynamics for a nematic tube. For the latter, the nematic active forcing couples to the surface bending terms of the nematic free energy, resulting in extensile (active ruffling) and contractile (active pearling) instabilities in the tube shape, as well as active bend instabilities in the nematic texture. In comparison to the flat case, such bend instabilities now have a threshold set by the extrinsic curvature of the tube. Finally, we examine a topological defect located on an almost flat surface, and show that there exists a steady state where a combination of defect elasticity, activity and non-negligible spin connection drive a shape change in the surface.

JFM classification

Biological Fluid Dynamics: Membranes Complex Fluids: Active matter Complex Fluids: Liquid crystals
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 957 , 25 February 2023 , A4
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Al-Izzi, S.C. & Morris, R.G. 2021 Active flows and deformable surfaces in development. Semin. Cell Dev. Biol. 120, 44–52.CrossRefGoogle ScholarPubMed
Al-Izzi, S.C., Sens, P. & Turner, M.S. 2020 a Shear-driven instabilities of membrane tubes and dynamin-induced scission. Phys. Rev. Lett. 125, 018101.CrossRefGoogle ScholarPubMed
Al-Izzi, S.C., Sens, P., Turner, M.S. & Komura, S. 2020 b Dynamics of passive and active membrane tubes. Soft Matt. 16, 9319.CrossRefGoogle ScholarPubMed
Alert, R. 2022 Fingering instability in active nematic droplets. J. Phys. A: Math. Theor. 55, 234009.CrossRefGoogle Scholar
Arroyo, M. & DeSimone, A. 2009 Relaxation dynamics of fluid membranes. Phys. Rev. E 79, 031915.CrossRefGoogle ScholarPubMed
Audoly, B. & Pomeau, Y. 2010 Elasticity and Geometry: From Hair Curls to the Non-linear Response of Shells. Oxford University Press.Google Scholar
Bächer, C., Khoromskaia, D., Salbreux, G. & Gekle, S. 2021 A three-dimensional numerical model of an active cell cortex in the viscous limit. Front. Phys. 9, 753230.CrossRefGoogle Scholar
Barrett, J.W., Garcke, H. & Nürnberg, R. 2016 A stable numerical method for the dynamics of fluidic membranes. Numer. Math. 134, 783–822.CrossRefGoogle ScholarPubMed
Bell, S., Lin, S.-Z., Rupprecht, J.-F. & Prost, J. 2022 Active nematic flows over curved surfaces. Phys. Rev. Lett. 129, 118001.CrossRefGoogle ScholarPubMed
Binysh, J., Kos, Z̆., C̆opar, S., Ravnik, M. & Alexander, G.P. 2020 Three-dimensional active defect loops. Phys. Rev. Lett. 124, 088001.CrossRefGoogle ScholarPubMed
Blanch-Mercader, C., Guillamat, P., Roux, A. & Kruse, K. 2021 a Integer topological defects of cell monolayers: mechanics and flows. Phys. Rev. E 103, 012405.CrossRefGoogle ScholarPubMed
Blanch-Mercader, C., Guillamat, P., Roux, A. & Kruse, K. 2021 b Quantifying material properties of cell monolayers by analyzing integer topological defects. Phys. Rev. Lett. 126, 028101.CrossRefGoogle ScholarPubMed
Boedec, G., Jaeger, M. & Leonetti, M. 2014 Pearling instability of a cylindrical vesicle. J. Fluid Mech. 743, 262–279.CrossRefGoogle Scholar
DeGennes, P.G. & Prost, J. 1993 The Physics of Liquid Crystals. Clarendon Press.Google Scholar
Denniston, C., Orlandini, E. & Yeomans, J.M. 2001 Lattice Boltzmann simulations of liquid crystal hydrodynamics. Phys. Rev. E 63, 056702.CrossRefGoogle ScholarPubMed
Doi, M. 2011 Onsager's variational principle in soft matter. J. Phys.: Condens. Matter 23, 284118.Google ScholarPubMed
Doostmohammadi, A., Ignés-Mullol, J., Yeomans, J.M. & Sagués, F. 2018 Active nematics. Nat. Commun. 9, 3246.CrossRefGoogle ScholarPubMed
Edwards, S.A. & Yeomans, J.M. 2009 Spontaneous flow states in active nematics: a unified picture. Europhys. Lett. 85, 18008.CrossRefGoogle Scholar
Fernández-Nieves, A., Link, D.R., Márquez, M. & Weitz, D.A. 2007 Topological changes in bipolar nematic droplets under flow. Phys. Rev. Lett. 98, 087801.CrossRefGoogle ScholarPubMed
Fournier, J.-B. & Galatola, P. 2007 Critical fluctuations of tense fluid membrane tubules. Phys. Rev. Lett. 98, 018103.CrossRefGoogle ScholarPubMed
Frank, F.C. 1958 I. Liquid crystals. On the theory of liquid crystals. Discuss. Faraday Soc. 25, 19–28.CrossRefGoogle Scholar
Frank, J.R. & Kardar, M. 2008 Defects in nematic membranes can buckle into pseudospheres. Phys. Rev. E 77, 041705.CrossRefGoogle ScholarPubMed
Frankel, T. 2011 The Geometry of Physics: An Introduction. Cambridge University Press.CrossRefGoogle Scholar
Giomi, L., Bowick, M.J., Mishra, P., Sknepnek, R. & Marchetti, M.C. 2014 Defect dynamics in active nematics. Phil. Trans. R. Soc. A 372, 20130365.CrossRefGoogle ScholarPubMed
Gurin, K.L., Lebedev, V.V. & Muratov, A.R. 1996 Dynamic instability of a membrane tube. J. Expl Theor. Phys. 83, 321–326.Google Scholar
Hoffmann, L.A., Carenza, L.N., Eckert, J. & Giomi, L. 2022 Defect-mediated morphogenesis. Sci. Adv. 8, eabk2712.CrossRefGoogle ScholarPubMed
Hu, D., Zhang, P. & Weinan, E. 2007 Continuum theory of a moving membrane. Phys. Rev. E 75, 041605.CrossRefGoogle ScholarPubMed
Jahnke, K., Huth, V., Mersdorf, U., Liu, N. & Göpfrich, K. 2022 Bottom-up assembly of synthetic cells with a DNA cytoskeleton. ACS Nano 16, 7233–7241.CrossRefGoogle ScholarPubMed
Jülicher, F., Grill, S.W. & Salbreux, G. 2018 Hydrodynamic theory of active matter. Rep. Prog. Phys. 81, 076601.CrossRefGoogle ScholarPubMed
Keber, F.C., Loiseau, E., Sanchez, T., DeCamp, S.J., Giomi, L., Bowick, M.J., Marchetti, M.C., Dogic, Z. & Bausch, A.R. 2014 Topology and dynamics of active nematic vesicles. Science 645, 1135–1139.CrossRefGoogle Scholar
Khoromskaia, D. & Alexander, G.P. 2017 Vortex formation and dynamics of defects in active nematic shells. New J. Phys. 19, 103043.CrossRefGoogle Scholar
Khoromskaia, D. & Salbreux, G. 2023 Active morphogenesis of patterned epithelial shells. eLife 12, e75878.CrossRefGoogle ScholarPubMed
Kruse, K., Joanny, J.-F., Jülicher, F., Prost, J. & Sekimoto, K. 2004 Asters, vortices, and rotating spirals in active gels of polar filaments. Phys. Rev. Lett. 92, 078101.CrossRefGoogle ScholarPubMed
Kruse, K., Joanny, J.-F., Jülicher, F., Prost, J. & Sekimoto, K. 2005 Generic theory of active polar gels: a paradigm for cytoskeletal dynamics. Eur. Phys. J. E 16, 5–16.CrossRefGoogle ScholarPubMed
Lee, J.M. 1997 Riemannian Manifolds: an Introduction to Curvature. Graduate Texts in Mathematics, vol. 176. Springer.CrossRefGoogle Scholar
Marchetti, M.C., Joanny, J.-F., Ramaswamy, S., Liverpool, T.B., Prost, J., Rao, M. & Simha, R.A. 2013 Hydrodynamics of soft active matter. Rev. Mod. Phys. 85, 1143–1189.CrossRefGoogle Scholar
Maroudas-Sacks, Y., Garion, L., Shani-Zerbib, L., Livshits, A., Braun, E. & Kinneret, K. 2021 Topological defects in the nematic order of actin fibres as organization centres of hydra morphogenesis. Nat. Phys. 17, 251–259.CrossRefGoogle Scholar
Marsden, J.E. & Hughes, T.J.R. 1994 Mathematical Foundations of Elasticity. Dover Publications.Google Scholar
Metselaar, L., Yeomans, J.M. & Doostmohammadi, A. 2019 Topology and morphology of self-deforming active shells. Phys. Rev. Lett. 123, 208001.CrossRefGoogle ScholarPubMed
Mietke, A., Jülicher, F. & Sbalzarini, I.F. 2019 Self-organized shape dynamics of active surfaces. Proc. Natl Acad. Sci. USA 116, 29–34.CrossRefGoogle ScholarPubMed
Naganathan, S.R., Fürthauer, S., Nishikawa, M., Jülicher, F. & Grill, S.W. 2014 Active torque generation by the actomyosin cell cortex drives left–right symmetry breaking. eLife 3, e04165.CrossRefGoogle ScholarPubMed
Napoli, G. & Vergori, L. 2012 Extrinsic curvature effects on nematic shells. Phys. Rev. Lett. 108, 207803.CrossRefGoogle ScholarPubMed
Napoli, G. & Vergori, L. 2016 Hydrodynamic theory for nematic shells: the interplay among curvature, flow, and alignment. Phys. Rev. E 94, 020701(R).CrossRefGoogle ScholarPubMed
Narsimhan, V., Spann, A.P. & Shaqfeh, E.S.G. 2015 Pearling, wrinkling, and buckling of vesicles in elongational flows. J. Fluid Mech. 777, 1–26.CrossRefGoogle Scholar
Needham, T. 2021 Visual Differential Geometry and Forms: A Mathematical Drama in Five Acts. Princeton University Press.Google Scholar
Nelson, P., Powers, T. & Seifert, U. 1995 Dynamical theory of the pearling instability in cylindrical vesicles. Phys. Rev. Lett. 74 (17), 3384.CrossRefGoogle ScholarPubMed
Nestler, M. & Voigt, A. 2022 Active nematodynamics on curved surfaces – the influence of geometric forces on motion patterns of topological defects. Commun. Comput. Phys. 31, 947–965.CrossRefGoogle Scholar
Nitschke, I. & Voigt, A. 2022 Observer-invariant time derivatives on moving surfaces. J. Geom. Phys. 173, 104428.CrossRefGoogle Scholar
Pearce, D.J.G. 2020 Defect order in active nematics on a curved surface. New J. Phys. 22, 063051.CrossRefGoogle Scholar
Pearce, D.J.G., Ellis, P.W., Fernandez-Nieves, A. & Giomi, L. 2019 Geometrical control of active turbulence in curved topographies. Phys. Rev. Lett. 122, 168002.CrossRefGoogle ScholarPubMed
Powers, T. 2010 Dynamics of filaments and membranes in a viscous fluid. Rev. Mod. Phys. 82, 1607–1631.CrossRefGoogle Scholar
Prost, J., Jülicher, F. & Joanny, J.-F. 2015 Active gel physics. Nat. Phys. 11, 111–117.CrossRefGoogle Scholar
Ramaswamy, S. 2017 Active matter. J. Stat. Mech. 2017, 054002.CrossRefGoogle Scholar
Rangamani, P., Agrawal, A., Mandadapu, K.K., Oster, G. & Steigmann, D.J. 2013 Interaction between surface shape and intra-surface viscous flow on lipid membranes. Biomech. Model. Mechanobiol. 12, 833.CrossRefGoogle ScholarPubMed
Rank, M. & Voigt, A. 2021 Active flows on curved surfaces. Phys. Fluids 33, 072110.CrossRefGoogle Scholar
Rayleigh, Lord 1892 On the instability of a cylinder of viscous liquid under capillary force. Lond. Edinb. Dublin Philos. Mag. J. Sci. 34, 145–154.CrossRefGoogle Scholar
da Rocha, H.B., Bleyer, J. & Turlier, H. 2022 A viscous active shell theory of the cell cortex. J. Mech. Phys. Solids 164, 104876.CrossRefGoogle Scholar
Saffman, P.G. 1975 Brownian motion in thin sheets of viscous fluid. J. Fluid Mech. 73, 593–602.CrossRefGoogle Scholar
Saffman, P.G. & Delbrück, M. 1975 Brownian motion in biological membranes. Proc. Natl Acad. Sci. USA 72, 3111–3113.CrossRefGoogle ScholarPubMed
Sahu, A., Glisman, A., Tchoufag, J. & Mandadapu, K.K. 2020 a Geometry and dynamics of lipid membranes: the Scriven–Love number. Phys. Rev. E 101, 052401.CrossRefGoogle ScholarPubMed
Sahu, A., Omar, Y.A.D., Sauer, R.A. & Mandadapu, K.K. 2020 b Arbitrary Lagrangian–Eulerian finite element method for curved and deforming surfaces. I. General theory and application to fluid interfaces. J. Comput. Phys. 407, 109253.CrossRefGoogle Scholar
Salbreux, G. & Jülicher, F. 2017 Mechanics of active surfaces. Phys. Rev. E 96, 032404.CrossRefGoogle ScholarPubMed
Salbreux, G., Jülicher, F., Prost, J. & Callen-Jones, A. 2022 Theory of nematic and polar active fluid surfaces. Phys. Rev. Res. 4, 033158.CrossRefGoogle Scholar
Sanchez, T., Chen, D.T.N., DeCamp, S.J., Heymann, M. & Dogic, Z. 2012 Spontaneous motion in hierarchically assembled active matter. Nature 491, 431–435.CrossRefGoogle ScholarPubMed
Santiago, J.A. 2018 Stresses in curved nematic membranes. Phys. Rev. E 97, 052706.CrossRefGoogle ScholarPubMed
Santiago, J.A., Chacón-Acosta, G. & Monroy, F. 2019 Membrane stress and torque induced by Frank's nematic textures: a geometric perspective using surface-based constraints. Phys. Rev. E 100, 012704.CrossRefGoogle ScholarPubMed
Santiago, J.A. & Monroy, F. 2020 Mechanics of nematic membranes: Euler–Lagrange equations, Noether charges, stress, torque and boundary conditions of the surface Frank's nematic field. J. Phys. A: Math. Theor. 53, 165201.CrossRefGoogle Scholar
Saw, T.B., Doostmohammadi, A., Nier, V., Kocgozlu, L., Thampi, S., Toyama, Y., Marcq, P., Lim, C.T., Yeomans, J.M. & Ladoux, B. 2017 Topological defects in epithelia govern cell death and extrusion. Nature 544, 212–216.CrossRefGoogle ScholarPubMed
Scriven, L.E. 1960 Dynamics of a fluid interface equation of motion for Newtonian surface fluids. Chem. Engng Sci. 12, 98–108.CrossRefGoogle Scholar
Selinger, J.V., MacKintosh, F.C. & Schnur, J.M. 1996 Theory of cylindrical tubules and helical ribbons of chiral lipid membranes. Phys. Rev. E 53, 043804.CrossRefGoogle ScholarPubMed
Simon, C., et al. 2019 Actin dynamics drive cell-like membrane deformation. Nat. Phys. 15, 602–609.CrossRefGoogle Scholar
Steigmann, D.J. 1999 Fluid films with curvature elasticity. Arch. Rational Mech. Anal. 150, 127.CrossRefGoogle Scholar
Tchoufag, J., Sahu, A. & Mandadapu, K.K. 2022 Absolute vs convective instabilities and front propagation in lipid membrane tubes. Phys. Rev. Lett. 128, 068101.CrossRefGoogle ScholarPubMed
Terzi, M.M. & Deserno, M. 2017 Novel tilt-curvature coupling in lipid membranes. J. Chem. Phys. 147, 084702.CrossRefGoogle ScholarPubMed
Tomotika, S. 1935 On the instability of a cylindrical thread of a viscous liquid surrounded by another viscous fluid. Proc. R. Soc. Lond. A 150, 322–337.Google Scholar
Torres-Sánchez, A., Millán, D. & Arroyo, M. 2019 Modelling fluid deformable surfaces with an emphasis on biological interfaces. J. Fluid Mech. 872, 218–271.CrossRefGoogle Scholar
Torres-Sánchez, A., Santos-Oliván, D. & Arroyo, M. 2020 Approximation of tensor fields on surfaces of arbitrary topology based on local Monge parametrizations. J. Comput. Phys. 405, 109168.CrossRefGoogle Scholar
Vafa, F. & Mahadevan, L. 2022 Active nematic defects and epithelial morphogenesis. Phys. Rev. Lett. 129, 098102.CrossRefGoogle ScholarPubMed
Vasan, R., Rudraraju, S., Akamatsu, M., Garikipati, K. & Rangamani, P. 2020 A mechanical model reveals that non-axisymmetric buckling lowers the energy barrier associated with membrane neck constriction. Soft Matt. 16, 784.CrossRefGoogle ScholarPubMed
Waxman, A.M. 1984 Dynamics of a couple-stress fluid membrane. Stud. Appl. Maths 70, 63–86.CrossRefGoogle Scholar
Zhong-Can, O.-Y. & Helfrich, W. 1989 Bending energy of vesicle membranes: general expressions for the first, second, and third variation of the shape energy and applications to spheres and cylinders. Phys. Rev. A 39, 5280.CrossRefGoogle Scholar