Hostname: page-component-77c89778f8-sh8wx Total loading time: 0 Render date: 2024-07-17T02:56:41.176Z Has data issue: false hasContentIssue false
  • English
  • Français

The stability of oceanic fronts in a shallow water model

Published online by Cambridge University Press:  23 November 2015

Melanie Chanona ,
F. J. Poulin  and
J. Yawney
Melanie Chanona
Affiliation:
Department of Applied Mathematics, University of Waterloo, Waterloo, ON N2L 3G1, Canada
F. J. Poulin*
Affiliation:
Department of Applied Mathematics, University of Waterloo, Waterloo, ON N2L 3G1, Canada
J. Yawney
Affiliation:
Department of Applied Mathematics, University of Waterloo, Waterloo, ON N2L 3G1, Canada
*
†Email address for correspondence: fpoulin@uwaterloo.ca
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate mechanisms through which energy cascades from the mesoscale, 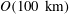$O(100~\text{km})$, to the submesoscale, 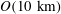$O(10~\text{km})$, for oceanic fronts in a reduced gravity shallow water model using two different profiles. The first idealization of an ocean front has an interfacial depth that is a smooth hyperbolic tangent profile and is an extension of the piecewise constant potential vorticity profile studied in Boss et al. (J. Fluid Mech., vol. 315, 1996, pp. 65–84). By considering a range of minimum depths, all of which have the same velocity profile, we are better able to isolate the effect of vanishing layer depths. We find that the most unstable mode exists in a one-layer model and does not need two layers, as previously speculated. Moreover, we find that even without a vanishing layer depth there are other modes that appear at both larger and smaller length scales that have a gravity wave structure. The second profile is the parabolic double front from Scherer & Zeitlin (J. Fluid Mech., vol. 613, 2008, pp. 309–327). We find more unstable modes than previously presented and also categorize them based on the mode number. In particular, we find there are pairs of unstable modes that have equal growth rates. We also study the nonlinear evolution of these oceanic fronts. It is determined that vanishing layer depths have significant effects on the unstable dynamics that arise. First, stronger gravity wave fields are generated. Second, cyclonic fluid that moves into the deeper waters is stretched preferentially more in comparison to the deep water scenario and destabilizes more easily. This results in smaller scale vortices, both cyclones and anticyclones, that have length scales in the submesoscale regime. Our results suggest that the nonlinear dynamics of a front can be very efficient at generating submesoscale motions.

JFM classification

Instability: Nonlinear instability Geophysical and Geological Flows: Ocean processes Geophysical and Geological Flows: Shallow water flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 785 , 25 December 2015 , pp. 462 - 485
Check for updates
Copyright
© 2015 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bartello, P. 1995 Geostrophic adjustment and inverse cascades in rotating stratified turbulence. J. Atmos. Sci. 52 (24), 4410–4428.2.0.CO;2>CrossRefGoogle Scholar
Boss, E., Paldor, N. & Thompson, L. 1996 Stability of a potential vorticity front: from quasi-geostrophy to shallow water. J. Fluid Mech. 315, 65–84.CrossRefGoogle Scholar
Bouchut, F., Ribstein, B. & Zeitlin, V. 2011 Inertial, barotropic and baroclinic instabilities of the Bickley jet in two-layer rotating shallow water model. Phys. Fluids 23, 126601,1–22.CrossRefGoogle Scholar
Capet, X., McWilliams, J. C., Molemaker, M. J. & Shchepetkin, A. F. 2008a Mesoscale to submesoscale transition in the California current system. Part I: flow structure, eddy flux, and observational tests. J. Phys. Oceanogr. 38, 29–43.CrossRefGoogle Scholar
Capet, X., McWilliams, J. C., Molemaker, M. J. & Shchepetkin, A. F. 2008b Mesoscale to submesoscale transition in the California current system. Part II: frontal processes. J. Phys. Oceanogr. 38, 44–64.CrossRefGoogle Scholar
Capet, X., McWilliams, J. C., Molemaker, M. J. & Shchepetkin, A. F. 2008c Mesoscale to submesoscale transition in the California current system. Part III: energy balance and flux. J. Phys. Oceanogr. 38, 2256–2269.CrossRefGoogle Scholar
Dritschel, D. G. & Vanneste, J. 2006 Instability of a shallow-water potential-vorticity front. J. Fluid Mech. 561, 237–254.CrossRefGoogle Scholar
Flierl, G. R., Malanotte-Rizzoli, P. & Zabusky, N. J. 1987 Nonlinear waves and coherent vortex structures in barotropic beta-plane jets. J. Phys. Oceanogr. 17, 1408–1438.2.0.CO;2>CrossRefGoogle Scholar
Ford, R.1993 Gravity wave generation by vortical flows in a rotating frame. PhD thesis, University of Cambridge.Google Scholar
Ford, R. 1994 Gravity wave radiation from vortex trains in rotationg shallow water. J. Fluid Mech. 281, 81–118.CrossRefGoogle Scholar
Frisch, U. 1995 Turbulence, the Legacy of A. N. Kolmogorov. Cambridge University Press.CrossRefGoogle Scholar
Gula, J., Plougonven, R. & Zeitlin, V. 2009 Ageostrophic instabilities of fronts in a channel in a stratified rotating fluid. J. Fluid Mech. 627, 485–507.Google Scholar
Gula, J. & Zeitlin, V. 2010 Instabilities of buoyancy-driven coastal currents and their nonlinear evolution in the two-layer rotating shallow water model. Part 1. Passive lower layer. J. Fluid Mech. 659, 69–93.Google Scholar
Iga, K. 1993 Reconsideration of Orlanski’s instability theory of frontal waves. J. Fluid Mech. 255, 213–236.CrossRefGoogle Scholar
Iga, K. 1999 Critical layer instability as a resonance between a non-singular mode and continuous modes. Fluid Dyn. Res. 25, 63–86.CrossRefGoogle Scholar
Iga, K. & Ikazaki, H. 2000 Instability of a front formed by two layers with uniform potential vorticity. J. Met. Soc. Japan 78 (4), 491–498.Google Scholar
Irwin, R. L. & Poulin, F. J. 2014 Geophysical instabilities in a two-layer, rotating shallow water model. J. Phys. Oceanogr. 44, 2718–2738.CrossRefGoogle Scholar
Killworth, P. D., Paldor, N. & Stern, M. 1984 Wave propagation and growth on a surface front in a two-layer geostrophic current. J. Mar. Res. 42, 761–785.CrossRefGoogle Scholar
Lahaye, N. & Zeitlin, V. 2015 Centrifugal, barotropic and baroclinic instabilities of isolated ageostrophic anticyclones in the two-layer rotating shallow water model and their nonlinear saturation. J. Fluid Mech. 762, 5–34.CrossRefGoogle Scholar
Liu, X., Osher, S. & Chan, T. 1994 Weighted essentially non-oscillatory schemes. J. Comput. Phys. 115 (1), 200–212.CrossRefGoogle Scholar
Olson, D. B. 1991 Rings in the ocean. Annu. Rev. Earth Planet. Sci. 19, 283–311.CrossRefGoogle Scholar
Paldor, N. 1983 Linear stability and stable modes of geostrophic fronts. Geophys. Astrophys. Fluid Dyn. 24, 299–326.Google Scholar
Pedlosky, J. 1987 Geophysical Fluid Dynamics. Springer.Google Scholar
Perret, G., Dubos, T. & Stegner, A. 2011 How large-scale and cyclogeostrophic barotropic instabilities favor the formation of anticyclonic vorticies in the ocean. J. Phys. Oceanogr. 41, 303–328.Google Scholar
Poulin, F. J. & Flierl, G. R. 2003 The nonlinear evolution of barotropically unstable jets. J. Phys. Oceanogr. 33 (10), 2173–2192.2.0.CO;2>CrossRefGoogle Scholar
Poulin, F. J. & Swaters, G. E. 1999 Sub-inertial dynamics of density-driven flows in a continuously stratified fluid on a sloping bottom. Part 1: model derivations and stabilty characteristics. Proc. R. Soc. Lond. A 455, 2281–2304.Google Scholar
Ribstein, B. & Zeitlin, V. 2013 Instabilities of coupled density fronts and their nonlinear evolution in the two-layer rotating shallow-water model: influence of the lower layer and the topography. J. Fluid Mech. 716, 528–565.CrossRefGoogle Scholar
Ripa, P. 1983 General stability conditions for zonal flows in a one-layer model on the ${\it\beta}$ -plane or the sphere. J. Fluid Mech. 126, 463–498.CrossRefGoogle Scholar
Scherer, E. & Zeitlin, V. 2008 Instability of coupled geostrophic density fronts and its nonlinear evolution. J. Fluid Mech. 613, 309–327.CrossRefGoogle Scholar
Shu, C. & Osher, S. 1988 Efficient implementation of essentially non-oscillatory shock-capturing schemes. J. Comput. Phys. 77 (2), 439–471.CrossRefGoogle Scholar
Talley, L. D., Pickard, G. L., Emery, W. J. & Swift, J. H. 2011 Descriptive Physical Oceanography: An Introduction. Elsevier.CrossRefGoogle Scholar
Teigen, S. H.2011 Water mass exchange in the sea west of Svalbard: a process study of flow instability and vortex generated heat fluxes in the West Spitsbergen Current. PhD thesis, University of Bergen.Google Scholar
Teigen, S. H., Nilsen, F., Skogseth, R., Gjevik, B. & Beszcynska-Möller, A. 2011 Baroclinic instability in the West Spitsbergen Current. J. Geophys. Res. 116, C07012.Google Scholar
Trefethen, L. N. 2000 Spectral Methods in MATLAB. SIAM.Google Scholar
Warn, T. 1986 Statistical mechanical equilibria of the shallow water equations. Tellus 38A, 1–11.CrossRefGoogle Scholar
Zeitlin, V.(Ed.) 2007 Nonlinear Dynamics of Rotating Shallow Water: Methods and Advances, vol. 2. Elsevier.Google Scholar
Zhang, X. & Shu, C. 2011 Maximum-principle-satisfying and positivity-preserving high-order schemes for conservation laws. Proc. R. Soc. Lond. A 467 (2134), 2752–2776.Google Scholar

Chanona et al. supplementary movie

Animation of the divergence of the mass flux for the nonlinear simulation of the parabolic double front.

Download Chanona et al. supplementary movie(Video)
Video 3.8 MB

Chanona et al. supplementary movie

Animation of the layer depth for the nonlinear simulation of the parabolic double front.

Download Chanona et al. supplementary movie(Video)
Video 943.9 KB

Chanona et al. supplementary movie

Animation of the vorticity for the nonlinear simulation of the parabolic double front.

Download Chanona et al. supplementary movie(Video)
Video 3.3 MB

Chanona et al. supplementary movie

Animation of the divergence of the mass flux for the nonlinear simulation of the Bickley jet with d = 0.

Download Chanona et al. supplementary movie(Video)
Video 7.7 MB

Chanona et al. supplementary movie

Animation of the layer depth for the nonlinear simulation of the Bickley jet with d = 0.

Download Chanona et al. supplementary movie(Video)
Video 1.7 MB

Chanona et al. supplementary movie

Animation of the vorticity for the nonlinear simulation of the Bickley jet with d = 0

Download Chanona et al. supplementary movie(Video)
Video 6.9 MB

Chanona et al. supplementary movie

Animation of the divergence of the mass flux for the nonlinear simulation of the Bickley jet with d = 150.

Download Chanona et al. supplementary movie(Video)
Video 12.8 MB

Chanona et al. supplementary movie

Animation of the layer depth for the nonlinear simulation of the Bickley jet with d = 150.

Download Chanona et al. supplementary movie(Video)
Video 2.2 MB

Chanona et al. supplementary movie

Animation of the vorticty for the nonlinear simulation of the Bickley jet with d = 150.

Download Chanona et al. supplementary movie(Video)
Video 5.1 MB