Hostname: page-component-8448b6f56d-xtgtn Total loading time: 0 Render date: 2024-04-23T09:50:48.825Z Has data issue: false hasContentIssue false
  • English
  • Français

Population-size-dependent branching process with linear rate of growth

Published online by Cambridge University Press:  14 July 2016

F. C. Klebaner
F. C. Klebaner*
Affiliation:
University of Melbourne
*
∗ Postal address: Department of Statistics, Richard Berry Building, University of Melbourne, Parkville, VIC 3052, Australia.
Get access Rights & Permissions [Opens in a new window]

Abstract

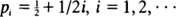The process we consider is a binary splitting, where the probability of division, , depends on the population size, 2i. We show that Zn converges to ∞ almost surely on a set 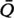of positive probability. Zn/n converges in distribution to a proper limit, 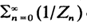diverges almost surely on converges almost surely on 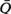and there are no constants cn such that Zn/cn converges in probability to a non-degenerate limit.

Keywords

MARKOV CHAINSPOPULATION MODELSTATE DEPENDENCEGROWTH RATES
Type
Research Papers
Information
Journal of Applied Probability , Volume 20 , Issue 2 , June 1983 , pp. 242 - 250
Copyright
Copyright © Applied Probability Trust 1983 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

[1] Feller, W. (1971) An Introduction to Probability Theory and its Applications, Vol. 2. Wiley, New York.Google Scholar
[2] Labkovskii, V. A. (1972) A limit theorem for generalized random branching process depending on the size of the population. Theory Prob. Appl. 17, 72–85.CrossRefGoogle Scholar
[3] Levina, L. V., Leontovich, A. M. and Piatetski-Shapiro, I. I. (1968) On a regulative branching process. Problemy Pederaci Informatsii 4, 72–82.Google Scholar
[4] Loève, M. (1955) Probability Theory. Foundations. Random Sequences. Van Nostrand, New York.Google Scholar
[5] Rao, K. S. and Kendall, D. G. (1950) On the generalized second limit-theorem in the calculus of probabilities. Biometrika 37, 224–230.CrossRefGoogle ScholarPubMed
[6] Schuh, H.-J. (1982) Sums of i.i.d. random variables and an application to the explosion criterion for Markov branching processes. J. Appl. Prob. 19, 29–38.CrossRefGoogle Scholar
[7] Teicher, H. (1980) Almost certain behaviour of row sums of double arrays. Conf. Analytic Methods for Probability Theory, Oberwolfach, Springer-Verlag, Berlin.Google Scholar