Article contents
A strong law of Erdös-Rényi type for cumulative processes in renewal theory
Published online by Cambridge University Press: 14 July 2016
Abstract
Let {Nt}t >0 be a renewal counting process (cf. Parzen (1962), p. 160) with underlying failure times 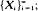 let
let 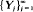 be a sequence of non-negative random variables and {Zt}t >0 an associated cumulative process, i.e.
be a sequence of non-negative random variables and {Zt}t >0 an associated cumulative process, i.e. 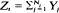 if Nt = 1, 2, …, and Zt = 0, if Nt = 0. By convention set Z0 = 0. Consider
if Nt = 1, 2, …, and Zt = 0, if Nt = 0. By convention set Z0 = 0. Consider 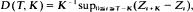 the maximum increment of the process {Zt}t >0 in [0, T] over a time K, 0 < K < T, divided by K. Under appropriate conditions it is shown that for a wide range of numbers a there exist constants C(a), uniquely determined by a and the distributions of the Xi's and Yj's, such that D(T, C log T) converges to a with probability 1. This result provides a renewal theoretic variant of Erdös and Rényi's (1970) ‘new law of large numbers’.
the maximum increment of the process {Zt}t >0 in [0, T] over a time K, 0 < K < T, divided by K. Under appropriate conditions it is shown that for a wide range of numbers a there exist constants C(a), uniquely determined by a and the distributions of the Xi's and Yj's, such that D(T, C log T) converges to a with probability 1. This result provides a renewal theoretic variant of Erdös and Rényi's (1970) ‘new law of large numbers’.
- Type
- Research Papers
- Information
- Copyright
- Copyright © Applied Probability Trust 1978
References
- 7
- Cited by




