Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Groenewald, P. C. N.
Ferreira, A. V.
van der Merwe, H. J.
and
Slippers, S. C.
1995.
A mathematical model for describing and predicting the lactation curve of Merino ewes.
Animal Science,
Vol. 61,
Issue. 1,
p.
95.
Pérochon, L.
Coulon, J. B.
and
Lescourret, F.
1996.
Modelling lactation curves of dairy cows with emphasis on individual variability.
Animal Science,
Vol. 63,
Issue. 2,
p.
189.
Groenewald, P. C. N.
Ferreira, A. V.
van der Merwe, H. J.
and
Slippers, S. C.
1996.
Application of Bayesian inference in the comparison of lactation curves of Merino ewes.
Animal Science,
Vol. 62,
Issue. 1,
p.
63.
Dijkstra, J.
France, J.
Dhanoa, M.S.
Maas, J.A.
Hanigan, M.D.
Rook, A.J.
and
Beever, D.E.
1997.
A Model to Describe Growth Patterns of the Mammary Gland During Pregnancy and Lactation.
Journal of Dairy Science,
Vol. 80,
Issue. 10,
p.
2340.
Cappio-Borlino, A.
Portolano, B.
Todaro, M.
Macciotta, N.P.P.
Giaccone, P.
and
Pulina, G.
1997.
Lactation Curves of Valle del Belice Dairy Ewes for Yields of Milk, Fat, and Protein Estimated with Test Day Models.
Journal of Dairy Science,
Vol. 80,
Issue. 11,
p.
3023.
Macciotta, N.P.P.
Cappio-Borlino, A.
and
Pulina, G.
1999.
Analysis of Environmental Effects on Test Day Milk Yields of Sarda Dairy Ewes.
Journal of Dairy Science,
Vol. 82,
Issue. 10,
p.
2212.
Pollott, G. E.
1999.
Describing the lactation curve of dairy animals.
Proceedings of the British Society of Animal Science,
Vol. 1999,
Issue. ,
p.
197.
Friggens, N.C.
Emmans, G.C.
and
Veerkamp, R.F.
1999.
On the use of simple ratios between lactation curve coefficients to describe parity effects on milk production.
Livestock Production Science,
Vol. 62,
Issue. 1,
p.
1.
Rodriguez-Zas, Sandra L.
Gianola, Daniel
and
Shook, George E.
2000.
Evaluation of models for somatic cell score lactation patterns in Holsteins.
Livestock Production Science,
Vol. 67,
Issue. 1-2,
p.
19.
Pollott, G. E.
and
Gootwine, E.
2000.
Appropriate mathematical models for describing the complete lactation of dairy sheep.
Animal Science,
Vol. 71,
Issue. 2,
p.
197.
Vargas, B.
Koops, W.J.
Herrero, M.
and
Van Arendonk, J.A.M.
2000.
Modeling Extended Lactations of Dairy Cows.
Journal of Dairy Science,
Vol. 83,
Issue. 6,
p.
1371.
Pollott, G.E.
2000.
A Biological Approach to Lactation Curve Analysis for Milk Yield.
Journal of Dairy Science,
Vol. 83,
Issue. 11,
p.
2448.
Macciotta, N.P.P.
Cappio-Borlino, A.
and
Pulina, G.
2000.
Time Series Autoregressive Integrated Moving Average Modeling of Test-Day Milk Yields of Dairy Ewes.
Journal of Dairy Science,
Vol. 83,
Issue. 5,
p.
1094.
Emmans, Gerry
and
Kyriazakis, Ilias
2001.
Consequences of genetic change in farm animals on food intake and feeding behaviour.
Proceedings of the Nutrition Society,
Vol. 60,
Issue. 1,
p.
115.
Martin, O.
and
Sauvant, D.
2002.
Metaanalysis of Input/Output Kinetics in Lactating Dairy Cows.
Journal of Dairy Science,
Vol. 85,
Issue. 12,
p.
3363.
Kominakis, A.P.
Abas, Z.
Maltaris, I.
and
Rogdakis, E.
2002.
A preliminary study of the application of artificial neural networks to prediction of milk yield in dairy sheep.
Computers and Electronics in Agriculture,
Vol. 35,
Issue. 1,
p.
35.
Grossman, M.
and
Koops, W.J.
2003.
Modeling Extended Lactation Curves of Dairy Cattle: A Biological Basis for the Multiphasic Approach.
Journal of Dairy Science,
Vol. 86,
Issue. 3,
p.
988.
Cant, John
2003.
Mathematical Modeling in Nutrition and the Health Sciences.
Vol. 537,
Issue. ,
p.
239.
Pulina, Giuseppe
Di Mauro, Corrado
Macciotta, Niccolò
and
Cappio-Borlino, Aldo
2003.
Determinism, Holism, and Complexity.
p.
245.
Prasad, Shiv
2003.
Modeling of Lactation Curves of Dairy Animals.
Journal of Applied Animal Research,
Vol. 24,
Issue. 1,
p.
79.
 (Mitscherlich),
(Mitscherlich), 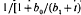 (Michaelis-Menten),
(Michaelis-Menten), 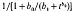 (generalized saturation kinetic), 1/(1 + b0
(generalized saturation kinetic), 1/(1 + b0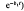 (logistic), b0 exp
(logistic), b0 exp 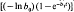 (Gompertz) and [1+ tanh(b0 + b1t)]/2 (hyperbolic tangent). Candidates for ϕ2 were e–ct (exponential) and 1/(1 + ct) (inverse straight line). The 12 models thus obtained and Y = Atb e–ct (Wood's model) were fitted to whole-lactation data from 23 animals. Mitscherlich x exponential, Michaelis-Menten x exponential, logistic x exponential, logistic × inverse straight line and Wood's model all fitted well. For these models, expressions for time to peak, maximum yield, total yield over a finite lactation and relative decline at the midway point of the declining phase were obtained. The Mitscherlich x exponential model generally fitted better than Wood's model and, unlike Wood's model, gives simple algebraic formulae for all these summary statistics.
(Gompertz) and [1+ tanh(b0 + b1t)]/2 (hyperbolic tangent). Candidates for ϕ2 were e–ct (exponential) and 1/(1 + ct) (inverse straight line). The 12 models thus obtained and Y = Atb e–ct (Wood's model) were fitted to whole-lactation data from 23 animals. Mitscherlich x exponential, Michaelis-Menten x exponential, logistic x exponential, logistic × inverse straight line and Wood's model all fitted well. For these models, expressions for time to peak, maximum yield, total yield over a finite lactation and relative decline at the midway point of the declining phase were obtained. The Mitscherlich x exponential model generally fitted better than Wood's model and, unlike Wood's model, gives simple algebraic formulae for all these summary statistics.



