Hostname: page-component-7479d7b7d-8zxtt Total loading time: 0 Render date: 2024-07-10T11:30:24.182Z Has data issue: false hasContentIssue false
  • English
  • Français

Controllablity of a quantum particle in a 1D variable domain

Published online by Cambridge University Press:  21 September 2007

Karine Beauchard
Karine Beauchard*
Affiliation:
CMLA, ENS Cachan, 61 avenue du président Wilson, 94235 Cachan cedex, France; Karine.Beauchard@cmla.ens-cachan.fr
Get access

Abstract

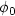We consider a quantum particle in a 1D infinite square potential well with variable length. It is a nonlinear control system in which the state is the wave function ϕ of the particle and the control is the length l(t) of the potential well. We prove the following controllability result : given $\phi_{0}$ close enough to an eigenstate corresponding to the length l = 1 and $\phi_{f}$ 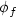close enough to another eigenstate corresponding to the length l=1, there exists a continuous function $l:[0,T] \rightarrow \mathbb{R}^{*}_{+}$ 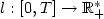with T > 0, such that l(0) = 1 and l(T) = 1, and which moves the wave function from $\phi_{0}$ 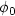to $\phi_{f}$ 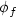in time T. In particular, we can move the wave function from one eigenstate to another one by acting on the length of the potential well in a suitable way. Our proof relies on local controllability results proved with moment theory, a Nash-Moser implicit function theorem and expansions to the second order.

Keywords

ControllabilitySchrödinger equationNash-Moser theoremmoment theory
Type
Research Article
Information
ESAIM: Control, Optimisation and Calculus of Variations , Volume 14 , Issue 1 , January 2008 , pp. 105 - 147
Copyright
© EDP Sciences, SMAI, 2007

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Albertini, F. and D'Alessandro, D., Notions of controllability for bilinear multilevel quantum systems. IEEE Trans. Automat. Control 48 (2003) 1399–1403. CrossRef
S. Alinhac and P. Gérard, Opérateurs pseudo-différentiels et théorème de Nash-Moser. Intereditions (Paris), collection Savoirs actuels (1991).
Altafini, C., Controllability of quantum mechanical systems by root space decomposition of su(n). J. Math. Phys. 43 (2002) 2051–2062. CrossRef
J.M. Ball, J.E. Marsden and M. Slemrod, Controllability for distributed bilinear systems. SIAM J. Control Optim. 20 (1982).
Baudouin, L., A bilinear optimal control problem applied to a time dependent Hartree-Fock equation coupled with classical nuclear dynamics. Portugaliae Matematica (N.S.) 63 (2006) 293–325.
Baudouin, L. and Salomon, J., Constructive solution of a bilinear control problem. C.R. Math. Acad. Sci. Paris 342 (2006) 119–124. CrossRef
Baudouin, L., Kavian, O. and Puel, J.-P., Regularity for a Schrödinger equation with singular potential and application to bilinear optimal control. J. Differential Equations 216 (2005) 188–222. CrossRef
K. Beauchard, Local controllability of a 1-D beam equation. SIAM J. Control Optim. (to appear).
Beauchard, K., Local Controllability of a 1-D Schrödinger equation. J. Math. Pures Appl. 84 (2005) 851–956. CrossRef
Beauchard, K. and Coron, J.-M., Controllability of a quantum particle in a moving potential well. J. Functional Analysis 232 (2006) 328–389. CrossRef
Brockett, R., Lie theory and control systems defined on spheres. SIAM J. Appl. Math. 25 (1973) 213–225. CrossRef
E. Cancès, C. Le Bris and M. Pilot, Contrôle optimal bilinéaire d'une équation de Schrödinger. C.R. Acad. Sci. Paris, Série I 330 (2000) 567–571.
Coron, J.-M., Global asymptotic stabilization for controllable systems without drift. Math. Control Signals Systems 5 (1992) 295–312. CrossRef
Coron, J.-M., Contrôlabilité exacte frontière de l'équation d'Euler des fluides parfaits incompressibles bidimensionnels. C. R. Acad. Sci. Paris 317 (1993) 271–276.
Coron, J.-M., On the controllability of 2-D incompressible perfect fluids. J. Math. Pures Appl. 75 (1996) 155–188.
Coron, J.-M., Local Controllability of a 1-D Tank Containing a Fluid Modeled by the shallow water equations. ESAIM: COCV 8 (2002) 513–554. CrossRef
J.-M. Coron, On the small-time local controllability of a quantum particule in a moving one-dimensional infinite square potential well. C.R. Acad. Sci., Série I 342 (2006) 103–108.
Coron, J.-M. and Crépeau, E., Exact boundary controllability of a nonlinear KdV equation with critical lengths. J. Eur. Math. Soc. 6 (2004) 367–398. CrossRef
Coron, J.-M. and Fursikov, A., Global exact controllability of the 2D Navier-Stokes equation on a manifold without boundary. Russ. J. Math. Phys. 4 (1996) 429–448.
Fursikov, A.V. and Imanuvilov, O.Yu., Exact controllability of the Navier-Stokes and Boussinesq equations. Russian Math. Surveys 54 (1999) 565–618. CrossRef
Glass, O., On the controllability of the 1D isentropic Euler equation. J. European Mathematical Society 9 (2007) 427–486. CrossRef
Glass, O., Exact boundary controllability of 3-D Euler equation. ESAIM: COCV 5 (2000) 1–44. CrossRef
Glass, O., On the controllability of the Vlasov-Poisson system. J. Differential Equations 195 (2003) 332–379. CrossRef
G. Gromov, Partial Differential Relations. Springer-Verlag, Berlin-New York-London (1986).
Haraux, A., Séries lacunaires et contrôle semi-interne des vibrations d'une plaque rectangulaire. J. Math. Pures Appl. 68 (1989) 457–465.
L. Hörmander, On the Nash-Moser Implicit Function Theorem. Annales Academiae Scientiarum Fennicae (1985) 255–259.
Horsin, T., On the controllability of the Burgers equation. ESAIM: COCV 3 (1998) 83–95. CrossRef
Ilner, R., Lange, H. and Teismann, H., Limitations on the control of Schrödinger equations. ESAIM: COCV 12 (2006) 615–635. CrossRef
T. Kato, Perturbation Theory for Linear operators. Springer-Verlag, Berlin, New-York (1966).
W. Krabs, On moment theory and controllability of one-dimensional vibrating systems and heating processes. Springer – Verlag (1992).
Lasiecka, I. and Triggiani, R., Optimal regularity, exact controllability and uniform stabilization of Schrödinger equations with Dirichlet controls. Differential Integral Equations 5 (1992) 571–535.
Lasiecka, I., Triggiani, R. and Zhang, X., Global uniqueness, observability and stabilization of nonconservative Schrödinger equations via pointwise Carlemann estimates. J. Inverse Ill Posed-Probl. 12 (2004) 183–231.
Lebeau, G., Contrôle de l'équation de Schrödinger. J. Math. Pures Appl. 71 (1992) 267–291.
Machtyngier, Exact, controllability for the Schrödinger equation. SIAM J. Contr. Opt. 32 (1994) 24–34.
Mirrahimi, M. and Rouchon, P., Controllability of quantum harmonic oscillators. IEEE Trans. Automat. Control 49 (2004) 745–747. CrossRef
Sontag, E., Control of systems without drift via generic loops. IEEE Trans. Automat. Control 40 (1995) 1210–1219. CrossRef
G. Turinici, On the controllability of bilinear quantum systems, in Mathematical Models and Methods for Ab Initio Quantum Chemistry, C. Le Bris and M. Defranceschi Eds., Lect. Notes Chemistry 74, Springer (2000).
Zuazua, E., Remarks on the controllability of the Schrödinger equation. CRM Proc. Lect. Notes 33 (2003) 193–211. CrossRef