Hostname: page-component-5c6d5d7d68-7tdvq Total loading time: 0 Render date: 2024-08-17T15:30:22.120Z Has data issue: false hasContentIssue false
  • English
  • Français

Stable sets and mean Li–Yorke chaos in positive entropy actions of bi-orderable amenable groups

Published online by Cambridge University Press:  01 June 2015

WEN HUANG  and
LEI JIN
WEN HUANG
Affiliation:
Wu Wen-Tsun Key Laboratory of Mathematics, USTC, Chinese Academy of Sciences, PR China Department of Mathematics, University of Science and Technology of China, Hefei, Anhui 230026, PR China email wenh@mail.ustc.edu.cn
LEI JIN
Affiliation:
Department of Mathematics, University of Science and Technology of China, Hefei, Anhui 230026, PR China email jinleim@mail.ustc.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

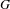It is proved that positive entropy implies mean Li–Yorke chaos for a $G$-system, where 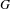$G$ is a countable, infinite, discrete, bi-orderable amenable group. Examples are given for the cases of integer lattice groups and groups of integer unipotent upper triangular matrices.

Type
Research Article
Information
Ergodic Theory and Dynamical Systems , Volume 36 , Issue 8 , December 2016 , pp. 2482 - 2497
Copyright
© Cambridge University Press, 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Akin, E.. Lectures on Cantor and Mycielski sets for dynamical systems. Chapel Hill Ergodic Theory Workshops (Contemporary Mathematics, 356) . American Mathematical Society, Providence, RI, 2004, pp.21–79.Google Scholar
Blanchard, F., Glasner, E., Kolyada, S. and Maass, A.. On Li–Yorke pairs. J. Reine Angew. Math. 547 (2002), 51–68.Google Scholar
Botto-Mura, R. and Rhemtulla, A.. Orderable Groups (Lecture Notes in Pure and Applied Mathematics, 27) . Marcel Dekker, New York–Basel, 1977.Google Scholar
Deroin, B., Navas, A. and Rivas, C.. Groups, orders, and dynamics. Preprint, 2014, arXiv:1408.5805.Google Scholar
Downarowicz, T.. Positive topological entropy implies chaos DC2. Proc. Amer. Math. Soc. 142 (2014), 137–149.Google Scholar
Downarowicz, T. and Lacroix, Y.. Forward mean proximal pairs and zero entropy. Israel J. Math. 191 (2012), 945–957.Google Scholar
Einsiedler, M. and Ward, T.. Ergodic Theory with a View Towards Number Theory (Graduate Texts in Mathematics, 259) . Springer, London, 2011.Google Scholar
Glasner, E.. Ergodic Theory via Joinings (Mathematical Surveys and Monographs, 101) . American Mathematical Society, Providence, RI, 2003.Google Scholar
Huang, W., Li, J. and Ye, X.. Stable sets and mean Li–Yorke chaos in positive entropy systems. J. Funct. Anal. 266(6) (2014), 3377–3394.Google Scholar
Huang, W., Xu, L. and Yi, Y.. Asymptotic pairs, stable sets and chaos in positive entropy systems. J. Funct. Anal. 268(4) (2015), 824–846.Google Scholar
Huang, W. and Ye, X.. Devaney’s chaos or 2-scattering implies Li–Yorke’s chaos. Topology Appl. 117(3) (2002), 259–272.CrossRefGoogle Scholar
Kerr, D. and Li, H.. Independence in topological and C ∗ -dynamics. Math. Ann. 338(4) (2007), 869–926.Google Scholar
Kerr, D. and Li, H.. Combinatorial independence and sofic entropy. Commun. Math. Stat. 1 (2013), 213–257.CrossRefGoogle Scholar
Kopytov, V. M. and Ya. Medvedev, N.. Right Ordered Groups (Siberian School of Algebra and Logic) . Plenum, New York, 1996.Google Scholar
Lindenstrauss, E.. Pointwise theorems for amenable groups. Invent. Math. 146 (2001), 259–295.Google Scholar
Lindenstrauss, E. and Weiss, B.. Mean topological dimension. Israel J. Math. 115 (2000), 1–24.Google Scholar
Ollagnier, J. M. and Pinchon, D.. The variational principle. Studia Math. 72(2) (1982), 151–159.CrossRefGoogle Scholar
Ornstein, D. and Weiss, B.. Mean distality and tightness. Proc. Steklov Inst. Math. 244(1) (2004), 295–302.Google Scholar
Stepin, A. M. and Tagi-Zade, A. T.. Variational characterization of topological pressure of the amenable groups of transformations. Dokl. Akad. Nauk SSSR 254(3) (1980), 545–549 (in Russian).Google Scholar