Hostname: page-component-5c6d5d7d68-wtssw Total loading time: 0 Render date: 2024-08-16T07:44:03.501Z Has data issue: false hasContentIssue false
  • English
  • Français

A New Tautological Relation in 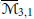via the Invariance Constraint

Published online by Cambridge University Press:  20 November 2018

D. Arcara  and
Y.-P. Lee
D. Arcara
Affiliation:
Department of Mathematics, St. Vincent College, Latrobe, PA, 15650-2690, USA e-mail: daniele.arcara@email.stvincent.edu
Y.-P. Lee
Affiliation:
Department of Mathematics, University of Utah, Salt Lake City, UT, 84112-0090, USA e-mail: yplee@math.utah.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A new tautological relation of 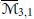${{\overline{M}}_{3,\,1}}$ in codimension 3 is derived and proved, using an invariance constraint from our previous work.

Keywords

14H10
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 52 , Issue 2 , 01 June 2009 , pp. 161 - 174
Copyright
Copyright © Canadian Mathematical Society 2009

Footnotes

The second author is partially supported by NSF and AMS Centennial Fellowship

References

[1] Arcara, D., Lee, Y.-P., Tautological equations in genus 2 via invariance constraints. Bull. Inst. Math. Acad. Sin. (N.S.) 2(2007), no. 1, 1–27.Google Scholar
[2] Arcara, D., Lee, Y.-P., On independence of generators of the tautological rings. http://arxiv.org/abs/math/0605488.Google Scholar
[3] Belorousski, P., Pandharipande, R., A descendent relation in genus 2. Ann. Scuola Norm. Sup. Pisa Cl. Sci. (4) 29(2000), no. 1, 171–191.Google Scholar
[4] Faber, C., Shadrin, S., Zvonkine, D., Tautological relations and the r-spin Witten conjecture. http://arxiv.org/abs/math/0612510.Google Scholar
[5] Getzler, E., Intersection theory on and elliptic Gromov–Witten invariants. J. Amer. Math. Soc. 10(1997), no. 4, 973–998.Google Scholar
[6] Getzler, E., Topological recursion relations in genus 2 . In: Integrable systems and algebraic geometry, World Sci. Publ., River Edge, NJ, 1998, pp. 73–106.Google Scholar
[7] Getzler, E., Looijenga, E., The Hodge polynomial of . http://arxiv.org/abs/math/9910174math.Google Scholar
[8] Graber, T. and Vakil, R., Relative virtual localization and vanishing of tautological classes on moduli spaces of curves. Duke Math. J. 130(2005), no. 1, 1–37.Google Scholar
[9] Kimura, T. and Liu, X., A genus-3 topological recursion relation. Comm. Math. Phys. 262(2006), no. 3, 645–661.Google Scholar
[10] Lee, Y.-P., Invariance of tautological equations. I. Conjectures and applications. J. Euro. Math. Soc. 10(2008), no. 2, 399–413.Google Scholar
[11] Lee, Y.-P., Invariance of tautological equations II: Gromov–Witten theory. http://arxiv.org/abs/math/0605708.Google Scholar
[12] Vakil, R., The moduli space of curves and Gromov-Witten theory. http://arxiv.org/abs/math/0602347.Google Scholar