Hostname: page-component-77c89778f8-fv566 Total loading time: 0 Render date: 2024-07-17T02:21:56.320Z Has data issue: false hasContentIssue false
  • English
  • Français

A Dimension-Free Weak-Type Estimate for Operators on UMD-Valued Functions

Published online by Cambridge University Press:  20 November 2018

Brian P. Kelly
Brian P. Kelly*
Affiliation:
Department of Mathematics, University of Louisiana at Monroe, Monroe, Louisiana 71211, USA
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 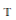$\mathbb{T}$ denote the unit circle in the complex plane, and let 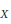$X$ be a Banach space that satisfies Burkholder’s $\text{UMD}$ condition. Fix a natural number, 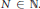$N\,\in \,\mathbb{N}$ . Let 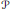$\mathcal{P}$ denote the reverse lexicographical order on 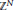${{\mathbb{Z}}^{N}}$ . For each 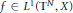$f\,\in \,{{L}^{1}}({{\mathbb{T}}^{N}},X)$ , there exists a strongly measurable function 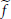$\tilde{f}$ such that formally, for all 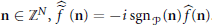$\mathbf{n}\,\in \,{{\mathbb{Z}}^{N}},\,\hat{\tilde{f}}\,\left( \mathbf{n} \right)\,=\,-i\,{{sgn }_{\mathcal{P}}}\left( \mathbf{n} \right)\hat{f}\left( \mathbf{n} \right)$ . In this paper, we present a summation method for this conjugate function directly analogous to the martingale methods developed by Asmar and Montgomery-Smith for scalar-valued functions. Using a stochastic integral representation and an application of Garling’s characterization of $\text{UMD}$ spaces, we prove that the associated maximal operator satisfies a weak-type (1, 1) inequality with a constant independent of the dimension 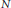$N$.

Keywords

43A1760H3046B09
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 43 , Issue 3 , 01 September 2000 , pp. 355 - 361
Copyright
Copyright © Canadian Mathematical Society 2000

References

[1] Asmar, N., Berkson, E. and Gillespie, T. A., Distributional control and generalized analyticity. Integral Equations Operator Theory 14 (1991), 311–341.Google Scholar
[2] Asmar, N., Kelly, B. P., and Montgomery-Smith, S., A note on UMD spaces and transference in vector-valued function spaces. Proc. Edinburgh Math. Soc. 39 (1996), 485–490.Google Scholar
[3] Asmar, N. and Montgomery-Smith, S., Dimension-free estimates for conjugate maximal functions and pointwise convergence. Studia Math., to appear.Google Scholar
[4] Berkson, E., Gillespie, T. A. and Muhly, P. S., Generalized analyticity in UMD spaces. Ark. Mat. 27 (1989), 1–14.Google Scholar
[5] Bourgain, J., Some remarks on Banach spaces in which martingale difference sequences are unconditional. Ark. Mat. 21 (1983), 163–168.Google Scholar
[6] Burkholder, D., A geometric condition that implies the existence of certain singular integrals of Banach-spacevalued functions. In: Proceedings, Conference on Harmonic Analysis in Honor of A. Zygmund, Chicago, 1981 (eds.W. Becker et al.),Wadsworth, Belmont, CA, 1983, 270–286.Google Scholar
[7] Burkholder, D., Gundy, R. F. and Silverstein, M. L., A maximal characterization of the class Hp. Trans. Amer. Math. Soc. 157 (1971), 137–153.Google Scholar
[8] Doob, J. L., Stochastic Processes. Wiley Publications in Statistics, New York, 1990.Google Scholar
[9] Garling, D. J. H., Brownian motion and UMD spaces. In: Probability and Banach spaces, Springer Lecture Notes in Math. 1221 (1986), 36–49.Google Scholar
[10] Helson, H., Conjugate series in several variables. Pacific J. Math. 9 (1959), 513–523.Google Scholar
[11] Kelly, B. P., Distributional controlled representations acting on vector-valued functions spaces. Doctoral Dissertation, University of Missouri, 1994.Google Scholar
[12] Zygmund, A., Trigonometric Series. 2nd edition (2 vols.), Cambridge University Press, 1959 Google Scholar