Hostname: page-component-848d4c4894-jbqgn Total loading time: 0 Render date: 2024-06-19T06:06:37.499Z Has data issue: false hasContentIssue false
  • English
  • Français

Artinianness of Certain Graded Local Cohomology Modules

Published online by Cambridge University Press:  20 November 2018

Amir Mafi  and
Hero Saremi
Amir Mafi
Affiliation:
Department of Mathematics, University of Kurdistan, P.O. Box: 416, Sanandaj, Iran andInstitute for Research in Fundamental Science (IPM), P.O. Box 19395-5746, Tehran, Iran e-mail: a_mafi@ipm.ir
Hero Saremi
Affiliation:
Department of Mathematics, Islamic Azad University, Sanandaj Branch, Sanandaj, Iranh saremi@iausdj.ac.irherosaremi@yahoo.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We show that if 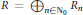$R\,=\,{{\oplus }_{n\in \mathbb{N}0}}\,{{R}_{n}}$ is a Noetherian homogeneous ring with local base ring 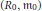$({{R}_{0}},\,{{m}_{0}})$, irrelevant ideal 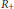${{R}_{+}}$, and 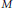$M$ a finitely generated graded $R$-module, then 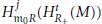$H_{{{m}_{0}}R}^{j}\,(H_{R+}^{t}\,(M))$ is Artinian for 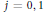$j\,=\,0,\,1$ where 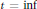$t\,=\,\inf ${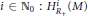$i\in {{\mathbb{N}}_{0}}:H_{R+}^{i}(M)$ is not finitely generated}. Also, we prove that if 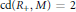$\text{cd(}{{R}_{+}},M)\,=\,2$, then for each 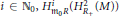$i\,\in \,{{\mathbb{N}}_{0}},\,H_{{{m}_{0}}R}^{i}\,(H_{R+}^{2}\,(M))$ is Artinian if and only if 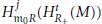$H_{{{m}_{0}}R}^{i+2}(H_{R+}^{1}(M))$ is Artinian, where 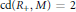$ \text{cd(}{{R}_{+}},\,M)$ is the cohomological dimension of 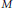$M$ with respect to 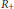${{R}_{+}}$. This improves some results of R. Sazeedeh.

Keywords

13D4513E10graded local cohomologyArtinian modules
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 55 , Issue 1 , 01 March 2012 , pp. 153 - 156
Copyright
Copyright © Canadian Mathematical Society 2012

References

[1] Aghapournahr, M. and Melkersson, L., Artinianness of local cohomology modules. arXiv:0809.3814v1 [math. AC].Google Scholar
[2] Brodmann, M. and Sharp, R. Y., Local cohomology–an algebraic introduction with geometric applications. Cambridge Studies in Advanced Mathematics 60, Cambridge University Press, Cambridge, 1998.Google Scholar
[3] Brodmann, M., Fumasoli, S., and Tajarod, R., Local cohomology over homogeneous rings with one-dimensional local base ring. Proc. Amer. Math. Soc. 131(2003), no. 10, 2977–2985. doi:10.1090/S0002-9939-03-07009-6Google Scholar
[4] Brodmann, M., Rohrer, F., and Sazeedeh, R., Multiplicities of graded components of local cohomology modules. J. Pure Appl. Alg., 197(2005), nos. 1-4, 249–278. doi:10.1016/j.jpaa.2004.08.034Google Scholar
[5] Hellus, M., Matlis duals of top local cohomology modules and the arithmetic rank of an ideal. Comm. Algebra 35(2007), no. 4, 1421–1432. doi:10.1080/00927870601142348Google Scholar
[6] Rotman, J., An Introduction to Homological Algebra. Pure and Applied Mathematics 85, Academic Press, New York, 1979.Google Scholar
[7] Rotthaus, C. and Sega, L. M., Some properties of graded local cohomology modules. J. Algebra 283(2005), no. 1, 232–247. doi:10.1016/j.jalgebra.2004.07.034Google Scholar
[8] Sazeedeh, R., Artinianness of graded local cohomology modules. Proc. Amer. Math. Soc. 135(2007), no. 8, 2339–2345. doi:10.1090/S0002-9939-07-08794-1Google Scholar
[9] Sazeedeh, R., Finiteness of graded local cohomology modules. J. Pure Appl. Algebra 212(2008), no. 1, 275–280. doi:10.1016/j.jpaa.2007.05.023Google Scholar