Hostname: page-component-76dd75c94c-8c549 Total loading time: 0 Render date: 2024-04-30T08:35:32.743Z Has data issue: false hasContentIssue false
  • English
  • Français

Mori's Program for 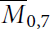with Symmetric Divisors

Published online by Cambridge University Press:  20 November 2018

Han-Bom Moon
Han-Bom Moon*
Affiliation:
Department of Mathematics, Fordham University, Bronx, NY 10458, USA e-mail: hmoon8@fordham.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We complete Mori's program with symmetric divisors for the moduli space of stable seven-pointed rational curves. We describe all birational models in terms of explicit blow-ups and blow-downs. We also give a moduli theoretic description of the first flip, which has not appeared in the literature.

Keywords

14H1014E30moduli of curvesminimal model programMori dream space
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 69 , Issue 3 , 01 June 2017 , pp. 613 - 649
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Alexeev, V. and Swinarski, D., Nef divisors on from GIT. In: Geometry and arithmetic, EMS Ser. Congr. Rep., Eur. Math. Soc., Zurich, 2012, pp. 1–21.http://dx.doi.org/10.4171/119-1/1 Google Scholar
[2] Alper, J., Fedorchuk, M., and Smyth, D. I., Singularities with action and the log minimal model program for . arxiv:1010.3751 Google Scholar
[3] Arcara, D., Bertram, A., Coskun, I., and Huizenga, J., The minimal model program for the Hilbert scheme of points on and Bridgeland stability. Adv. Math. 235(2013), 580–626.http://dx.doi.Org/10.1016/j.aim.2O12.11.018 Google Scholar
[4] Artin, M., Algebraization of formal moduli. II. Existence of modifications. Ann. of Math. (2) 91(1970), 88–135.Google Scholar
[5] Castravet, A. M., The Cox ring of . Trans. Amer. Math. Soc. 361(2009), no. 7, 3851–3878.http://dx.doi.Org/10.1090/S0002-9947-09-04641-8 Google Scholar
[6] Castravet, A.-M. and Tevelev, J., is not a Mori dream space. Duke Math. J. 164(2015), no. 8, 1641–1667. http://dx.doi.org/10.1215/00127094-3119846 Google Scholar
[7] Chen, D., Mori's program for the Kontsevich moduli space . Int. Math. Res. Not. IMRN 2008, Art. ID rnn 067.http://dx.doi.Org/10.1093/imrn/rnn016 Google Scholar
[8] Chen, D. and Coskun, I., Stable base locus decompositions of Kontsevich moduli spaces. Michigan Math. J. 59(2010), no. 2, 435–466.http://dx.doi.org/10.1307/mmj71281531466 Google Scholar
[9] Chen, D., Towards Mori's program for the moduli space of stable maps. Amer. J. Math. 133(2011), no. 5,1389–1419.http://dx.doi.Org/10.1353/ajm.2O11.0040 Google Scholar
[10] Fedorchuk, M., The final log canonical model of the moduli space of stable curves of genus 4. Int. Math. Res. Not. IMRN 2012, no. 24, 5650–5672.Google Scholar
[11] Fedorchuk, M. and Smyth, D. I., Ample divisors on moduli spaces of pointed rational curves. J. Algebraic Geom. 20(2011), 599–629.http://dx.doi.org/10.1090/S1056-3911-2011-00547-X Google Scholar
[12] Fedorchuk, M., Stability of genus five canonical curves. In: A celebration of algebraic geometry, 18, Clay Math. Proa, American Mathematics Society, Providence, RI, 2013, pp. 281–310.Google Scholar
[13] Fulton, W., Intersection theory. Second ed., Ergebnisse der Mathematik und ihrer Grenzgebiete, 3, Folge. A Series of Modern Surveys in Mathematics Springer-Verlag, Berlin, 1998.Google Scholar
[14] Fulton, W. and MacPherson, R., A compactification of configuration spaces. Ann. of Math. (2) 139(1994), 183–225.http://dx.doi.org/10.2307/2946631 Google Scholar
[15] Giansiracusa, N., Conformai blocks and rational normal curves. J. Algebraic Geom. 22(2013), no. 4, 773–793.http://dx.doi.Org/10.1090/S1056-3911-2013-00601-3 Google Scholar
[16] Giansiracusa, N., Jensen, D., and Moon, H.-B., GIT compactifications of and flips. Adv. Math. 248(2013), 242–278.http://dx.doi.org/10.101 6/j.aim.2O13.08.011 Google Scholar
[17] Gibney, A., Jensen, D., Moon, H.-B., and Swinarski, D., Veronese quotient models of and conformai blocks. Michigan Math. J. 62(2013), no. 4, 721–751.http://dx.doi.Org/10.1307/mmj71387226162 Google Scholar
[18] González, J. L. and Karu, K., Some non-finitely generated cox rings. Compositio Mathematica, to appear. arxiv:1407.6344 Google Scholar
[19] Grothendieck, A., Élcments de géométrie algébrique.II. Étude globale élémentaire de quelques classes de morphismes, Inst. Hautes Études Sci. Publ. Math. 8(1961), 222.Google Scholar
[20] Grothendieck, A., Éléments de géométrie algébrique.IV. Étude locale des schémas et des morphismes de schémas. II. Inst. Hautes Études Sci. Publ. Math. 24(1965), 231.Google Scholar
[21] Grothendieck, A., Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas. III. Inst. Hautes Études Sci. Publ. Math. 28(1966), 255.Google Scholar
[22] Harris, J., emphAlgebraic geometry. A first course. Graduate Texts in Mathematics, 133, Springer-Verlag, New York, 1992.Google Scholar
[23] Hartshorne, R., Algebraic geometry. Graduate Texts in Mathematics, 52, Springer-Verlag, NewYork-Heidelberg, 1977.Google Scholar
[24] Hassett, B., Classical and minimal models of the moduli space of curves of genus two. In: Geometric methods in algebra and number theory, Progr. Math., 235, Birkhäuser Boston, Boston, MA, 2005, pp. 169–192.http://dx.doi.Org/10.1007/0-81 76-441 7-2_8 Google Scholar
[25] Hassett, B., Moduli spaces of weighted pointed stable curves. Adv. Math. 173(2003), 316–352.http://dx.doi.Org/10.1016/S0001-8708(02)00058-0 Google Scholar
[26] Hassett, B. and Hyeon, D., Log canonical models for the moduli space of curves: the first divisorial contraction. Trans. Amer. Math. Soc. 361(2009), no. 8, 4471–4489.http://dx.doi.Org/10.1090/S0002-9947-09-04819-3 Google Scholar
[27] Hassett, B., Log minimal model program for the moduli space of stable curves: the first flip. Ann. of Math.(2) 177(2013), no. 3, 911–968.http://dx.doi.Org/10.4007/annals.2013.177.3.3 Google Scholar
[28] Hu, Y., Relative geometric invariant theory and universal moduli spaces. Internat. J. Math. 7(1996), 151–181.http://dx.doi.Org/10.1142/S0129167X96000098 Google Scholar
[29] Hu, Y. and Keel, Sean, Mori dream spaces and GIT. Michigan Math. J. 48(2000), 331–348. http://dx.doi.org/10.1307/mmjV1030132722 Google Scholar
[30] Hyeon, D. and Lee, Y., Log minimal model program for the moduli space of stable curves of genus three. Math. Res. Lett. 17(2010), no. 4, 625–636.http://dx.doi.org/10.4310/MRL.2010.v17.n4.a4 Google Scholar
[31] Keel, S., Intersection theory of moduli space of stable n-pointed curves of genus zero. Trans. Amer. Math. Soc. 330(1992), no. 2, 545–574.http://dx.doi.org/10.2307/2153922 Google Scholar
[32] Keel, S. and McKernan, J., Contractible extremal rays on . In: Handbook of moduli II, Adv. Lect. Math. (ALM), 25, Int. Press, Somerville, MA, 2013.Google Scholar
[33] Kiem, Y.-H. and Moon, H.-B., Moduli spaces of weighted pointed stable rational curves via GIT. Osaka J. Math. 48 (2011), 1115–1140.Google Scholar
[34] Kim, B., Logarithmic stable maps. In: New developments in algebraic geometry, integrable systems and mirror symmetry (RIMS, Kyoto, 2008), Adv. Stud. Pure Math., 59, Math. Soc. Japan, Tokyo, 2010, pp. 167–200.Google Scholar
[35] Kim, B., Kresch, A., and Oh, Y.-G., A compactification of the space of maps from curves. Trans. Amer. Math. Soc. 366(2014), no. 1, 51–74.http://dx.doi.org/10.1090/S0002-9947-2013-05845-X Google Scholar
[36] Kirwan, F. C., Partial desingularisations of quotients of nonsingular varieties and their Betti numbers. Ann. of Math. (2) 122(1985), no. 1, 41–85.http://dx.doi.Org/10.2307/1971369 Google Scholar
[37] Knutson, D., Algebraic spaces, Lecture Notes in Mathematics, 203, Springer-Verlag, Berlin-New York, 1971.Google Scholar
[38] Kollár, J., Rational curves on algebraic varieties. Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge, 32, Springer-Verlag, Berlin, 1996. http://dx.doi.org/10.1007/978-3-662-03276-3 Google Scholar
[39] Kontsevich, M. and Manin, Y., Gromov-Witten classes, quantum cohomology, and enumerative geometry. Comm. Math. Phys. 164(1994), no. 3, 525–562.Google Scholar
[40] Lopez Martin, A., Virtual normalization and virtual fundamental classes. Proc. Amer. Math. Soc. 140(2012), 2235–2240.http://dx.doi.org/10.1090/S0002-9939-2011-11089-X Google Scholar
[41] Moon, H.-B., Log canonical models for the moduli space of stable pointed rational curves. Proc. Amer. Math. Soc. 141(2013), no. 11, 3771–3785.http://dx.doi.Org/10.1090/S0002-9939-2013-11674-6 Google Scholar
[42] Moon, H.-B., A family of divisors on and their log canonical models. J. Pure Appl. Algebra 219(2015), 4642–4652.http://dx.doi.Org/10.1016/j.jpaa.2015.02.036 Google Scholar
[43] Moon, H.-B.,Mori-s program for with symmetric divisors Math. Nachr. 288(2015), no. 7, 824–836.http://dx.doi.org/10.1002/mana.201300289 Google Scholar
[44] Mumford, D., The red book of varieties and schemes. Lecture Notes in Mathematics, 1358, Springer-Verlag, Berlin, 1999. http://dx.doi.Org/10.1007/b62130 Google Scholar
[45] Olsson, M. C., The logarithmic cotangent complex. Math. Ann. 333(2005), no. 4, 859–931.http://dx.doi.org/10.1007/s00208-005-0707-6 Google Scholar
[46] Pandharipande, R., The canonical class of (Pr,d) and enumerative geometry. Internat. Math. Res. Notices 1997, no. 4, 173–186.http://dx.doi.0rg/10.1155/S1073792897000123 Google Scholar
[47] Schubert, D., A new compactification of the moduli space of curves. Compositio Math. 78(1991), 297–313.Google Scholar
[48] Simpson, M., On log canonical models of the moduli space of stable pointed genus zero curves. Thesis (Ph.D.), Rice University, 2008 Google Scholar
[49] Smyth, D. I., Towards a classification of modular compactifications of Mg,n. Invent. Math. 192(2013), no. 12, 459–503.http://dx.doi.org/10.1007/s00222-012-0416-1 Google Scholar