Hostname: page-component-77c89778f8-gvh9x Total loading time: 0 Render date: 2024-07-19T13:47:24.406Z Has data issue: false hasContentIssue false
  • English
  • Français

Bivariate Polynomials of Least Deviation from Zero

Published online by Cambridge University Press:  20 November 2018

Borislav D. Bojanov ,
Werner Haußmann  and
Geno P. Nikolov
Borislav D. Bojanov
Affiliation:
Department of Mathematics University of Sofia Boul. James Bourchier 5 1164 Sofia Bulgaria, email: boris@fmi.uni-sofia.bg
Werner Haußmann
Affiliation:
Department of Mathematics Gerhard-Mercator-University Lotharstrasse 65 47048 Duisburg Germany, email: haussmann@math.uni-duisburg.de
Geno P. Nikolov
Affiliation:
Department of Mathematics University of Sofia Boul. James Bourchier 5 1164 Sofia Bulgaria, email: geno@fmi.uni-sofia.bg
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Bivariate polynomials with a fixed leading term 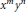${{x}^{m}}{{y}^{n}}$ , which deviate least from zero in the uniform or 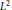${{L}^{2}}$-norm on the unit disk 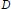$D$ (resp. a triangle) are given explicitly. A similar problem in 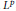${{L}^{p}}$ , 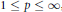$1\,\le \,p\,\le \,\infty $ is studied on 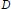$D$ in the set of products of linear polynomials.

Keywords

41A1041A5041A63
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 53 , Issue 3 , 01 June 2001 , pp. 489 - 505
Copyright
Copyright © Canadian Mathematical Society 2001

References

[1] Achieser, N. I., Vorlesungen über Approximationstheorie. Akademie-Verlag, Berlin, 1967.Google Scholar
[2] Bojanov, B., An extension of the Pizzetti formula for polyharmonic functions. Acta Math. Hungar., to appear.Google Scholar
[3] Bojanov, B. and Petrova, G., Numerical integration over a disc. A new Gaussian quadrature formula. Numer. Math. 80 (1998), 39–59.Google Scholar
[4] Borwein, P. and Erdélyi, T., Polynomials and Polynomial Inequalities. Springer, Berlin-Heidelberg-New York, 1995.Google Scholar
[5] Braß, H., Quadraturverfahren. Vandenhoeck und Ruprecht, Göttingen, 1977.Google Scholar
[6] Ehlich, H. and Zeller, K., Čebyšev–Polynome in mehreren Veränderlichen. Math. Z. 93 (1966), 142–143.Google Scholar
[7] Fromm, J., L1-approximation to zero. Math. Z. 151 (1976), 31–33.Google Scholar
[8] Gearhart, W. B., Some Chebyshev approximations by polynomials in two variables. J. Approx. Theory 8 (1973), 195–209.Google Scholar
[9] Haußmann, W. and Zeller, K., Blending interpolation and best L1-approximation. Arch. Math. (Basel) 40 (1983), 545–552.Google Scholar
[10] Haußmann, W. and Zeller, K., Mixed norm multivariate approximation with blending functions. In: Constructive Theory of Functions (eds. Bl. Sendov et al.), Publ. House Bulg. Acad. Sci., Sofia, 1984, 403–408.Google Scholar
[11] Kroó, A., Chebyshev-type extremal problems for multivariate polynomials. East J. Approx. 5 (1999), 211–221.Google Scholar
[12] Milovanović, G. V., Mitinović, D. S. and Rassias, T. M., Topics in Polynomials: Extremal Problems, Inequalities, Zeros. World Scientific Publ., River Edge, NJ, 1994.Google Scholar
[13] Newman, D. J. and Xu, Y., Tchebycheff polynomials on a triangular region. Constr. Approx. 9 (1993), 543–546.Google Scholar
[14] Oskolkov, K., Orthonormal systems of ridge- and radial polynomials. A construction based on symmetrization and Chebyshev type ideas. Lecture Notes, Univ. South Carolina, Columbia, 1996.Google Scholar
[15] Rack, H.-J., On multivariate polynomial L2-approximation to zero. Anal. Math. (Budapest) 10 (1984), 241–247.Google Scholar
[16] Rack, H.-J., On multivariate polynomial L1-approximation to zero and related coefficient inequalities. In: Multivariate Approximation Theory III (eds.W. Schempp et al.), Internat. Ser. Numer. Math. 75, Birkhäuser, Basel, 1985, 332–342.Google Scholar
[17] Reimer, M., On multivariate polynomials of least deviation from zero on the unit ball. Math. Z. 153 (1977), 51–58.Google Scholar
[18] Reimer, M., On multivariate polynomials of least deviation from zero on the unit cube. J. Approx. Theory 23 (1978), 65–69.Google Scholar
[19] Reimer, M., Constructive Theory of Functions. B. I. Wissenschaftsverlag, Mannheim-Wien-Zürich, 1990.Google Scholar
[20] Rivlin, T. J., The Chebyshev Polynomials. Wiley, New York-London-Sydney-Toronto, 1974.Google Scholar
[21] Šac, E., Certain polynomials of least deviation in the metric L2 . (Russian) Tallin. Polytehn. Inst. Toimetised Seer. A No. 293 (1970), 23–26.Google Scholar
[22] Sloss, J. M., Chebyshev approximation to zero. Pacific J. Math. 15 (1965), 305–313.Google Scholar