Article contents
An Elementary Proof of the Prime-Number Theorem for Arithmetic Progressions
Published online by Cambridge University Press: 20 November 2018
Extract
In this paper we shall give an elementary proof of the theorem
(1.1) 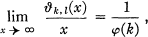
where φ(k) denotes Euler's function, and
(1.2) 
where p denotes the prime, and 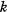 and
and  are integers with (
are integers with (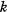 ,
, ) = 1,
) = 1, positive.
positive.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1950
References
1 “An Elementary Proof of the Prime-number Theorem,” Ann. of Math., vol. 50 (1949), 305–313.Google Scholar
2 “An Elementary Proof of Dirichlet's Theorem about Primes in an Arithmetic Progression,” Ann. of Math., vol. 50 (1949), 297–304.Google Scholar
3
We write ![]() instead of
instead of ![]() where no misunderstanding can occur.Google Scholar
where no misunderstanding can occur.Google Scholar
4 Instead of (2.8) we might use the somewhat sharper inequality

which can be proved in a similar way.
5 See for instance Dirichlet-Dedekind: Vorlesungen iiber Zahlentheorie (the beginning of §135)
6 For example, by showing that the number of terms with ![]() .
.
7 For example, by noting that a “period-parallelogram“ may always be chosen so that neither of its sides is greater than a diagonal.
8 Or, otherwise expressed, that the lattice may be built up of “period-parallelograms“ with both sides ![]() .
.
9 By residues, we understand here residues belonging to the reduced residue system.
10 By values we mean here residues mod ![]() .
.
11 For there is then a y in the interval with ![]() .
.
12 For there is then a y in the interval with ![]() .
.
- 33
- Cited by




