Hostname: page-component-77c89778f8-vsgnj Total loading time: 0 Render date: 2024-07-20T01:33:19.349Z Has data issue: false hasContentIssue false
  • English
  • Français

The interruption phenomenon for generalized continued fractions

Published online by Cambridge University Press:  17 April 2009

M.G. de Bruin
M.G. de Bruin
Affiliation:
Instituut voor Propedeutische Wiskunde, Universiteit van Amsterdam, Amsterdam, Netherlands.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

After defining a generalized C-fraction (a kind of Jacobi-Perron algorithm) for an n-tuple of formal power series over 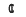(n ≥ 2), the connection between interruptions in the algorithm and linear dependence over 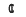[x] of the power series is studied.

Examples will be given showing that the algorithm behaves in a way similar to the Jacobi-Perron algorithm for an n-tuple of real numbers (the gcd-algorithm): there do exist n-tuples of formal power series f(1), f(2), …, f(n) with a C-n-fraction without interruptions but for which 1, f(1), f(2), …, f(n) is nevertheless linearly dependent over 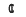[x].

Moreover an example will be given of algebraic functions f, g of degree n over 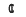[x] (formally defined) for which the C-n-fraction for f, f2, …, fn has just one interruption and that for g, g2, …, gn 1 none, while of course 1. f, f2, …, fn and 1, g, g2, …, gn admit (only) one dependence relation over 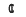[x].

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 19 , Issue 2 , October 1978 , pp. 245 - 272
Copyright
Copyright © Australian Mathematical Society 1979

References

[1]Bernstein, Leon, The Jacobi-Perron algorithm, its theory and application (Lecture Notes in Mathematics, 207. Springer-Verlag, Berlin, Heidelberg, Hew York, 1971).Google Scholar
[2]de Bruin, Marcelis Gerrit, “Generalized C-fractions and a multidimensional Padé table” (Diss. Universiteit van Amsterdam, Amsterdam, 1974).Google Scholar
[3]de Bruin, M.G., “Convergence along steplines in a generalized Padé table”, Padé and rational approximation: theory and applications, 15–22 (Proc. Internat. Sympos. University of South Florida, Tampa, 1976. Academic Press [Harcourt Brace Jovanovich], New York, San Francisco, London, 1977).CrossRefGoogle Scholar
[4]de Bruin, M.G., “Convergence of generalized C-fractions”, J. Approximation Theory (to appear).Google Scholar
[5]Dubois, Eugène, “Algorithme de Jacobi dans un corps de séries formelles” (Thèse, Université de Caen, France, 1970).Google Scholar
[6]Le Roux, Roger Paysant, “Périodicité de l'algorithme de Jacobi-Perron dans un corps de séries formelles et dans le corps des nombres réels” (Thèse, Université de Caen, France, 1970).Google Scholar
[7]Perron, Oskar, “Grundlagen für eine Theorie des Jacobischen Kettenbruchalgorithmus”, Math. Ann. 64 (1907), 1–76.Google Scholar
[8]Perron, Oskar, Die Lehre von den Kettenbrüchen, 3., verb, und erweiterte Auflage Band II (BG Teubner Verlag, Stuttgard, 1957).Google Scholar
[9]Schweiger, Fritz, The metrical theory of Jacobi-Perron algorithm (Lecture Notes in Mathematics, 334. Springer-Verlag, Berlin, Heidelberg, New York, 1973).Google Scholar
[10]Wall, H.S., Analytic theory of continued fractions (Van Nostrand, Toronto, New York, London, 1948).Google Scholar