Hostname: page-component-77c89778f8-7drxs Total loading time: 0 Render date: 2024-07-16T18:27:14.974Z Has data issue: false hasContentIssue false
  • English
  • Français

The Dirichlet problem for degenerate elliptic 2-dimensional Monge-Ampère equation

Published online by Cambridge University Press:  17 April 2009

Kazuo Amano
Kazuo Amano
Affiliation:
Centre for Mathematical Analysis, Australian National University, G.P.O. Box 4, Canberra, A.C.T. 2601, Australia
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

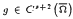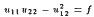We study the following Dirichlet problem for the degenerate elliptic Monge-Ampère equation: Given , f ≥ 0 and , find a solution , t ≥ 2, satisfying in Ω and u = g on ∂Ω. Since f is nonnegative, we cannot apply any standard elliptic methods. In this paper, we use an iteration scheme of Nash-Moser type and a priori estimates for degenerate elliptic operators, and solve the Dirichlet problem for a certain class of f and g.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 37 , Issue 3 , June 1988 , pp. 389 - 410
Copyright
Copyright © Australian Mathematical Society 1988

References

[1]Amano, K., ‘Maximum principles of degenerate elliptic-parabolic operators’, Indiana Univ. Math. J. 28 (1979), 545–557.CrossRefGoogle Scholar
[2]Amano, K., ‘The global hypoellipticity for degenerate elliptic-parabolic operators’, J. Math. Soc. Japan (to appear).Google Scholar
[3]Bakelman, I.J., ‘Generalized elliptic solutions of the Dirichlet problem for n-dimensional Mongo-Ampère equations’, part 1, Proc. Sympos. Pure Math. 45 (1986), 73–102.CrossRefGoogle Scholar
[4]Caffarelli, L., Nirenberg, L. and Spruck, J., ‘The Dirichlet problem for nonlinear second order elliptic equation I. Monge-Ampère equation’, Comm. Pure Appl. Math. 37 (1984), 369–402.CrossRefGoogle Scholar
[5]Chen, Y., ‘Degenerate Monge-Ampère equations’ (to appear).Google Scholar
[6]Gilbarg, D. and Trudinger, N.S., Elliptic partial differential equations of second order, 2nd edition (Springer-Verlag, Berlin, Heidelberg, New York, 1983).Google Scholar
[7]Krylov, N.V., ‘Boundedly nonhomogeneous elliptic and parabolic equations’, Math. USSR-Izv. 20 (1983), 459–492.CrossRefGoogle Scholar
[8]Krylov, N.V., ‘Boundedly nonhomogeneous elliptic and parabolic equations in a domain’, Math. USSR-Izv. 22 (1984), 67–97.CrossRefGoogle Scholar
[9]Krylov, N.V., ‘On degenerate nonlinear elliptic equations’, Math. USSR-Sb. 48 (1984), 307–326.CrossRefGoogle Scholar
[10]Lin, C.S., ‘The local isometric embedding in R 3 of 2-dimensional Riemannian manifolds with nonnegative curvature’, J. Differential Geom. 21 (1985), 213–230.CrossRefGoogle Scholar
[11]Lions, P.L., ‘Sur les èquations cle Monge-Ampère I”, Manuscripta Math. 41 (1983), 1–44.CrossRefGoogle Scholar
[12]Lions, P.L., ‘Sur les èquations de Monge-Ampère II’, Arch. Rational Mech. Anal 89 (1985), 93–122.CrossRefGoogle Scholar
[13]Oleinik, O.A. and Radkevich, E.V., Second order equations with nonnegative characteristic form (Amer. Math. Soc. Providence, Rhode Island and Plenum Press, 1973).CrossRefGoogle Scholar
[14]Rauch, J. and Taylor, B.A., ‘The Dirichlet problem for the multidimensional Monge-Ampère equations’, Rocky Mountain J. Math. 7 (1977), 345–364.CrossRefGoogle Scholar
[15]Trudinger, N.S., ‘On degenerate fully nonlinear elliptic equation in balls’, Bull. Austral. Math. Soc. 35 (1987), 299–307.CrossRefGoogle Scholar
[16]Urbas, J.I.E., Elliptic equations of Monge-Ampère type (Thesis, Australian National University, 1984).Google Scholar