No CrossRef data available.
Article contents
A NOTE ON THE NUMBER OF SOLUTIONS OF TERNARY PURELY EXPONENTIAL DIOPHANTINE EQUATIONS
Published online by Cambridge University Press: 10 June 2022
Abstract
Let a, b, c be fixed coprime positive integers with
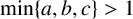 $\min \{a,b,c\}>1$
. We discuss the conjecture that the equation
$\min \{a,b,c\}>1$
. We discuss the conjecture that the equation
 $a^{x}+b^{y}=c^{z}$
has at most one positive integer solution
$a^{x}+b^{y}=c^{z}$
has at most one positive integer solution
 $(x,y,z)$
with
$(x,y,z)$
with
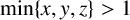 $\min \{x,y,z\}>1$
, which is far from solved. For any odd positive integer r with
$\min \{x,y,z\}>1$
, which is far from solved. For any odd positive integer r with
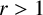 $r>1$
, let
$r>1$
, let
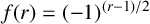 $f(r)=(-1)^{(r-1)/2}$
and
$f(r)=(-1)^{(r-1)/2}$
and
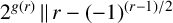 $2^{g(r)}\,\|\, r-(-1)^{(r-1)/2}$
. We prove that if one of the following conditions is satisfied, then the conjecture is true: (i)
$2^{g(r)}\,\|\, r-(-1)^{(r-1)/2}$
. We prove that if one of the following conditions is satisfied, then the conjecture is true: (i)
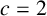 $c=2$
; (ii) a, b and c are distinct primes; (iii)
$c=2$
; (ii) a, b and c are distinct primes; (iii)
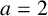 $a=2$
and either
$a=2$
and either
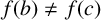 $f(b)\ne f(c)$
, or
$f(b)\ne f(c)$
, or
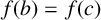 $f(b)=f(c)$
and
$f(b)=f(c)$
and
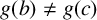 $g(b)\ne g(c)$
.
$g(b)\ne g(c)$
.
Keywords
MSC classification
- Type
- Research Article
- Information
- Bulletin of the Australian Mathematical Society , Volume 107 , Issue 1 , February 2023 , pp. 53 - 65
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
The third author is supported by JSPS KAKENHI Grant Number 18K03247.





