We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper we study the zero sets of harmonic functions on open sets in 
${{\mathbb{R}}^{N}}$ and holomorphic functions on open sets in 
${{\mathbb{C}}^{N}}$. We show that the non-extendability of such zero sets is a generic phenomenon.
Let (M,I,J,K) be a compact hyperkähler manifold,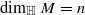 
, and L a non-trivial holomorphic line bundle on (M,I). Using the quaternionic Dolbeault complex, we prove the following vanishing theorem for holomorphic cohomology of L. If c1(L) lies in the closure 
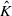of the dual Kähler cone, then Hi(L)=0 for i>n. If c1(L) lies in the opposite cone 
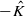, then Hi(L)=0 for i<n. Finally, if c1(L) is neither in 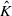nor in 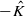, then Hi(L)=0 for 
.
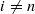