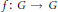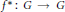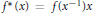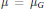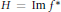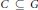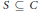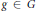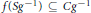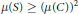1 results
Transformations and Colorings of Groups
-
- Journal:
- Canadian Mathematical Bulletin / Volume 50 / Issue 4 / 01 December 2007
- Published online by Cambridge University Press:
- 20 November 2018, pp. 632-636
- Print publication:
- 01 December 2007
-
- Article
-
- You have access
- Export citation
-
Let
$G$ be a compact topological group and let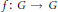 
$f:\,G\,\to \,G$ be a continuous transformation of $G$. Define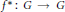 
${{f}^{*}}:G\to G$ by 
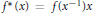${{f}^{*}}\left( x \right)\,=\,f\left( {{x}^{-1}} \right)x$ and let 
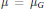$\mu \,=\,{{\mu }_{G}}$ be Haar measure on $G$. Assume that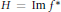 
$H\,=\,\text{IM}\,{{f}^{*}}$ is a subgroup of $G$ and for every measurable 
$C\,\subseteq \,H,\,{{\mu }_{G}}{{\left( \left( {{f}^{*}} \right) \right)}^{-1}}\left( \left( C \right) \right)\,=\,\mu H\left( C \right)$. Then for every measurable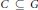 
$C\,\subseteq \,G$, there exist 
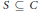$S\subseteq C$ and 
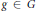$g\,\in \,G$ such that 
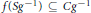$f\left( S{{g}^{-1}} \right)\,\subseteq \,C{{g}^{-1}}$ and 
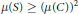$\mu \left( S \right)\,\ge \,{{\left( \mu \left( C \right) \right)}^{2}}$.
