1. Introduction
Granular mixtures of particles that differ in size or density tend to demix into striking segregation patterns (e.g. radial segregation in rotating drums (Hill, Gioia & Amaravadi Reference Hill, Gioia and Amaravadi2004; Gray & Ancey Reference Gray and Ancey2011), granular fingering (Pouliquen, Delour & Savage Reference Pouliquen, Delour and Savage1997) or self-induced Rayleigh–Taylor instability (D'Ortona & Thomas Reference D'Ortona and Thomas2020)). Various factors influence the segregation patterns, including gravity, gradients of concentration and granular temperature, and secondary flows. Among these, segregation attributed purely to gravity has been studied the most (Khakhar, McCarthy & Ottino Reference Khakhar, McCarthy and Ottino1997; Savage & Lun Reference Savage and Lun1988; Gray & Thornton Reference Gray and Thornton2005); however, the role played by gradients in the granular temperature is beginning to be understood (Arnarson & Jenkins Reference Arnarson and Jenkins2004; Larcher & Jenkins Reference Larcher and Jenkins2013, Reference Larcher and Jenkins2015); with spheres of two sizes made of the same material, the larger migrate towards regions of lower granular temperature; for spheres of the same size made of different materials, the more massive move downward under gravity. Slow and dense gravitational flows of poly-sized granular mixture have earned serious attention. In many of these flows, larger particles migrate at the surface of the flow while smaller ones percolate to the bottom. The dominant mechanisms driving this segregation process is commonly identified as kinetic sieving and squeeze expulsion (Savage & Lun Reference Savage and Lun1988).
Recently, Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) highlighted the existence of highly inhomogeneous flows on steep slopes, named ‘supported flows’ (Taberlet et al. Reference Taberlet, Richard, Jenkins and Delannay2007) and characterized by a dense core floating over a dilute and highly agitated layer of grains. We present here discrete numerical simulations that reveal a new segregation pattern emerging in high-speed granular flows, in which large particles are trapped within the flow in the dense core. This size segregation is driven by granular temperature gradients rather than gravity and can be described by the kinetic theory for binary mixtures. The granular temperature is a measure of the energy of the velocity fluctuations of the grains. Interestingly, this segregating behaviour promotes the transport capability of the flow of binary mixtures, which can be one of the mechanisms involved in long run-out avalanches observed in nature. A flow enhancement due simply to the presence of spheres of two sizes is also predicted by kinetic theory (Larcher & Jenkins Reference Larcher and Jenkins2019) for particle-fluid flows.
2. Simulation methodology
To unravel the role of complex secondary flows on the segregation process of a granular mixture of spheres, numerical simulations using the discrete element method (DEM) have been performed. The details of the method can be found in Brodu, Richard & Delannay (Reference Brodu, Richard and Delannay2013). We recall here briefly the main ingredients. The simulation cell is composed of smooth, flat and frictional bottom and parallel sidewalls (see figure 1), in a similar way that is usually done experimentally (Louge & Keast Reference Louge and Keast2001; Richard et al. Reference Richard, Valance, Métayer, Sanchez, Crassous, Louge and Delannay2008). The simulation cell width ![]() $W$ is chosen to be
$W$ is chosen to be ![]() $W=40D$, with
$W=40D$, with ![]() $D$ a unit of length, and periodic boundary conditions are applied in the streamwise direction with a periodic length
$D$ a unit of length, and periodic boundary conditions are applied in the streamwise direction with a periodic length ![]() $L=20D$. The cell is tilted by an angle
$L=20D$. The cell is tilted by an angle ![]() $\theta$ to produce flows. Particles are subject to gravity and interact through frictional visco-elastic contacts with other particles. These interactions employ a linear visco-elastic model with normal and tangential coefficients of restitution
$\theta$ to produce flows. Particles are subject to gravity and interact through frictional visco-elastic contacts with other particles. These interactions employ a linear visco-elastic model with normal and tangential coefficients of restitution ![]() $e_{n}^{gg}=0.972$ and
$e_{n}^{gg}=0.972$ and ![]() $e_{t}^{gg}=0.25$, respectively. The normal spring stiffness is chosen as
$e_{t}^{gg}=0.25$, respectively. The normal spring stiffness is chosen as ![]() $k_{n}^{gg}=2\times 10^{5}m^\ast g/D$, with
$k_{n}^{gg}=2\times 10^{5}m^\ast g/D$, with ![]() $m^\ast$ and
$m^\ast$ and ![]() $g$ being mass and acceleration units, respectively; the tangential spring stiffness is computed from the relation provided by Brodu et al. (Reference Brodu, Richard and Delannay2013):
$g$ being mass and acceleration units, respectively; the tangential spring stiffness is computed from the relation provided by Brodu et al. (Reference Brodu, Richard and Delannay2013): ![]() $7k_{t}^{gg}({\rm \pi} ^{2}+[\ln (e_{n}^{gg})]^{2})=2k_{n}^{gg}({\rm \pi} ^{2}+[\ln (e_{t}^{gg})]^{2})$. The tangential force is bounded according to a Coulomb friction model with friction coefficient
$7k_{t}^{gg}({\rm \pi} ^{2}+[\ln (e_{n}^{gg})]^{2})=2k_{n}^{gg}({\rm \pi} ^{2}+[\ln (e_{t}^{gg})]^{2})$. The tangential force is bounded according to a Coulomb friction model with friction coefficient ![]() $\mu ^{gg}$. The same set of parameters is used for particles/wall contacts:
$\mu ^{gg}$. The same set of parameters is used for particles/wall contacts: ![]() $e_{n}^{gw}=0.8$,
$e_{n}^{gw}=0.8$, ![]() $e_{t}^{gw}=0.35$,
$e_{t}^{gw}=0.35$, ![]() $k_{n}^{gw}=2\times 10^{5}$,
$k_{n}^{gw}=2\times 10^{5}$, ![]() $\mu ^{gw}=0.593$. These parameter values are taken from Louge & Keast (Reference Louge and Keast2001) and correspond to glass beads and aluminum walls to match materials usually used in experiments.
$\mu ^{gw}=0.593$. These parameter values are taken from Louge & Keast (Reference Louge and Keast2001) and correspond to glass beads and aluminum walls to match materials usually used in experiments.

Figure 1. Scheme of the simulation cell of width ![]() $W$ and length
$W$ and length ![]() $L$. The flow is bounded by infinitely high (in
$L$. The flow is bounded by infinitely high (in ![]() $z$ direction) flat walls in the transverse direction
$z$ direction) flat walls in the transverse direction ![]() $y$, and periodic boundary conditions are applied in the stream direction
$y$, and periodic boundary conditions are applied in the stream direction ![]() $x$. The box is inclined by an angle
$x$. The box is inclined by an angle ![]() $\theta$.
$\theta$.
A time step of ![]() $2\times 10^{-5}\sqrt {(D/g)}$ was chosen as a good compromise between numerical stability requirements and reasonable simulation duration. The total mass in the system is controlled by the mass hold-up, which basically represents the height of the grain assembly when densely packed and is defined by
$2\times 10^{-5}\sqrt {(D/g)}$ was chosen as a good compromise between numerical stability requirements and reasonable simulation duration. The total mass in the system is controlled by the mass hold-up, which basically represents the height of the grain assembly when densely packed and is defined by ![]() $H=\sum _{i} m_{i}/(\rho ^\ast LW)$, with
$H=\sum _{i} m_{i}/(\rho ^\ast LW)$, with ![]() $\rho ^\ast =6m^\ast /{\rm \pi} D^{3}$, the reference material density, and
$\rho ^\ast =6m^\ast /{\rm \pi} D^{3}$, the reference material density, and ![]() $m_{i}$, the mass of the grain
$m_{i}$, the mass of the grain ![]() $i$. All the simulation outcomes have been obtained with a mass hold-up
$i$. All the simulation outcomes have been obtained with a mass hold-up ![]() $H=6D$. Binary mixtures have been used. The two grain species have the same material density
$H=6D$. Binary mixtures have been used. The two grain species have the same material density ![]() $\rho ^\ast$, but differ in size, with a mean radii
$\rho ^\ast$, but differ in size, with a mean radii ![]() $r_S$ and
$r_S$ and ![]() $r_L$, respectively, for the small and large. The radii of each size are uniformly distributed by
$r_L$, respectively, for the small and large. The radii of each size are uniformly distributed by ![]() $8\,\%$ around the mean value. The radius of the ‘mixture sphere’ is defined as the average of both radii,
$8\,\%$ around the mean value. The radius of the ‘mixture sphere’ is defined as the average of both radii, ![]() $r=(r_S+r_L)/2$. The grains have masses
$r=(r_S+r_L)/2$. The grains have masses ![]() $m_S$ and
$m_S$ and ![]() $m_L$, with
$m_L$, with ![]() $m= m_S + m_L$, number densities,
$m= m_S + m_L$, number densities, ![]() $n_S$ and
$n_S$ and ![]() $n_L$, with
$n_L$, with ![]() $n=n_S + n_L$, and mass densities,
$n=n_S + n_L$, and mass densities, ![]() $\rho _S=m_S n_S$ and
$\rho _S=m_S n_S$ and ![]() $\rho _L=m_L n_L$, with
$\rho _L=m_L n_L$, with ![]() $\rho =\rho _S+\rho _L$. We define volume concentrations for the small and large grains,
$\rho =\rho _S+\rho _L$. We define volume concentrations for the small and large grains, ![]() $c_S=4{\rm \pi} n_S r_S^3/3$ and
$c_S=4{\rm \pi} n_S r_S^3/3$ and ![]() $c_L=4{\rm \pi} n_L r_L^3/3$, and for the mixture
$c_L=4{\rm \pi} n_L r_L^3/3$, and for the mixture ![]() $c=c_S+c_L$, and we denote the volume concentration fraction of the small particles in the mixture as
$c=c_S+c_L$, and we denote the volume concentration fraction of the small particles in the mixture as ![]() $\varPhi _S=c_S/c$, which is equivalent to the mass concentration fraction for grains made of the same material. The granular temperature
$\varPhi _S=c_S/c$, which is equivalent to the mass concentration fraction for grains made of the same material. The granular temperature ![]() $T$ of the mixture is defined as
$T$ of the mixture is defined as ![]() $T=(n_S T_S + n_L T_L)/n$ where
$T=(n_S T_S + n_L T_L)/n$ where ![]() $T_i=(1/2)m_i<(\boldsymbol {v}_i-\boldsymbol {V})\boldsymbol {\cdot } (\boldsymbol {v}_i-\boldsymbol {V})>$ with
$T_i=(1/2)m_i<(\boldsymbol {v}_i-\boldsymbol {V})\boldsymbol {\cdot } (\boldsymbol {v}_i-\boldsymbol {V})>$ with ![]() $i={S,L}$ (
$i={S,L}$ (![]() $\boldsymbol {v}_i$ is the particle velocity of species
$\boldsymbol {v}_i$ is the particle velocity of species ![]() $i$ and
$i$ and ![]() $\boldsymbol {V}$ is the barycentric velocity of the mixture).
$\boldsymbol {V}$ is the barycentric velocity of the mixture).
Most of the simulation outcomes were obtained for binary mixtures with a radii ratio ![]() $r_L/r_S=2$ and a mixture diameter
$r_L/r_S=2$ and a mixture diameter ![]() $2r=1.125 D$ (i.e.
$2r=1.125 D$ (i.e. ![]() $2r_S=0.75 D$ and
$2r_S=0.75 D$ and ![]() $2r_S=1.5 D$) and various volume concentration fraction
$2r_S=1.5 D$) and various volume concentration fraction ![]() $\varPhi _S$ of small particles ranging from
$\varPhi _S$ of small particles ranging from ![]() $0$ to
$0$ to ![]() $100\,\%$. For the comparison with the kinetic theory, we also employed mixtures with a smaller radii ratio
$100\,\%$. For the comparison with the kinetic theory, we also employed mixtures with a smaller radii ratio ![]() $r_L/r_S=1.35$ and a mixture diameter
$r_L/r_S=1.35$ and a mixture diameter ![]() $2r=1 D$ (i.e.
$2r=1 D$ (i.e. ![]() $2r_S=0.85 D$ and
$2r_S=0.85 D$ and ![]() $2r_L=1.15D$). The particles of the mixture were initially displayed on a regular lattice but randomly distributed between small and large according to the prescribed volume concentration fraction. The assembly of particles is dropped at a small altitude from the bottom of the cell with a finite but small streamwise velocity
$2r_L=1.15D$). The particles of the mixture were initially displayed on a regular lattice but randomly distributed between small and large according to the prescribed volume concentration fraction. The assembly of particles is dropped at a small altitude from the bottom of the cell with a finite but small streamwise velocity ![]() $V_x=5\sqrt {gD}$. After a transient which never exceeds
$V_x=5\sqrt {gD}$. After a transient which never exceeds ![]() $1000\sqrt {(D/g)}$ time units, for the set of parameters investigated so far, the flow reaches a steady state in which flow kinematic and segregation patterns remain stationary (i.e. relative variations are less than
$1000\sqrt {(D/g)}$ time units, for the set of parameters investigated so far, the flow reaches a steady state in which flow kinematic and segregation patterns remain stationary (i.e. relative variations are less than ![]() $1\,\%$). We explored a large range of inclination angles (from
$1\,\%$). We explored a large range of inclination angles (from ![]() $19^{\circ }$ to
$19^{\circ }$ to ![]() $50^{\circ }$) for various mass concentration fractions of the mixture and the flow always achieved a steady state. The coarse-grained fields (volume fraction, velocity and granular temperature) are computed following Serero et al. (Reference Serero, Goldenberg, Noskowicz and Goldhirsch2008) with a Lucy polynomial for the weighting function as proposed by Weinhart et al. (Reference Weinhart, Thornton, Luding and Bokhove2012). The window size of the coarse-graining procedure is twice the mean particle diameter, i.e.
$50^{\circ }$) for various mass concentration fractions of the mixture and the flow always achieved a steady state. The coarse-grained fields (volume fraction, velocity and granular temperature) are computed following Serero et al. (Reference Serero, Goldenberg, Noskowicz and Goldhirsch2008) with a Lucy polynomial for the weighting function as proposed by Weinhart et al. (Reference Weinhart, Thornton, Luding and Bokhove2012). The window size of the coarse-graining procedure is twice the mean particle diameter, i.e. ![]() $2(r_L+r_S)$. The field values are spatially averaged along the streamwise direction and time averaged over
$2(r_L+r_S)$. The field values are spatially averaged along the streamwise direction and time averaged over ![]() $200$ time units, once the flow reached its steady state.
$200$ time units, once the flow reached its steady state.
3. Supported flows and segregation patterns
As a start, we describe briefly the main features of the flow regimes obtained for monodisperse particles with diameter ![]() $2r=1D\pm 0.08D$. Packing fraction and longitudinal velocity maps of the flow cross-section for three different inclination angles are presented in figure 2. For a low inclination angle (
$2r=1D\pm 0.08D$. Packing fraction and longitudinal velocity maps of the flow cross-section for three different inclination angles are presented in figure 2. For a low inclination angle (![]() $\theta =19^{\circ }$), a dense unidirectional flow is observed with grains arranged in horizontal layers. At an intermediate angle (
$\theta =19^{\circ }$), a dense unidirectional flow is observed with grains arranged in horizontal layers. At an intermediate angle (![]() $\theta =25^{\circ })$, the layering is broken and a denser region emerged within the flow accompanied with a pair of counter-rotating longitudinal rolls. One can note a slight concavity of the free surface with a trough in the centre of the cell. At a higher inclination angle (
$\theta =25^{\circ })$, the layering is broken and a denser region emerged within the flow accompanied with a pair of counter-rotating longitudinal rolls. One can note a slight concavity of the free surface with a trough in the centre of the cell. At a higher inclination angle (![]() $\theta =35^{\circ }$), a supported state develops. The latter is characterized by the presence of a very dense core surrounded by a dilute layer of highly agitated grains. This scenario that leads to the emergence of supported flows was already evidenced by Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for wider channels (
$\theta =35^{\circ }$), a supported state develops. The latter is characterized by the presence of a very dense core surrounded by a dilute layer of highly agitated grains. This scenario that leads to the emergence of supported flows was already evidenced by Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for wider channels (![]() $W=68D)$ and is also observed with smaller gap widths (Zhu, Delannay & Valance Reference Zhu, Delannay and Valance2021). For
$W=68D)$ and is also observed with smaller gap widths (Zhu, Delannay & Valance Reference Zhu, Delannay and Valance2021). For ![]() $W=40D$, the transition towards supported flows occurs at a critical inclination angle
$W=40D$, the transition towards supported flows occurs at a critical inclination angle ![]() $\theta _c=27^\circ$.
$\theta _c=27^\circ$.

Figure 2. Monodisperse flows (![]() $2r=1D$) for
$2r=1D$) for ![]() $H=6D$ and
$H=6D$ and ![]() $W=40D$: (a) Packing fraction maps together with transverse velocity (black lines) for a dense flow (
$W=40D$: (a) Packing fraction maps together with transverse velocity (black lines) for a dense flow (![]() $\theta =19^{\circ }$), an intermediate flow (
$\theta =19^{\circ }$), an intermediate flow (![]() $\theta =25^{\circ }$) and a supported flow (
$\theta =25^{\circ }$) and a supported flow (![]() $\theta =35^{\circ }$), as already evidenced in Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for a wider channel. (b) Corresponding longitudinal velocities.
$\theta =35^{\circ }$), as already evidenced in Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for a wider channel. (b) Corresponding longitudinal velocities.
We first investigated the segregation patterns obtained for a binary mixture with equal mass (i.e. equivalently, equal volume) of small and large particles (![]() $\varPhi _S=0.5$) and with a size ratio equal to
$\varPhi _S=0.5$) and with a size ratio equal to ![]() $2$ (i.e.
$2$ (i.e. ![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75$). Figure 3 shows the relative local concentration of large particles
$2r_S=0.75$). Figure 3 shows the relative local concentration of large particles ![]() $c_L/c$ within the flow cross-section for three inclination angles (
$c_L/c$ within the flow cross-section for three inclination angles (![]() $19^{\circ }$,
$19^{\circ }$, ![]() $25^{\circ }$ and
$25^{\circ }$ and ![]() $35^{\circ }$), together with streamlines to visualize the longitudinal rolls. For an inclination of
$35^{\circ }$), together with streamlines to visualize the longitudinal rolls. For an inclination of ![]() $19^{\circ }$, the flow is unidirectional and uniformly sheared and a classical reverse grading is observed, with large particles at the top of the flow, while small particles have percolated down to the bottom.
$19^{\circ }$, the flow is unidirectional and uniformly sheared and a classical reverse grading is observed, with large particles at the top of the flow, while small particles have percolated down to the bottom.

Figure 3. (a) Bidisperse flows (![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75D$): relative concentration of large particles
$2r_S=0.75D$): relative concentration of large particles ![]() $c_L/c$ in the flow cross-section vs inclination angles for a mass fraction of small particles
$c_L/c$ in the flow cross-section vs inclination angles for a mass fraction of small particles ![]() $\varPhi _S=0.5$. Streamlines are plotted to highlight the presence of convection rolls (black lines). (b) Corresponding maps of the granular temperature
$\varPhi _S=0.5$. Streamlines are plotted to highlight the presence of convection rolls (black lines). (b) Corresponding maps of the granular temperature ![]() $T$ of the mixture. (c,d) Vertical profiles of the relative volume fraction of the large particle,
$T$ of the mixture. (c,d) Vertical profiles of the relative volume fraction of the large particle, ![]() $c_L/c$, and of the granular temperature, computed at
$c_L/c$, and of the granular temperature, computed at ![]() $y=10D$.
$y=10D$.
Upon increasing the inclination angle (i.e. ![]() $\theta \approx 25^\circ$), a pair of longitudinal rolls develops within the flow. The convection tends to mix the granular systems because large particles are incorporated in the bulk flow. A transverse segregation also clearly appears: there is a depletion of large particles between the two longitudinal rolls. At higher inclination (i.e.
$\theta \approx 25^\circ$), a pair of longitudinal rolls develops within the flow. The convection tends to mix the granular systems because large particles are incorporated in the bulk flow. A transverse segregation also clearly appears: there is a depletion of large particles between the two longitudinal rolls. At higher inclination (i.e. ![]() $\theta =30^\circ$), as in the monodisperse case, a supported flow regime emerges with a dense core ‘floating’ over a highly agitated and dilute layer. Despite the formation of the dense core, convection rolls are still present. Surprisingly, the setting of this supported state leads to the emergence of a new segregation pattern in which the large particles no longer reside at the surface of the flow but are trapped within the dense, cooler core, as clearly evidenced for
$\theta =30^\circ$), as in the monodisperse case, a supported flow regime emerges with a dense core ‘floating’ over a highly agitated and dilute layer. Despite the formation of the dense core, convection rolls are still present. Surprisingly, the setting of this supported state leads to the emergence of a new segregation pattern in which the large particles no longer reside at the surface of the flow but are trapped within the dense, cooler core, as clearly evidenced for ![]() $\theta =35^\circ$. More precisely, two spots of high concentration of large grains are located at the centre of the longitudinal rolls, surrounded by a mixed layer of large and small particles. Pure layers of small particles are present close to the bottom and lateral walls, and the upper part of the flows consists essentially of small particles. This segregated state is not a transient but a mature and steady state. An intermediate segregation pattern is seen for an angle of
$\theta =35^\circ$. More precisely, two spots of high concentration of large grains are located at the centre of the longitudinal rolls, surrounded by a mixed layer of large and small particles. Pure layers of small particles are present close to the bottom and lateral walls, and the upper part of the flows consists essentially of small particles. This segregated state is not a transient but a mature and steady state. An intermediate segregation pattern is seen for an angle of ![]() $25^{\circ }$, for which the flow is not yet in a supported regime.
$25^{\circ }$, for which the flow is not yet in a supported regime.
Figure 4 shows the vertical position of the centre of mass of the mixture together with that of the large and small particles, respectively, vs the tangent of the inclination angle ![]() $\theta$. Interestingly, an inversion in the vertical position of the centre of mass of the large and small particles clearly appears between
$\theta$. Interestingly, an inversion in the vertical position of the centre of mass of the large and small particles clearly appears between ![]() $25^{\circ }$ and
$25^{\circ }$ and ![]() $30^{\circ }$, which corresponds to the range of angles in which supported flows develop for the set of parameters investigated. This unusual segregation pattern appears to be a direct consequence of the establishment of the supported flow. Furthermore, the linear relation between the centre of mass and the tangent of the angle of inclination, derived by Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for supported flows (
$30^{\circ }$, which corresponds to the range of angles in which supported flows develop for the set of parameters investigated. This unusual segregation pattern appears to be a direct consequence of the establishment of the supported flow. Furthermore, the linear relation between the centre of mass and the tangent of the angle of inclination, derived by Brodu et al. (Reference Brodu, Delannay, Valance and Richard2015) for supported flows (![]() $\theta >27^{\circ }$), is also seen for a binary mixture and is robust to change of mixture composition.
$\theta >27^{\circ }$), is also seen for a binary mixture and is robust to change of mixture composition.

Figure 4. Vertical position of the centre of mass of the mixture (solid line green triangle), large particles (dashed line red diamond) and small particles (dashed line blue dots) vs the inclination angle ![]() $\theta$.
$\theta$. ![]() $50{:}50$ mixture by mass with
$50{:}50$ mixture by mass with ![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75D$.
$2r_S=0.75D$.
4. Influence of the mixture concentration
The influence of the relative concentration of small particles ![]() $\varPhi _S$ within the mixture was explored. We kept the same radii ratio of 2 as for previous simulations (i.e.
$\varPhi _S$ within the mixture was explored. We kept the same radii ratio of 2 as for previous simulations (i.e. ![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75D$). Profiles of streamwise velocity
$2r_S=0.75D$). Profiles of streamwise velocity ![]() $V_{x}$ are presented in figure 5(a) for a low inclination angle, where dense flows are expected. The two limit cases (i.e.
$V_{x}$ are presented in figure 5(a) for a low inclination angle, where dense flows are expected. The two limit cases (i.e. ![]() $\varPhi _s=0$ and
$\varPhi _s=0$ and ![]() $1$) correspond to monodisperse flows with particles of diameter
$1$) correspond to monodisperse flows with particles of diameter ![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75D$, respectively. The vertical velocity profiles corresponding to these monodisperse flows indicate that the flow is uniformly sheared, with an ordering of grains in horizontal layers (Jenkins & Larcher Reference Jenkins and Larcher2017). The monosize flow with small particles is faster than that with large particles, as predicted by Jenkins & Larcher (Reference Jenkins and Larcher2017) for unconfined layered shear flows. In addition, decreasing the particle size while maintaining the width of the channel constant leads to a decrease of the lateral confinement exerted by the walls, resulting in an increase of the mean flow velocity. Theses two limit cases provide bounds for the comparison with binary mixtures.
$2r_S=0.75D$, respectively. The vertical velocity profiles corresponding to these monodisperse flows indicate that the flow is uniformly sheared, with an ordering of grains in horizontal layers (Jenkins & Larcher Reference Jenkins and Larcher2017). The monosize flow with small particles is faster than that with large particles, as predicted by Jenkins & Larcher (Reference Jenkins and Larcher2017) for unconfined layered shear flows. In addition, decreasing the particle size while maintaining the width of the channel constant leads to a decrease of the lateral confinement exerted by the walls, resulting in an increase of the mean flow velocity. Theses two limit cases provide bounds for the comparison with binary mixtures.

Figure 5. Vertical profiles of the streamwise velocity (a,b) and mixture concentration ![]() $c$ (c,d) for binary mixtures with relative volume concentration of small particles
$c$ (c,d) for binary mixtures with relative volume concentration of small particles ![]() $\varPhi _S$ varying from
$\varPhi _S$ varying from ![]() $0$ to
$0$ to ![]() $1$: (a,c) dense regimes
$1$: (a,c) dense regimes ![]() $\theta =20{^\circ }$, (b,d) supported regime
$\theta =20{^\circ }$, (b,d) supported regime ![]() $\theta =35^{\circ }$. Binary mixtures with
$\theta =35^{\circ }$. Binary mixtures with ![]() $2r_L=1.5D$ and
$2r_L=1.5D$ and ![]() $2r_S=0.75D$.
$2r_S=0.75D$.
Dense flow regimes of binary mixtures exhibit contrasting features. At any finite concentration investigated so far, the velocity profile looks like a plug flow with almost no shearing but a finite slip at the bottom. This is a consequence of the influence of the lateral boundaries. Note also that a weak concentration of small particles in the mixture (![]() $\varPhi _S=0.05$ and
$\varPhi _S=0.05$ and ![]() $0.1$) leads to a drastic reduction of the flow velocity in comparison with monodisperse flows of large particles. Mixture seems thus to prevent the formation of ordered layers, which slow the flow.
$0.1$) leads to a drastic reduction of the flow velocity in comparison with monodisperse flows of large particles. Mixture seems thus to prevent the formation of ordered layers, which slow the flow.
A completely different behaviour is observed for rapid flows, for which the supported regime is expected. Indeed, the slowdown for binary mixtures observed for dense flow regimes no longer exists and the streamwise velocity of mixtures is always higher than for monodisperse flows of large particles (figure 5). Surprisingly, there is an optimal concentration of small particles (approximately ![]() $\varPhi _S=0.4$, see figure 5) that produces flows with velocities higher than the expected most favourable case, corresponding to a monodisperse flow of small particles. This is also seen in the flow of mixtures of a fluid and a binary mixtures of spheres above a particle bed (Larcher & Jenkins Reference Larcher and Jenkins2019).
$\varPhi _S=0.4$, see figure 5) that produces flows with velocities higher than the expected most favourable case, corresponding to a monodisperse flow of small particles. This is also seen in the flow of mixtures of a fluid and a binary mixtures of spheres above a particle bed (Larcher & Jenkins Reference Larcher and Jenkins2019).
The mass flow rate, which indicates the capacity of the flow to transport matter, is also highly influenced by the nature of the flow regime (dense vs supported). The variation of the mass flow rate as a function of the mixture concentration is reported in figure 6 for the dense and supported regimes, respectively. In the dense regime, the mass flow rate exhibits a plateau for mixture concentration ![]() $\varPhi _S$ between
$\varPhi _S$ between ![]() $0.2$ and
$0.2$ and ![]() $0.8$. In this range of concentration, the mass flow rate is independent of the mixture concentration. This is simply a consequence of the invariance of the flow profiles in this concentration regime (cf. figure 5). For small concentrations of the mixture, there is, in contrast, a drop of the mass flow rate which is mainly due to a decrease of the mass flow rate of the large particles. This drop is closely related to the decrease observed in the flow velocity profile for small mixture concentrations. The large concentration limit is also singular with an enhancement of the mass flow rate in comparison with the value of the plateau.
$0.8$. In this range of concentration, the mass flow rate is independent of the mixture concentration. This is simply a consequence of the invariance of the flow profiles in this concentration regime (cf. figure 5). For small concentrations of the mixture, there is, in contrast, a drop of the mass flow rate which is mainly due to a decrease of the mass flow rate of the large particles. This drop is closely related to the decrease observed in the flow velocity profile for small mixture concentrations. The large concentration limit is also singular with an enhancement of the mass flow rate in comparison with the value of the plateau.

Figure 6. Mass flow rate ![]() $Q$ (green stars) as a function of the relative volume concentration of small particles
$Q$ (green stars) as a function of the relative volume concentration of small particles ![]() $\varPhi _s$ in the mixture for dense flows at
$\varPhi _s$ in the mixture for dense flows at ![]() $\theta =20^{\circ }$ (a) and supported flows at
$\theta =20^{\circ }$ (a) and supported flows at ![]() $\theta =35^{\circ }$ (b). Here
$\theta =35^{\circ }$ (b). Here ![]() $Q$ is the sum of the mass flow rate of the large (red triangles) and small (blue dots) particles.
$Q$ is the sum of the mass flow rate of the large (red triangles) and small (blue dots) particles.
For the supported regime (![]() $\theta =35^{\circ }$), an optimum of the mass flow rate emerges for a finite value of the mixture concentration:
$\theta =35^{\circ }$), an optimum of the mass flow rate emerges for a finite value of the mixture concentration: ![]() $\varPhi _S\approx 0.4$. Moreover, a wide range of concentration provides a mass flow rate which is higher than the monodisperse case of small particles. The optimum seems to be governed by the large particles. Indeed, the mass flow rate of the large particles also displays an optimum for a finite concentration of the mixture (of the order of
$\varPhi _S\approx 0.4$. Moreover, a wide range of concentration provides a mass flow rate which is higher than the monodisperse case of small particles. The optimum seems to be governed by the large particles. Indeed, the mass flow rate of the large particles also displays an optimum for a finite concentration of the mixture (of the order of ![]() $\varPhi _S\approx 0.3$). In contrast, the mass flow rate of the small particles exhibits a monotone, regular increase with increasing mixture concentration. This result indicates that the establishment of the supported regime for a binary mixture promotes the transport capacity of the flow. The increase in transport efficiency is the result of the segregation process concentrating the large particles within the dense core, which is the most rapid part of the flow.
$\varPhi _S\approx 0.3$). In contrast, the mass flow rate of the small particles exhibits a monotone, regular increase with increasing mixture concentration. This result indicates that the establishment of the supported regime for a binary mixture promotes the transport capacity of the flow. The increase in transport efficiency is the result of the segregation process concentrating the large particles within the dense core, which is the most rapid part of the flow.
5. Interpretation and discussion
In dense gravity-driven flows, the larger particles often tend to rise at the top of the granular packing against gravity, usually associated with the kinetic sieving mechanism introduced by Savage & Lun (Reference Savage and Lun1988). At low inclination, we observe dense flows which exhibit such segregation. In contrast, at higher inclination, the observed segregation pattern is different. The large particles stand neither at the bottom of the flow nor at the top but are localized within the flow at rather constant elevation (i.e. at ![]() $z=10D$). The large-particle layer constitutes the densest part of the flow and is sandwiched between two dilute layers of fines (see figure 3c). The elevation of the large-particle layer corresponds to the location where the granular temperature is minimum (see figure 3d).
$z=10D$). The large-particle layer constitutes the densest part of the flow and is sandwiched between two dilute layers of fines (see figure 3c). The elevation of the large-particle layer corresponds to the location where the granular temperature is minimum (see figure 3d).
The temperature difference between the dilute layer above the dense core and the dense core increases with increasing angle. This seems to indicate that the segregation is driven by temperature gradients. This is apparently different from segregation in gravity-driven flows at moderate inclination angle, but vertical temperature gradients can also play a role in these. Segregation driven by temperature gradients may be understood in the framework of the kinetic theory for binary mixtures of dense granular gases (Arnarson & Jenkins Reference Arnarson and Jenkins2004; Garzó Reference Garzó2008, Reference Garzó2009; Larcher & Jenkins Reference Larcher and Jenkins2013, Reference Larcher and Jenkins2015; Jenkins & Larcher Reference Jenkins and Larcher2020). We anticipate that this kinetic theory, based on instantaneous, pairwise collisions alone, is, for the value of the sliding friction employed in the simulations, relevant until a mixture concentration of 0.60. For higher volume fractions, more enduring interactions at compliant contacts, associated with ephemeral force chains, become increasingly important (Berzi, Jenkins & Richard Reference Berzi, Jenkins and Richard2020). This critical value of the concentration is a known function of the sliding friction, when the tangential coefficient is unity (Berzi & Vescovi Reference Berzi and Vescovi2015); we employ a slightly larger value for the lower value of tangential restitution in the simulations. We next employ this framework and outline the derivation of the equation that predicts a local measure of segregation in a binary mixture of spheres that do not differ much in size or mass. We then obtain two-dimensional solutions of this equation and compare the prediction with the data of the numerical simulations of a supported flow.
The balance of linear momentum for each type of sphere, written in terms of dimensional variables, has the form (e.g. Jenkins & Mancini Reference Jenkins and Mancini1987)
where ![]() $I=L, S$ labels the two sizes of spheres,
$I=L, S$ labels the two sizes of spheres, ![]() ${\boldsymbol {v}}_I$ is the species velocity,
${\boldsymbol {v}}_I$ is the species velocity, ![]() $\boldsymbol{\varPi}_I$ the species pressure tensor, which in a dense flow contains only collisional contributions,
$\boldsymbol{\varPi}_I$ the species pressure tensor, which in a dense flow contains only collisional contributions, ![]() ${\boldsymbol {F}}_I$ is the external force on a sphere
${\boldsymbol {F}}_I$ is the external force on a sphere ![]() $I$, and
$I$, and ![]() ${\boldsymbol {\phi }}_L = -{\boldsymbol {\phi }}_S$ are the forces of interaction between the two sizes of spheres. Segregation of the two sizes is predicted from the weighted difference of the momentum balances, when the inertia of the spheres is neglected and only the contributions of the partial pressures,
${\boldsymbol {\phi }}_L = -{\boldsymbol {\phi }}_S$ are the forces of interaction between the two sizes of spheres. Segregation of the two sizes is predicted from the weighted difference of the momentum balances, when the inertia of the spheres is neglected and only the contributions of the partial pressures, ![]() $p_L$ and
$p_L$ and ![]() $p_S$, to the pressure tensors are retained:
$p_S$, to the pressure tensors are retained:
in which the partial pressures are given in a dense flow by
where ![]() $K_{IJ}=(2/3){\rm \pi} n_I n_J r_{IJ}^3 g_{IJ}$, with
$K_{IJ}=(2/3){\rm \pi} n_I n_J r_{IJ}^3 g_{IJ}$, with ![]() $I = L, S$ and
$I = L, S$ and ![]() $J= L, S$;
$J= L, S$; ![]() $r_{ IJ} = r_ I + r_J$; and the radial distribution functions
$r_{ IJ} = r_ I + r_J$; and the radial distribution functions ![]() $g_{IJ}$ for the two types of spheres will be given later in their approximate forms. This approximate form of the weighted difference of the balances of momentum of the two sizes of spheres provides an equation for predicting local segregation in the dense, inclined flow.
$g_{IJ}$ for the two types of spheres will be given later in their approximate forms. This approximate form of the weighted difference of the balances of momentum of the two sizes of spheres provides an equation for predicting local segregation in the dense, inclined flow.
The Chapman–Enskog procedure for the determination of the velocity distribution function and constitutive relations for a dense binary mixture, as in Jenkins & Mancini (Reference Jenkins and Mancini1989), Arnarson & Jenkins (Reference Arnarson and Jenkins2004), Larcher & Jenkins (Reference Larcher and Jenkins2013, Reference Larcher and Jenkins2015), leads to an expression for the difference in velocities of the two types of spheres:
where ![]() $n_L$ and
$n_L$ and ![]() $n_S$ are the two number densities,
$n_S$ are the two number densities, ![]() $n = n_L + n_S$;
$n = n_L + n_S$; ![]() $D_{LS}$ is the diffusion coefficient,
$D_{LS}$ is the diffusion coefficient,
 \begin{equation} D_{LS}= \frac{3}{2} \frac{1}{n g_{LS}} \left( \frac{2 m_{LS} T}{{\rm \pi} m_L m_S} \right)^{1/2} \frac{1}{8 r_{LS}^2} ; \end{equation}
\begin{equation} D_{LS}= \frac{3}{2} \frac{1}{n g_{LS}} \left( \frac{2 m_{LS} T}{{\rm \pi} m_L m_S} \right)^{1/2} \frac{1}{8 r_{LS}^2} ; \end{equation}
and ![]() ${\boldsymbol {d}}_L$ is the diffusion force of the large spheres,
${\boldsymbol {d}}_L$ is the diffusion force of the large spheres,
in which ![]() $P = p_L + p_S$ is the total pressure and
$P = p_L + p_S$ is the total pressure and ![]() $\mu _L$ is the chemical potential of the large spheres; and
$\mu _L$ is the chemical potential of the large spheres; and ![]() $K_T$ is their thermal diffusion coefficient, all of which will also be given later in approximation. The expression for the velocity difference (5.4) has the same form as the weighted difference of the momentum balances (5.2), when the particle interaction
$K_T$ is their thermal diffusion coefficient, all of which will also be given later in approximation. The expression for the velocity difference (5.4) has the same form as the weighted difference of the momentum balances (5.2), when the particle interaction ![]() ${\boldsymbol {\phi }}_S$ is taken to be
${\boldsymbol {\phi }}_S$ is taken to be
 \begin{align} {\boldsymbol{\phi}}_S&={-}K_{LS} \frac{m_S-m_L}{m_{LS}} \boldsymbol{\nabla} T + \frac{n_L n_S}{n}T \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S) \nonumber\\ &\quad + n_L \frac{\partial}{\partial n_L} \left( \mu_L -\frac{p_L}{T} \right) \boldsymbol{\nabla} n_L + n_L \frac{\partial}{\partial n_S} \left( \mu_L -\frac{p_L}{T} \right) \boldsymbol{\nabla} n_S + n T K_T \boldsymbol{\nabla} T . \end{align}
\begin{align} {\boldsymbol{\phi}}_S&={-}K_{LS} \frac{m_S-m_L}{m_{LS}} \boldsymbol{\nabla} T + \frac{n_L n_S}{n}T \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S) \nonumber\\ &\quad + n_L \frac{\partial}{\partial n_L} \left( \mu_L -\frac{p_L}{T} \right) \boldsymbol{\nabla} n_L + n_L \frac{\partial}{\partial n_S} \left( \mu_L -\frac{p_L}{T} \right) \boldsymbol{\nabla} n_S + n T K_T \boldsymbol{\nabla} T . \end{align} With the connection between (5.4) and (5.2) established, we adopt (5.5) and (5.6) for the velocity differences and follow Arnarson & Jenkins (Reference Arnarson and Jenkins2004) and Larcher & Jenkins (Reference Larcher and Jenkins2013, Reference Larcher and Jenkins2015) in employing approximations to ![]() $g_{LS}$,
$g_{LS}$, ![]() $\mu _L$ and
$\mu _L$ and ![]() $K_T$ that are linear in the small values of
$K_T$ that are linear in the small values of ![]() $\delta r= r_L/r_S-1$ and
$\delta r= r_L/r_S-1$ and ![]() $\delta m=(m_L-m_S)/m$, where, again,
$\delta m=(m_L-m_S)/m$, where, again, ![]() $m = m_S+m_L$. In addition, we here retain only those terms that dominate in the dense limit, and express these in terms of the measure of segregation
$m = m_S+m_L$. In addition, we here retain only those terms that dominate in the dense limit, and express these in terms of the measure of segregation ![]() $X=(n_L-n_S)/(2n)$. The segregation index
$X=(n_L-n_S)/(2n)$. The segregation index ![]() $X$ is the local difference in number fractions of the two sizes of spheres; at points at which it is
$X$ is the local difference in number fractions of the two sizes of spheres; at points at which it is ![]() $0$, there is no segregation; where it is
$0$, there is no segregation; where it is ![]() $1/2$, there are only large spheres; and where it is
$1/2$, there are only large spheres; and where it is ![]() $-1/2$ there are only small spheres. Because
$-1/2$ there are only small spheres. Because ![]() $X$ varies between
$X$ varies between ![]() $-1/2$ and
$-1/2$ and ![]() $1/2$, we also ignore products of it with
$1/2$, we also ignore products of it with ![]() $\delta r$ and
$\delta r$ and ![]() $\delta m$. Then, when expressed in terms of the mixture concentration,
$\delta m$. Then, when expressed in terms of the mixture concentration, ![]() $c$, and
$c$, and ![]() $\lambda =c(3-c)/(2-c)$, the approximation to the radial distribution functions for a binary mixture is given by (e.g. Larcher & Jenkins Reference Larcher and Jenkins2015)
$\lambda =c(3-c)/(2-c)$, the approximation to the radial distribution functions for a binary mixture is given by (e.g. Larcher & Jenkins Reference Larcher and Jenkins2015)
and
in which we regard the mixture as a single phase of identical spheres of radius ![]() $r \equiv (r_ S + r_L ) / 2$ and concentration
$r \equiv (r_ S + r_L ) / 2$ and concentration ![]() $c\approx (4{\rm \pi} /3)r^3n(1+ 3X\delta r)$, and take
$c\approx (4{\rm \pi} /3)r^3n(1+ 3X\delta r)$, and take ![]() $G/c\equiv 5.69(c_M-0.49)/(c_M-c)$, the dense contact radial distribution function of Torquato (Reference Torquato1995), with a singularity at the critical volume fraction
$G/c\equiv 5.69(c_M-0.49)/(c_M-c)$, the dense contact radial distribution function of Torquato (Reference Torquato1995), with a singularity at the critical volume fraction ![]() $c_M$, rather than that of Mansoori et al. (Reference Mansoori, Carnahan, Starling and Leland1971), appropriate for lower volume fractions, with a singularity at unity,
$c_M$, rather than that of Mansoori et al. (Reference Mansoori, Carnahan, Starling and Leland1971), appropriate for lower volume fractions, with a singularity at unity,
where ![]() $H \equiv {\rm d}G/{\rm d}c=G(c_M/c)/(c_M-c)$ and
$H \equiv {\rm d}G/{\rm d}c=G(c_M/c)/(c_M-c)$ and
With these, when the only external force is the vector of the gravitational acceleration, ![]() ${\boldsymbol {g}}$, the contributions from the external forces cancel, and (5.4) and (5.6) yield
${\boldsymbol {g}}$, the contributions from the external forces cancel, and (5.4) and (5.6) yield
 \begin{align} \frac{n_L n_S}{n^2} \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S)&={-}\frac{\rho_L}{\rho} \frac{1}{nT} \boldsymbol{\nabla} P + \frac{n_L}{n} 4G \left[ 1 + \frac{1}{2} \frac{n_S}{n}(3+\lambda)\delta r + \frac{n_S}{n} \delta m\right]\frac{\boldsymbol{\nabla} T}{T} \nonumber\\ &\quad +\frac{3}{29} \frac{n_L n_S}{n^2} G \left({-}2 \delta r + 21 \delta m \right) \frac{\boldsymbol{\nabla} T}{T}\nonumber\\ &\quad +\frac{n_L}{n^2} \left[ \left( \frac{n}{n_L} +4cH +24cH \frac{n_S}{n} \delta r \right) \boldsymbol{\nabla} n_L + 4c H \boldsymbol{\nabla} n_S \right] . \end{align}
\begin{align} \frac{n_L n_S}{n^2} \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S)&={-}\frac{\rho_L}{\rho} \frac{1}{nT} \boldsymbol{\nabla} P + \frac{n_L}{n} 4G \left[ 1 + \frac{1}{2} \frac{n_S}{n}(3+\lambda)\delta r + \frac{n_S}{n} \delta m\right]\frac{\boldsymbol{\nabla} T}{T} \nonumber\\ &\quad +\frac{3}{29} \frac{n_L n_S}{n^2} G \left({-}2 \delta r + 21 \delta m \right) \frac{\boldsymbol{\nabla} T}{T}\nonumber\\ &\quad +\frac{n_L}{n^2} \left[ \left( \frac{n}{n_L} +4cH +24cH \frac{n_S}{n} \delta r \right) \boldsymbol{\nabla} n_L + 4c H \boldsymbol{\nabla} n_S \right] . \end{align}
The spatial gradients of ![]() $n_L=n(0.5+X)$ and
$n_L=n(0.5+X)$ and ![]() $n_S=n(0.5-X)$ may be expressed in terms of those of
$n_S=n(0.5-X)$ may be expressed in terms of those of ![]() $c$ and
$c$ and ![]() $X$ using the approximations
$X$ using the approximations
and
Then
 \begin{align} \frac{n_S}{n} \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S) &={-}\frac{m_L}{\rho} \frac{1}{T} \boldsymbol{\nabla} P + 4G \left[ 1 + \frac{1}{2} \frac{n_S}{n}(3+\lambda)\delta r + \frac{n_S}{n} \delta m\right]\frac{\boldsymbol{\nabla} T}{T} \nonumber\\ &\quad + \frac{3}{29} \frac{n_S}{n} G \left({-}2 \delta + 21 \delta m \right) \frac{\boldsymbol{\nabla} T}{T} + \frac{n}{n_L} \boldsymbol{\nabla} X + 4cH(1+1.5\delta r) \frac{ \boldsymbol{\nabla} c}{c} . \end{align}
\begin{align} \frac{n_S}{n} \frac{1}{D_{LS}} ({\boldsymbol{v}}_L -{\boldsymbol{v}}_S) &={-}\frac{m_L}{\rho} \frac{1}{T} \boldsymbol{\nabla} P + 4G \left[ 1 + \frac{1}{2} \frac{n_S}{n}(3+\lambda)\delta r + \frac{n_S}{n} \delta m\right]\frac{\boldsymbol{\nabla} T}{T} \nonumber\\ &\quad + \frac{3}{29} \frac{n_S}{n} G \left({-}2 \delta + 21 \delta m \right) \frac{\boldsymbol{\nabla} T}{T} + \frac{n}{n_L} \boldsymbol{\nabla} X + 4cH(1+1.5\delta r) \frac{ \boldsymbol{\nabla} c}{c} . \end{align} The mixture pressure ![]() $P$ is given in terms of the mixture concentration, in approximation, as
$P$ is given in terms of the mixture concentration, in approximation, as
Then, when only gravity is present, the sum of the species momentum balances of (5.1) is
or, upon using (5.17) and ![]() $\rho \approx [3/(4{\rm \pi} r^3)](m/2)c$,
$\rho \approx [3/(4{\rm \pi} r^3)](m/2)c$,
With this, the concentration gradient may be eliminated from (5.16):
 \begin{align} \frac{{\boldsymbol{v}}_L -{\boldsymbol{v}}_S}{D_{LS}} &= 4G \left[ \left( \frac{1}{2} (3+\lambda)-\frac{177}{58} \right)\delta r + \frac{179}{116} \delta m \right] \frac{\nabla T}{T} + \frac{m}{2} ( 3 \delta r - 2 \delta m) \frac{{\boldsymbol{g}}}{T} \nonumber\\ &\quad + \frac{n^2}{n_L n_S} \boldsymbol{\nabla} X . \end{align}
\begin{align} \frac{{\boldsymbol{v}}_L -{\boldsymbol{v}}_S}{D_{LS}} &= 4G \left[ \left( \frac{1}{2} (3+\lambda)-\frac{177}{58} \right)\delta r + \frac{179}{116} \delta m \right] \frac{\nabla T}{T} + \frac{m}{2} ( 3 \delta r - 2 \delta m) \frac{{\boldsymbol{g}}}{T} \nonumber\\ &\quad + \frac{n^2}{n_L n_S} \boldsymbol{\nabla} X . \end{align}We apply this equation to a steady, fully developed flow in which the diffusion velocities vanish.
We make the approximations that the mixture concentration in the dense part of the flow is uniform and equal to 0.59. Then, ![]() $\lambda = 1.01$,
$\lambda = 1.01$, ![]() $G =6.49$ and, with
$G =6.49$ and, with ![]() $\boldsymbol {g}=-g \cos \theta \boldsymbol {k}$, where the unit vector
$\boldsymbol {g}=-g \cos \theta \boldsymbol {k}$, where the unit vector ![]() $\boldsymbol {k}$ is normal to the flow and directed upward, (5.20) has the dimensionless form
$\boldsymbol {k}$ is normal to the flow and directed upward, (5.20) has the dimensionless form
 \begin{equation} {\boldsymbol{\nabla}} \left[ \ln \left(\frac{1+2X}{1-2X}\right)^{1/2} + \left( 6.2 \delta m -4.2 \delta r \right) G \ln w \right]={-}\left( \delta m -1.5 \delta r \right) \frac{\cos \theta}{ w^2} \, {\boldsymbol{k}} , \end{equation}
\begin{equation} {\boldsymbol{\nabla}} \left[ \ln \left(\frac{1+2X}{1-2X}\right)^{1/2} + \left( 6.2 \delta m -4.2 \delta r \right) G \ln w \right]={-}\left( \delta m -1.5 \delta r \right) \frac{\cos \theta}{ w^2} \, {\boldsymbol{k}} , \end{equation}
where ![]() $w^2\equiv 2T/(mgD)$. The assumption made regarding the uniformity of the mixture concentration, and its value, are in rough agreement with the measurements in the neighbourhood of the segregation features in the simulations.
$w^2\equiv 2T/(mgD)$. The assumption made regarding the uniformity of the mixture concentration, and its value, are in rough agreement with the measurements in the neighbourhood of the segregation features in the simulations.
If the spheres are made of the same material, ![]() $\delta m = 1.5\delta r$. In this case, the right-hand side of (5.21) vanishes and, with our assumption of the uniformity of the concentration, gravity influences segregation only through the temperature. Then,
$\delta m = 1.5\delta r$. In this case, the right-hand side of (5.21) vanishes and, with our assumption of the uniformity of the concentration, gravity influences segregation only through the temperature. Then, ![]() $6.2\delta m - 4.2\delta r = 5.1\delta r$, and (5.21) may be written as
$6.2\delta m - 4.2\delta r = 5.1\delta r$, and (5.21) may be written as
 \begin{equation} \boldsymbol{\nabla} \left[ {\ln {{\left( {\frac{{1 + 2X}}{{1 - 2X}}} \right)}^{1/2}} + 5.1G\delta r\ln w} \right] = 0. \end{equation}
\begin{equation} \boldsymbol{\nabla} \left[ {\ln {{\left( {\frac{{1 + 2X}}{{1 - 2X}}} \right)}^{1/2}} + 5.1G\delta r\ln w} \right] = 0. \end{equation}The integral of this equation is
where ![]() $w_0$ is the value of
$w_0$ is the value of ![]() $w$ at which
$w$ at which ![]() $X = 0$.
$X = 0$.
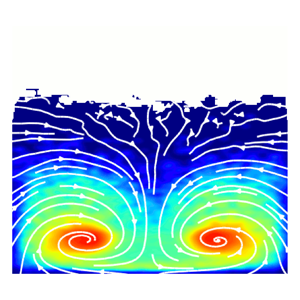
In figure 7, we compare values of the segregation index ![]() $X$, based on the fields of temperature and concentration measured in the simulations, with those predicted by (5.23) for a
$X$, based on the fields of temperature and concentration measured in the simulations, with those predicted by (5.23) for a ![]() $50{:}50$ mixture in mass with
$50{:}50$ mixture in mass with ![]() $r_L/r_S=1.35$ (i.e.
$r_L/r_S=1.35$ (i.e. ![]() $\delta r=0.35$). The location of the segregation is roughly the same, but the centres of the segregation patterns in the simulations are distinct and separated. In our view, the difference in the patterns is due to the presence of convection, which is not incorporated in the theory. The convection rolls result in mixing that brings smaller spheres into the centre of the flow and creates what appears to be a more intense segregation between them. Consequently, the predicted pattern of segregation shows less contrast between the rolls, and their centres are not so distinct and clearly separated.
$\delta r=0.35$). The location of the segregation is roughly the same, but the centres of the segregation patterns in the simulations are distinct and separated. In our view, the difference in the patterns is due to the presence of convection, which is not incorporated in the theory. The convection rolls result in mixing that brings smaller spheres into the centre of the flow and creates what appears to be a more intense segregation between them. Consequently, the predicted pattern of segregation shows less contrast between the rolls, and their centres are not so distinct and clearly separated.

Figure 7. (a) The values of the segregation index ![]() $X$ measured in the numerical simulation for a
$X$ measured in the numerical simulation for a ![]() $50{:}50$ mixture in mass with
$50{:}50$ mixture in mass with ![]() $r_L/r_S=1.35$ (i.e.
$r_L/r_S=1.35$ (i.e. ![]() $\delta r=0.35$); and (b) the values of
$\delta r=0.35$); and (b) the values of ![]() $X$ predicted by the theory.
$X$ predicted by the theory.
6. Conclusion
We have outlined the features of a regime of rapid inclined flow of a binary mixture of spheres as seen in discrete numerical simulations. The regime occurs at relatively high inclinations and involves enhanced transport that is associated with the development of circulation secondary flows and the concentration of the larger spheres within them in response to gradients of granular temperature. This is in contrast to denser flows at lower inclinations that exhibit a simpler segregation pattern with larger spheres at the top, often associated with percolation of the smaller spheres, but also explained as due to gradients of granular temperature. We have also characterized the ratio of mass fraction of small spheres that provides the greatest enhancement of the rapid flows. Finally, we have indicated that the observed segregation patterns in the rapid flows are consistent with the predictions of a kinetic theory for binary mixtures that incorporates the influence of gradients of granular temperature on the segregation.
Additional discrete numerical simulations are necessary to further understand the details of the parameter space, particularly the influence of the particle properties on the flow and segregation in the rapid flows. Also, it would be useful to develop numerical solutions to the continuum equations of the kinetic theory to predict the two-dimensional fields of mixture concentration, velocity and granular temperature in dense, steady, fully developed inclined flows. This would permit a complete comparison between the predictions of the theory and what is seen in the discrete numerical simulations.
Funding
A.N., R.D. and A.V. acknowledge the support of the French Research National Agency through the project ANR-16-CE01-0005.
Declaration of interests
The authors report no conflict of interest.