Hostname: page-component-5c6d5d7d68-wpx84 Total loading time: 0 Render date: 2024-08-15T09:10:54.892Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on the differentiability of discrete Palmer's linearization

Part of: Dynamical systems with hyperbolic behavior Smooth dynamical systems: general theory

Published online by Cambridge University Press:  22 March 2023

Álvaro Castañeda  and
Néstor Jara
Álvaro Castañeda
Affiliation:
Departamento de Matemáticas, Universidad de Chile, Casilla 653, Santiago, Chile (castaneda@uchile.cl, nestor.jara@ug.uchile.cl)
Néstor Jara
Affiliation:
Departamento de Matemáticas, Universidad de Chile, Casilla 653, Santiago, Chile (castaneda@uchile.cl, nestor.jara@ug.uchile.cl)
Get access Rights & Permissions [Opens in a new window]

Abstract

In the context of discrete nonautonomous dynamics, we prove that the homeomorphisms in the linearization theorem are $C^2$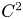 diffeomorphisms. In contrast to other related works, our result does not involve non-resonance conditions or spectral gaps. Our approach is based on the interlacing of the properties of nonautonomous hyperbolicity of the linear part, and boundedness and Lipschitzness of the nonlinearities. Moreover, we propose a functional approach to find conditions for regularity of arbitrary degree.

Keywords

Nonautonomous hyperbolicitynonautonomous difference equationsmooth linearization

MSC classification

Primary: 37C60: Nonautonomous smooth dynamical systems
Secondary: 37D25: Nonuniformly hyperbolic systems (Lyapunov exponents, Pesin theory, etc.)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 2 , April 2024 , pp. 600 - 628
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Backes, L. and Dragičević, D., Smooth linearization of nonautonomous coupled systems. arXiv:2202.12367.Google Scholar
Barreira, L. and Valls, C.. A Grobman–Hartman theorem for nonuniformly hyperbolic dynamics. J. Differ. Equations 228 (2006), 285–310.Google Scholar
Belickiĭ, G. R.. Functional equations and the conjugacy of local diffeomorphisms of a finite smoothness class. Dokl. Akad. Nauk SSSR 202 (1972), 255–258.Google Scholar
Belickiĭ, G. R.. Equivalence and normal forms of germs of smooth mappings. Uspekhi Mat. Nauk 33 (1978), 95–155, 263.Google Scholar
Castañeda, Á., González, P. and Robledo, G.. Topological equivalence of nonautonomous difference equations with a family of dichotomies on the half line. Commun. Pure Appl. Anal. 20 (2021), 511–532.Google Scholar
Castañeda, Á., Monzón, P. and Robledo, G.. Smoothness of topological equivalence on the half-line for nonautonomous systems. Proc. R. Soc. Edinburgh Sect. A 150 (2020), 2484–2502.Google Scholar
Castañeda, Á. and Robledo, G.. Differentiability of Palmer's linearization theorem and converse result for density functions. J. Differ. Equations 259 (2015), 4634–4650.Google Scholar
Coffman, C. V. and Schäffer, J. J.. Dichotomies for linear difference equations. Math. Ann. 172 (1967), 139–166.Google Scholar
Coppel, W. A.. Dichotomies in stability theory. Lecture Notes in Mathematics (Berlin: Springer-Verlag, 1978).Google Scholar
Cuong, L. V., Doan, T. S. and Siegmund, S.. A Sternberg theorem for nonautonomous differential equations. J. Dyn. Differ. Equations 31 (2019), 1279–1299.Google Scholar
Dragičević, D., Zhang, W. and Zhang, W.. Smooth linearization of nonautonomous difference equations with a nonuniform dichotomy. Math. Z. 292 (2019), 1175–1193.Google Scholar
Dragičević, D., Zhang, W. and Zhang, W.. Smooth linearization of nonautonomous differential equations with a nonuniform dichotomy. Proc. Lond. Math. Soc. 121 (2020), 32–50.Google Scholar
Hartman, P.. A lemma in the theory of structural stability of differential equations. Proc. Am. Math. Soc. 11 (1960), 610–620.Google Scholar
Kloeden, P. E. and Rasmussen, M. Nonautonomous dynamical systems. Mathematical Surveys and Monographs, vol. 176 (Providence, RI: AMS, 2011).Google Scholar
Palmer, K. J.. A generalization of Hartman's linearization theorem. J. Math. Anal. Appl. 41 (1973), 753–758.Google Scholar
Plastock, R.. Homeomorphisms between Banach spaces. Trans. Am. Math. Soc. 200 (1974), 169–183.Google Scholar
Pugh, C. C.. On a theorem of P. Hartman. Am. J. Math. 91 (1969), 363–367.CrossRefGoogle Scholar
Sacker, R. J. and Sell, G. R.. A spectral theory for linear differential systems. J. Differ. Equations 27 (1978), 320–358.Google Scholar
Shi, J. L. and Xiong, K. Q.. On Hartman's linearization theorem and Palmer's linearization theorem. J. Math. Anal. Appl. 192 (1995), 813–832.Google Scholar
Sternberg, S.. Local contractions and a theorem of Poincaré. Am. J. Math. 79 (1957), 809–824.Google Scholar
Sternberg, S.. On the structure of local homeomorphisms of Euclidian $n$-space, I. Am. J. Math. 80 (1958), 623–631.Google Scholar