Hostname: page-component-76fb5796d-22dnz Total loading time: 0 Render date: 2024-04-26T04:58:48.224Z Has data issue: false hasContentIssue false
  • English
  • Français

Global existence and large time behaviour for the pressureless Euler–Naver–Stokes system in ℝ3

Part of: Equations of mathematical physics and other areas of application Kinematics

Published online by Cambridge University Press:  22 February 2023

Shanshan Guo ,
Guochun Wu  and
Yinghui Zhang
Shanshan Guo
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 400047, China (guoshanshan0516@163.com)
Guochun Wu
Affiliation:
Fujian Province University Key Laboratory of Computational Science, School of Mathematical Sciences, Huaqiao University, Quanzhou 362021, People's Republic of China (guochunwu@126.com)
Yinghui Zhang*
Affiliation:
School of Mathematics and Statistics, Guangxi Normal University, Guilin, Guangxi 541004, People's Republic of China (yinghuizhang@mailbox.gxnu.edu.cn)
*
*Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the global Cauchy problem for a two–phase flow model consisting of the pressureless Euler equations coupled with the isentropic compressible Navier–Stokes equations through a drag forcing term. This model was first derived by Choi–Kwon [J. Differential Equations, 261(1) (2016), pp. 654–711] by taking the hydrodynamic limit of the Vlasov/compressible Navier–Stokes equations. Under the assumption that the initial perturbation is sufficiently small, Choi–Kwon [J. Differential Equations, 261(1) (2016), pp. 654–711] established the global well–posedness and large time behaviour for the three dimensional periodic domain $\mathbb {T}^3$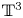. However, up to now, the global well–posedness and large time behaviour for the three dimensional Cauchy problem still remain unsolved. In this paper, we resolve this problem by proving the global existence and optimal decay rates of classic solutions for the three dimensional Cauchy problem when the initial data is near its equilibrium. One of key observations here is that to overcome the difficulties arising from the absence of pressure in the Euler equations, we make full use of the drag forcing term and the dissipative structure of the Navier–Stokes equations to closure the energy estimates of the variables for the pressureless Euler equations.

Keywords

Pressureless Euler equationscompressible Navier–Stokes equationsCauchy problemglobal existencelarge time behaviour

MSC classification

Primary: 35Q30: Navier-Stokes equations
Secondary: 35Q70: PDEs in connection with mechanics of particles and systems 70B05: Kinematics of a particle 35Q83: Vlasov-like equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 1 , February 2024 , pp. 328 - 352
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Baranger, C., Boudin, L., Jabin, P.-E. and Mancini, S.. A modeling of biospray for the upper airways. CEMRACS 2004-mathematics and applications to biology and medicine. ESAIM Proc. 14 (2005), 41–47.CrossRefGoogle Scholar
Boudin, L., Desvillettes, L. and Motte, R.. A modelling of compressible droplets in a fluid. Commun. Math. Sci. 1 (2003), 657–669.CrossRefGoogle Scholar
Boudin, L., Desvillettes, L., Grandmont, C. and Moussa, A.. Global existence of solutions for the coupled Vlasov and Navier–Stokes equations. Differ. Int. Equ. 22 (2009), 1247–1271.Google Scholar
Boudin, L., Grandmont, C. and Moussa, A.. Global existence of solutions to the incompressible Navier–Stokes–Vlasov equations in a time-dependent domain. J. Differ. Equ. 262 (2017), 1317–1340.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P. and Karper, T. K.. On the analysis of a coupled kinetic–fluid model with local alignment forces. Ann. Inst. H. Poincar Anal. Non Linaire 33 (2016), 273–307.CrossRefGoogle Scholar
Carrillo, J. A. and Goudon, T.. Stability and asymptotic analysis of a fluid–particle interaction model. Comm. Partial Differ. Equ. 31 (2006), 1349–1379.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P., Tadmor, E. and Tan, C.. Critical thresholds in 1D Euler equations with nonlocal forces. Math. Models Methods Appl. Sci. 26 (2016), 185–206.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P. and Zatorska, E.. On the pressureless damped Euler–Poisson equations with quadratic confinement: critical thresholds and large–time behavior. Math. Models Methods Appl. Sci. 26 (2016), 2311–2340.CrossRefGoogle Scholar
Chen, G.-Q. and Wang, D.. Convergence of shock capturing schemes for the compressible Euler–Poisson equations. Commun. Math. Phys. 179 (1996), 333–364.CrossRefGoogle Scholar
Chen, Q. and Tan, Z.. Time decay of solutions to the compressible Euler equations with damping. Kinetic Related Models 7 (2014), 605–619.CrossRefGoogle Scholar
Choi, Y.-P.. Compressible Euler equations interacting with incompressible flow. Kinet. Relat. Models 8 (2015), 335–358.CrossRefGoogle Scholar
Choi, Y.-P. and Kwon, B.. The Cauchy problem for the pressureless Euler/isentropic Navier–Stokes equations. J. Differ. Equ. 261 (2016), 654–711.CrossRefGoogle Scholar
Choi, Y.-P.. Global classical solutions and large–time behavior of the two–phase fluid model. SIAM J. Math. Anal. 48 (2016), 3090–3122.CrossRefGoogle Scholar
Choi, Y.-P. and Jung, J.. On the Cauchy problem for the pressureless Euler–Navier–Stokes system in the whole space. J. Math. Fluid Mech. 23 (2021), 16.CrossRefGoogle Scholar
Engelberg, S.. Formation of singularities in the Euler and Euler–Poisson equations. Physica D 98 (1996), 67–74.CrossRefGoogle Scholar
Ertzbischoff, L.. Decay and absorption for the Vlasov–Navier–Stokes system with gravity in a half–space, arXiv:2107.02200 (2021).Google Scholar
Ertzbischoff, L., Han-Kwan, D. and Moussa, A.. Concentration versus absorption for the Vlasov–Navier–Stokes system on bounded domains, arXiv:2101.05157v1 (2021).CrossRefGoogle Scholar
Goudon, T., Jabin, P.-E. and Vasseur, A.. Hydrodynamic limit for the Vlasov–Navier–Stokes equations: I. Light particles regime. Indiana Univ. Math. J. 53 (2004), 1495–1515.CrossRefGoogle Scholar
Goudon, T., Jabin, P.-E. and Vasseur, A.. Hydrodynamic limit for the Vlasov–Navier–Stokes equations: II. Fine particles regime. Indiana Univ. Math. J. 53 (2004), 1517–1536.CrossRefGoogle Scholar
Ha, S.-Y., Kang, M.-J. and Kwon, B.. A hydrodynamic model for the interaction of Cucker–Smale particles and incompressible fluids. Math. Models Methods Appl. Sci. 24 (2014), 2311–2359.CrossRefGoogle Scholar
Han-Kwan, D.. Large time behavior of small data solutions to the Vlasov–Navier–Stokes system on the whole space, arXiv:2006.09848v1 (2020).Google Scholar
Han-Kwan, D., Moussa, A. and Moyano, I.. Large time behavior of the Vlasov–Navier–Stokes system on the torus. Arch. Rational Mech. Anal. 236 (2020), 1273–1323.CrossRefGoogle Scholar
Kato, T.. The Cauchy problem for quasi–linear symmetric hyperbolic systems. Arch. Rational Mech. Anal. 58 (1975), 181–205.CrossRefGoogle Scholar
Liu, H. and Tadmor, E.. Spectral dynamics of the velocity gradient field in restricted fluid flows. Commun. Math. Phys. 228 (2002), 435–466.CrossRefGoogle Scholar
Majda, A.. Compressible fluid flow and systems of conservation laws in several space variables (Berlin/New York: Springer-Verlag, 1984).CrossRefGoogle Scholar
Matsumura, A. and Nishida, T.. The initial value problem for the equation of motion of compressible viscous and heat–conductive fluids. Proc. Japan Acad. Ser. A Math. Sci. 55 (1979), 337–342.CrossRefGoogle Scholar
Mellet, A. and Vasseur, A.. Asymptotic analysis for a Vlasov-Fokker-Planck/compressible Navier-Stokes equations. Comm. Math. Phys. 281 (2008), 573–596.CrossRefGoogle Scholar
Nirenberg, L.. On elliptic partial differential equations. Annali della Scuola Normale Superiore di Pisa–Classe di Scienze 13 (1959), 115–162.Google Scholar
ORourke, P. J.. Collective drop effects on vaporizing liquid sprays, PhD thesis, Los Alamos National Laboratory, 1981.Google Scholar
Vinkovic, I., Aguirre, C. and Simoens, S.. Large eddy simulation of droplet dispersion for inhomogeneous turbulent wall flow. Int. J. Multiph. Flow 32 (2006), 344–364.CrossRefGoogle Scholar
Williams, F. A.. Spray combustion and atomization. Phys. Fluids 1 (1958), 541–555.CrossRefGoogle Scholar
Wu, G. C., Zhang, Y. H. and Zou, L.. Optimal large–time behavior of the two–phase fluid model in the whole space. SIAM J. Math. Anal. 52 (2020), 5748–5774.CrossRefGoogle Scholar
Yu, C.. Global weak solutions to the incompressible Navier–Stokes–Vlasov equations. J. Math.Pures Appl. 100 (2013), 275–293.CrossRefGoogle Scholar