Hostname: page-component-848d4c4894-75dct Total loading time: 0 Render date: 2024-04-30T12:25:05.009Z Has data issue: false hasContentIssue false
  • English
  • Français

Cavitation of a spherical body under mechanical and self-gravitational forces

Part of: Coupling of solid mechanics with other effects Elastic materials Variational principles of physics

Published online by Cambridge University Press:  08 January 2024

Pablo V. Negrón–Marrero Open the ORCID record for Pablo V. Negrón–Marrero [Opens in a new window]  and
Jeyabal Sivaloganathan
Pablo V. Negrón–Marrero
Affiliation:
Department of Mathematics, University of Puerto Rico, Humacao PR 00791-4300, Puerto Rico (pablo.negron1@upr.edu)
Jeyabal Sivaloganathan
Affiliation:
Department of Mathematical Sciences, University of Bath, Bath BA2 7AY, UK (masjs@bath.ac.uk)
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we look for minimizers of the energy functional for isotropic compressible elasticity taking into consideration the effect of a gravitational field induced by the body itself. We consider two types of problems: the displacement problem in which the outer boundary of the body is subjected to a Dirichlet-type boundary condition, and the one with zero traction on the boundary but with an internal pressure function. For a spherically symmetric body occupying the unit ball $\mathcal {B}\in \mathbb {R}^3$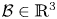, the minimization is done within the class of radially symmetric deformations. We give conditions for the existence of such minimizers, for satisfaction of the Euler–Lagrange equations, and show that for large displacements or large internal pressures, the minimizer must develop a cavity at the centre. We discuss a numerical scheme for approximating the minimizers for the displacement problem, together with some simulations that show the dependence of the cavity radius and minimum energy on the displacement and mass density of the body.

Keywords

non-linear elasticitycavitationself-gravityinternal pressure

MSC classification

Primary: 74B20: Nonlinear elasticity 74F99: None of the above, but in this section 49S05: Variational principles of physics (should also be assigned at least one other classification number in section 49)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , First View , pp. 1 - 31
Check for updates
Copyright
Copyright © The Author(s), 2024. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ball, J. M.. Constitutive inequalities and existence theorems in nonlinear elastostatics. In Nonlinear Analysis and Mechanics Vol. 1 (ed. R.J. Knops). (Heriot-Watt Symposium, Pitman, 1977).Google Scholar
Ball, J. M., Currie, J. C. and Olver, P. J.. Null Lagrangians, weak continuity and variational problems of arbitrary order. J. Funct. Anal. 41 (1981), 135–174.CrossRefGoogle Scholar
Ball, J. M.. Discontinuous equilibrium solutions and cavitation in nonlinear elasticity. Phil. Trans. Royal Soc. London A 306 (1982), 557–611.Google Scholar
Ball, J. M.. Some open problems in elasticity. In Geometry, Mechanics, and Dynamics. (Springer, New York, 2002, pp. 3–59).CrossRefGoogle Scholar
Beig, R. and Schmidt, B. G.. Static, self-gravitating elastic bodies. Proc. R. Soc. Lond. A 459 (2003), 109–115.CrossRefGoogle Scholar
Calogero, S. and Leonori, T.. Ground states of self-gravitating elastic bodies. Calc. Var. Partial. Differ. Equ. 54 (2012).Google Scholar
Gent, A. N. and Tompkins, D. A.. Nucleation and growth of bubbles in elastomers. J. Appl. Phys. 40 (1969), 2520–2525.CrossRefGoogle Scholar
Jia, F., Kodio, O., Chapman, S. and Goriely, A.. On the figure of elastic planets I: gravitational collapse and infinitely many equilibria. Proc. R. Soc. A: Math. Phys. Eng. Sci. 475 (2019).CrossRefGoogle ScholarPubMed
Neuberger, J. W.. Sobolev gradients and differential equations, Lecture Notes in Math., Vol. 1670 (Springer, Berlin, 1997).CrossRefGoogle Scholar
Sivaloganathan, J.. Uniqueness of regular and singular equilibria for spherically symmetric problems of nonlinear elasticity. Arch. Rat. Mech. Anal. 96 (1986), 97–136.CrossRefGoogle Scholar
Sivaloganathan, J.. Cavitation, the incompressible limit and material inhomogeneity. Q. Appl. Math. 49 (1991), 521–541.CrossRefGoogle Scholar
Sivaloganathan, J.. On cavitation and degenerate cavitation under internal hydrostatic pressure. Proc. R. Soc. A 455 (1999), 3645–3664.CrossRefGoogle Scholar