Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Hall, R. R.
1996.
Proof of a conjecture of Heath-Brown concerning quadratic residues.
Proceedings of the Edinburgh Mathematical Society,
Vol. 39,
Issue. 3,
p.
581.
Granville, Andrew
and
Soundararajan, K.
1999.
Topics in Number Theory.
p.
1.
Granville, Andrew
and
Soundararajan, K.
2003.
Decay of Mean Values of Multiplicative Functions.
Canadian Journal of Mathematics,
Vol. 55,
Issue. 6,
p.
1191.
Fainsilber, Laura
Kurlberg, Pär
and
Wennberg, Bernt
2006.
Lattice points on circles and discrete velocity models for the Boltzmann equation.
SIAM Journal on Mathematical Analysis,
Vol. 37,
Issue. 6,
p.
1903.
Coons, Michael
and
Dahmen, Sander R.
2011.
On the residue class distribution of the number of prime divisors of an integer.
Nagoya Mathematical Journal,
Vol. 202,
Issue. ,
p.
15.
Coons, Michael
and
Dahmen, Sander R.
2011.
On the residue class distribution of the number of prime divisors of an integer.
Nagoya Mathematical Journal,
Vol. 202,
Issue. ,
p.
15.
Balog, A.
Rivat, J.
and
Sárközy, A.
2014.
On arithmetic properties of sumsets.
Acta Mathematica Hungarica,
Vol. 144,
Issue. 1,
p.
18.
Frantzikinakis, Nikos
and
Host, Bernard
2016.
Higher order Fourier analysis of multiplicative functions and applications.
Journal of the American Mathematical Society,
Vol. 30,
Issue. 1,
p.
67.
Tenenbaum, Gérald
2017.
Moyennes effectives de fonctions multiplicatives complexes.
The Ramanujan Journal,
Vol. 44,
Issue. 3,
p.
641.
Frantzikinakis, Nikos
and
Host, Bernard
2017.
Multiple ergodic theorems for arithmetic sets.
Transactions of the American Mathematical Society,
Vol. 369,
Issue. 10,
p.
7085.
TERÄVÄINEN, JONI
2018.
ON BINARY CORRELATIONS OF MULTIPLICATIVE FUNCTIONS.
Forum of Mathematics, Sigma,
Vol. 6,
Issue. ,
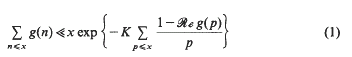
 for all primes p, where
for all primes p, where  is a fixed subset of the closed unit disc
is a fixed subset of the closed unit disc 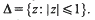 Thus our point of view is similar to that of Halász [Hz 2] in that we seek a general inequality in terms of simple quantities, albeit g(p) may have a quite irregular distribution. We are not concerned here with the problem of asymptotic formulae for the sum on the left of (1) studied by (among others) Delange [D], Halász [Hz 1] and Wirsing [W].
Thus our point of view is similar to that of Halász [Hz 2] in that we seek a general inequality in terms of simple quantities, albeit g(p) may have a quite irregular distribution. We are not concerned here with the problem of asymptotic formulae for the sum on the left of (1) studied by (among others) Delange [D], Halász [Hz 1] and Wirsing [W].



