Hostname: page-component-76fb5796d-22dnz Total loading time: 0 Render date: 2024-04-26T16:11:25.725Z Has data issue: false hasContentIssue false
  • English
  • Français

On an equation occurring in Falkner and Skan's approximate treatment of the equations of the boundary layer

Published online by Cambridge University Press:  24 October 2008

D. R. Hartree
D. R. Hartree
Affiliation:
St John's College
Get access Rights & Permissions [Opens in a new window]

Extract

The differential analyser has been used to evaluate solutions of the equation

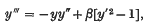
with boundary conditions y = y′ = 0 at x = 0, y′ → 1 as x → ∞, which occurs in Falkner and Skan's approximate treatment of the laminar boundary layer. A numerical iterative method has been used to improve the accuracy of the solutions, and the results show that the accuracy of the machine solutions is about 1 in 1000, or rather better.

It is shown that the conditions are insufficient to specify a unique solution for negative values of β a discussion of this situation is given, and it is shown that for the application to be made of the solution the appropriate condition is that y′ → 1 from below, and as rapidly as possible, as x → ∞. The condition that y′ → 1 from below can be satisfied only for values of β0, greater than a limiting value β0, whose value is approximately − 0·199, and which is related to the point at which the laminar boundary layer breaks away from the boundary.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 33 , Issue 2 , April 1937 , pp. 223 - 239
Copyright
Copyright © Cambridge Philosophical Society 1937

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Falkner, V. M. and MissSkan, S. W., Phil. Mag. 12 (1931), 865;CrossRefGoogle ScholarAero. Res. Comm. R. and M. 1314.Google Scholar

† Bush, V., J. Franklin Inst. 212 (1931), 447.CrossRefGoogle Scholar

‡ Hartree, D. R., Nature, 135 (1935), 940.CrossRefGoogle Scholar

§ Falkner and Skan, loc. cit. equation (11).

* Falkner and Skan, loc. cit. equation (26).

* It is assumed here that Δy does not increase so rapidly that y″ Δy does not tend to zero; this is confirmed by the solution (11), since there is an exponential factor in the asymptotic approach of y″ to 0, and Δy only increases as a power of x.

† Whittaker, and Watson, , Modern Analysis (4th ed.) (Cambridge, 1927),Google Scholar § 16·5.

* This argument assumes that if Δ1y′ is the variation of y′ for a change Δβ of β, then Δ1y′/Δβ does not tend to zero as rapidly as y″, which is found to be the case.

* V. Bush, loc. cit.

† Loc. cit. Table I. Their results extend to β = 4/3 only. y″(0) of the present paper is −2a 2/[F 2(s)]½ in Falkner and Skan's notation.

* The counters are of the “direct drive” type, reading to tenths of a revolution, and the number of revolutions of the y′ counter was 1024y′, so that the last figure of the counter reading corresponds to a fourth decimal in y′. This fourth decimal was kept in the readings and interpolations, though it was expected that it would be purely nominal.

* Howarth, L., Aero. Res. Comm. R. and M. 1632 (1934), p. 10,Google Scholar equation (2·43), pp. 11, 12, and Table I.

† Falkner, V. M., Phil. Mag. 21 (1936), 624.CrossRefGoogle Scholar