An ingenious construction due to Connelly and Henderson (2) has shown that there exists a rectilinearly triangulated convex polyhedron P in 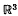having the property that at least one vertex of the triangulation lies in the interior of a face of P, and yet there is no isomorphic triangulation of a convex polyhedron P′ all of whose vertices are vertices of P′. Thus the assertion beginning on the top line of p. 354 of (1) is false, which leaves a gap in the proof of essentially the main result of (1), namely that any rectilinearly triangulated convex polyhedron in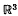can be simplicially collapsed onto its boundary minus a 2-simplex σ. The purpose of this note is to show that the theorem is nevertheless still true. In any case the Corollaries 2 and 3 in (1) are unaffected by the error.